Henderson-Hasselbalch Equation – Estimating the pH Of Buffers

What is Henderson Hasselbalch Equation?

Henderson Hassekbalch equation is easy to determine the ionisation constants of strong acids and bases using direct methods. However, weak acids and bases have a very low extent of ionisation, and the determination gets tougher. Thus, to approximate the pH of weak acids and bases, we use the Henderson-Hasselbalch Equation.

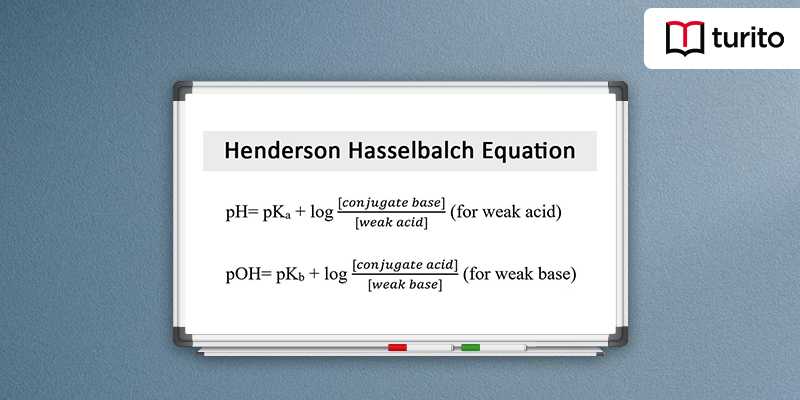
pH = pKa + log10 ([A^–]/[HA]),

where [A^–] is the molar concentration of the acid’s conjugate base.

and [HA] represents the molar concentration of the weak acid.

The pH of an aqueous solution is the measure of its level of acidity or alkalinity. The acid dissociation constant (pKa) is a quantitative measure of the acid strength in the solution. When you combine the two concepts, the Henderson Hasselbalch equation gives a relationship between the pH of aqueous acids and their pKa. So, when given the concentration of the acid and its conjugate base, or the base and the corresponding conjugate acid, you can estimate the pH of a buffer solution using the Henderson equation. 

Let’s recall: What is a buffer solution? Any solution that resists a change in pH when an acid or base is added is called a buffer solution.  Its pH remains constant as the solution is diluted. However, usually small amounts of acid and base are added to it.  A buffer solution typically comprises a weak acid and its salts or a weak base and its salts.

History of Henderson Hasselbalch Equation 

The equation was first derived by Lawrence Joseph Henderson– an American chemist. Later, Karl Albert Hasselbalch– a Danish chemist, re-expressed the equation in logarithmic terms. Thus, the equation derives its name from the two scientists participating in its formulation. 

Derivation of the Henderson Hasselbalch Equation

Taking an example of ionisation of weak acid HA, we can derive the equation as follows:

HA + H₂O ⇋ H⁺ + A⁻

Its acid dissociation constant (Ka) can be written as follows:

Ka = [H⁺] [A⁻][HA]

Now taking the negative log of both sides:

−log Ka= −log [H⁺] [A⁻][HA]

⇒−log Ka= −log [H⁺] –log [A⁻][HA]

We know that −log [H⁺]= pH 

and

−log Ka = pKa

So, the above equation can also be written as,

pKa = pH – log [A⁻][HA]

On rearranging the equation,

⇒ pH = pKa + log[A⁻][HA]

This equation is named the Henderson Hasselbalch equation or Henderson equation. 

Assumptions made in Deriving the Henderson Equation Following assumptions have been made in deriving the Henderson Hasselbalch equation: Assumption 1: The acid, HA, is monobasic.  Assumption 2: The self-dissociation of water can be ignored. Assumption 3: The salt is completely dissociated in the solution.

Derivation of Henderson Hasselbalch Equation for Base

The equation helps estimate the pH of a buffer solution. We can also find the equilibrium pH in acid-base reactions. 

We use the equation to infer when

pH = pKa

log [A⁻][HA] = 0

[A⁻] = [HA]

So when the molar concentration of the acid’s conjugate base [A⁻] is equal to the molar concentration of the weak acid, then pH = pKa, i.e., the acid will be half dissociated.

In the same manner, we can determine the dissociation of a weak base B:

B + H₂O ⇋ OH⁻ + HB⁺

Its base dissociation constant (Kb) can be written as:

Kb = [BH+] [OH⁻][B]

Taking the negative log on both sides

−logKb = −log [BH⁺] [OH⁻][B]

⇒−logKb = −log [OH⁻] −log [BH⁺][B]

We know that −log [OH⁻] = pOH and −logKb = pKb

So, we can write the equation as follows:

pKb= pOH − log [BH⁺][B]

On rearranging the equation, we get the Henderson Hasselbalch equation,

⇒pOH = pKb + log [BH⁺][B]

Here

[BH⁺] is the molar concentration of the conjugate base and [ B ] is the molar concentration of a weak base.

Limitations of the Henderson Hasselbalch Equation

The Henderson Hasselbalch equation isn’t able to predict the right values for the strong bases and strong acids. This limitation occurs because it takes several assumptions in its formulation. It assumes the concentration of the acid and its conjugate base at chemical equilibrium remains the same as the formal concentration.

The equation doesn’t consider the self-dissociation by water. Thus, it doesn’t offer accurate pH values for very dilute buffer solutions.

Solved Examples for Calculating the pH of a Buffer Solution

Example 1: A buffer solution containing 0.4M CH₃COOH and 0.6M CH₃COO^–. The Ka of CH₃COOH is 1.8  10^-5. Calculate the pH of the buffer solution.

According to the Henderson Hasselbalch equation, pH = pKa + log ([CH₃COO–]/[CH₃COOH])

Ka = 1.8  10^-5

⇒ pKa= -log (1.8  10^-5)

= 4.7

On substituting the values in the equation, we have:

pH = 4.7 + log (0.6M /0.4M)

= 4.7 + log (1.5)

= 4.7 + 0.17

= 4.87

Thus, the pH of the solution is 4.87.

Example 2: An acetic acid solution has a pH of 6.75 and pKa = 4.75. Find the ratio of acid to its conjugate base.

The Henderson Hasselbalch equation is as follows:

pH = pKa + log[A⁻][HA]

Substituting the given values in the equation:

6.75 = 4.75 + log[A⁻][HA]

2 = log[A⁻][HA]

On removing log

102 = [A⁻][HA] = 100

So, the ratio of acid to the conjugate base will be the reciprocal of the above, i.e., [HA]/[A⁻] = 100.

Example 3: If you have to produce a buffer solution with a pH of 5.75 and you have 30.0g of acetic acid. What amount of sodium acetate (in moles) would you add to achieve the desired pH? Given the pKa of acetic acid = 4.75.

Using the Henderson Hasselbalch equation:

pH = pKa + log[A⁻][HA]

And assuming that these ions will occupy the same volume,

log = [A⁻][HA] = log [mol of A⁻][mol of HA]

Using the formula of acetic acid, we know that 30g of acetic acid is equal to 0.5mol since the molar mass of acetic acid is 60 g/mol.

Thus, using the above values, we get the following equation:

5.75 = 4.75 + log[A⁻][0.5]

Solving the above equation for A⁻, we get 5 mol.

Therefore, 5 mol of sodium acetate will be needed to reach the desired pH in a buffer solution.

Example 4: A hydrofluoric acid solution has a 0.3M concentration. The Ka for HF is 7.2∗10⁻⁴.  If we slowly add sodium hydroxide to this solution, what will the pH of the solution be at the half equivalence point?

Using the Henderson-Hasselbalch equation, you need not worry about using both the acid and the base molar amounts. The concentration of the conjugate base at the half equivalence point will be equal to that of the weak acid, and you can easily simplify the equation.

The Henderson-Hasselbalch equation is as follows:

pH= pKa + log [A⁻]/[HA]

On simplifying the above equation for half equivalence point:

pH = pKa

So, the pH is equal to the acid dissociation constant.

Since the acid dissociation constant for hydrofluoric acid is given, the pH at the half equivalence point can be calculated as follows:

pH = −log [7.2∗10⁻⁴]

pH = 3.14

Therefore, the pH of the solution at the half equivalence point is 3.14.

Points to remember when solving the Henderson Hasselbalch equation: Make sure you choose the pKa if it is not already given.  Pay special attention to positive and negative numbers.  pH is always equal to the negative log of the concentration of hydrogen ions in the solution. When only half of the acid dissociates, the value of [A]/[HA] is 1.

Henderson Hasselbalch Equation Calculator

You can also use the online Henderson Hasselbalch calculator to calculate the pH. The steps involved in the process are as follows:

Step 1: Enter the conjugate acid/base molar concentration and dissociation constant in the input field.

Step 2: Then click the “Calculate pH” button to get the result.

Step 3: Finally, the hydrogen ion concentration in the buffer solution will be given in the output field.

Conclusion

Henderson Hasselbalch equation is a useful formula for calculating the pH of a buffer solution. It works for both acids and bases. The equation helps calculate the amount of acid and conjugate base that must be combined to prepare a buffer solution with a particular pH. 

The equation is valid when it has acid and conjugate base equilibrium concentrations. In the case of solutions containing strong acids or bases, the equilibrium concentrations can be far from those suggested by neutralisation stoichiometry. 

Frequently Asked Questions

Q1. What is a strong base?

A strong base is fully ionic. For example, potassium hydroxide and sodium hydroxide are strong bases. It is conceived that a strong base gets 100% divided into hydroxide ions and metal ions such that one mole of sodium hydroxide dissociates to give a solution containing a mole of hydroxide ions.

Q2. What is the difference between KA and pKa?

Ka is the acid dissociation constant, and pKa is its -log. Similarly, Kb is the base dissociation constant, and pKb is its -log. These acid and base dissociation constants are expressed in terms of moles per litre (mol / L).

Q3. Which acids and bases’ pH can be determined using the Henderson Hasselbalch equation?

The equation is only an approximation of the pH of acids and bases. It should not be used for strong or concentrated solutions. It is also not suitable for extremely low pH acids or high pH bases.