Newton’s Second Law of Motion : Definition, Formulas

Newton’s first law of motion introduced you to the concept of inertia and why a body at rest or in motion continues to be in that state. It implies that objects at equilibrium have all forces balanced. The first law leaves you wondering what would happen when an external force is applied to the body? How would a change in balanced forces affect the state of equilibrium? The solution to your query lies in Newton’s second law of motion. 

Table of Content 

• What is Newton’s Second Law of Motion?
• Formula Derivation
• Force of Changing Mass
• Solved Examples
• Examples from Real Life 

What is Newton’s Second Law of Motion?

The first law of motion suggested that when the forces acting on a body are balanced, the acceleration is 0 m/s, i.e., the objects at equilibrium do not accelerate. So, according to Newton, an unbalanced force accelerates an object. 

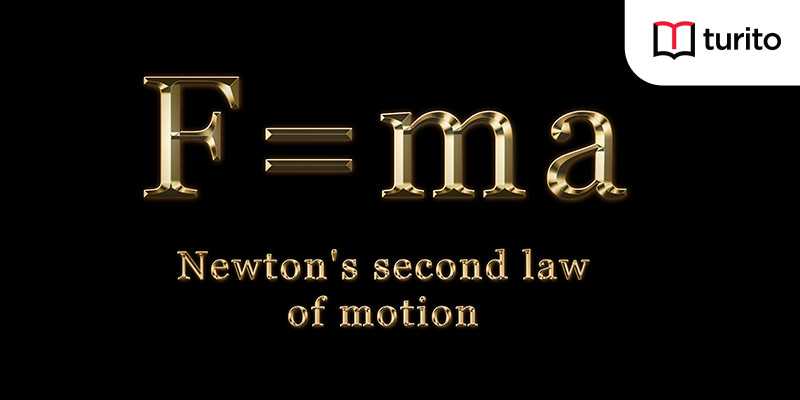
Newton’s second law of motion definition: Force is equal to the momentum change rate. When the mass is constant, the force on the body equals mass times acceleration.

Newton’s second law of motion can be written in the following mathematical form.

F = ma

Understanding Newton’s Second Law 

Newton’s second law suggests that the acceleration of a body is dependent on two variables-

• The net force acting on the object
• Mass of the object

Since F= ma, acceleration in a body will be directly proportional to the net force acting on it but inversely proportional to the body’s mass of the body. 

So, on increasing the force applied to an object, the acceleration will increase too. However, when the object’s mass increases, the acceleration will decrease. 

Some common applications of Newton’s second law of motion are mentioned below:

• Pushing or pulling an empty cart can be compared to a loaded cart that has more mass.
• On applying the same force to move a car and a bike, the car’s acceleration will be lesser than the bike’s because the car has more mass. 
• On kicking a ball, you apply a force on it in a specific direction. It moves in the direction as per the amount of force applied. If you apply more force, the distance covered by the ball will be more.  

Think about it:What would happen to acceleration if you double the force applied and double the mass of a body?

Newton’s Second Law of Motion Formula Derivation

If you assume a moving point object to have mass = m, 

Let its initial velocity be u, and the final velocity be v.

The momentum of the body will be p = mass×velocity

So, the initial momentum will be written as follows:

P_i=mu

The final momentum will be written as:

P_f=mv

The change in momentum can be represented by the following mathematical expression:

 ⇒△p =P_f − P_i

 ⇒△p = mv− mu

Now, if you take the time interval for this change of momentum to be t. Then, the rate of change of momentum is written as follows:

 ⇒Rate of change of momentum =△p/t

⇒△p/t =mv – mu / t

The expression can also be written as follows:

⇒△p/t =m (v-u)/t

Since it is known that (v-u)/t is the expression for the acceleration of the body, on substituting the value, we get

⇒△p/t =ma

Newton’s second law of motion states that F ∝ △p/t

So, F∝ma

Removing the proportionality sign,

⇒F=kma

Here k is the proportionality constant. 

Calculating for unit force,

1 unit of force = k×(1kg)×(1m/_s²)

Therefore k=1/1×1=1

So, when the value of k is taken 1, the equation becomes,

 ⇒F = ma

Force for Changing Mass

Suppose a body at a point (0) defined by location  ^X_o and time t_o has a mass m0 and travels with a velocity v_o. 

When a force F is applied, it moves to point 1, defined by location X₁ and time t₁. Also, the velocity and mass of the body undergo change during travel. The mass changes to values m₁, and the velocity becomes v₁. 

As per Newton’s second law, you can determine the new values of m1 and v1 when given the value of force acting on the body using the following formula:

F= m1v1 -m0v0 / t1-t0

Solved Examples 

Following are some solved examples based on Newton’s second law of motion. 

Example 1: How much force is needed to accelerate a 100 kg car at 5 m/s2?Answer: Newton’s second law of motion establishes a relationship between the object’s mass, the net force, and its acceleration: Therefore, using the following formula, you can calculate the force:  F = ma On substituting the values 100 kg × 5 m/s2= 500 N Therefore, the force needed to accelerate a 100 kg car at 5 m/s2 is 500N

Example 2: A train starts its journey from the railway station and gains a speed of 40 m/s within 80 seconds. If the total mass of the train is 10000 kg along with the passengers, what is the total force applied by the train engine to pull the train?Answer: Given: initial velocity = u = 0 m/s2 Final velocity = v= 40 m/s Time= t= 80 seconds Mass= m= 10,000 kg F = ma a = (v-u)/t a = (40-0)/80 a = 1/2 m/s2 a = 0.5 m/s2 F= ma Substituting the value of a in the above equation F= 10,000 (0.5) F= 5000 N Thus, the force applied by the train engine is 5000 N.

Check Your Knowledge: Q1. What acceleration would result when a 12N net force is applied to a 5 kg object?  Q2. If a net force of 25 N is applied to a body and it causes it to accelerate at a rate of 10m/s2, what would be the mass of the body?  Q3. A sled is accelerating at a rate of 7 m/s2. If the mass remains constant but the net force is tripled, what will be the new acceleration of the sled?

Newton’s Second Law of Motion Examples from Real Life

1. Rocket Launch

Watching a rocket take off often leaves you with the thought that how many people or how much mass can it carry off the earth into space. Newton’s second law of motion explains the concept of rockets in terms of their mass, acceleration, and force. 

The force applied to the rocket to enable its upward movement is termed thrust. The greater the thrust, the greater will be the acceleration. Also, the acceleration depends on the rocket’s mass. So, a lighter rocket, presumably with fewer people, low mass engines, payload, propellants, and equipment, will have faster acceleration.

But why does a rocket gain speed as it rises? Does Newton’s law explain that too?

Yes. The engines burn the propellant and fuel the rocket. As the rocket advances upwards, the propellant is consumed. Consequently, the rocket’s mass reduces, and the rocket ascends. In view of Newton’s second law of motion, the acceleration of the rocket increases as its mass decreases, which is why it lifts off slowly at first but later speeds up.

2. Car Crash

Since the second law of motion states that force is the product of the body’s mass and acceleration, the force with which two cars collide is dependent either on the mass or the car’s acceleration. Suppose the acceleration or mass of the car increases, and the collision force will also increase. 

3. An object dropped from a height.

When you drop an object from a certain height, it accelerates downwards. This acceleration is further augmented due to the gravitational pull of the earth. The acceleration increases as the object reach nearer to the earth’s surface. According to the second law of motion, acceleration is directly proportional to the force. So, when the object hits the ground, the impact force acts on it. This force explains why a thrown from a taller building suffers more than the same object thrown from a comparatively shorter building.

Frequently Asked Questions

1. Why does a car have a greater acceleration than a truck when given a push with an equal amount of force?

A. Newton’s second law of motion explains acceleration produced in cars and trucks by applying an equal magnitude of force to both. On pushing a car and a truck with the same force, the car accelerates more because its mass is far lesser than the mass of the truck.

2. Why are racing cars designed to be lightweight?

A. According to the second law of motion, cars with reduced weight have a higher speed as a lower mass means more acceleration. A higher acceleration ensures greater chances of winning the race.

3. How does a karate player break bricks?

A. A karate player employs the concept of the second law to break bricks. As the law says that the force applied on a body is proportional to the acceleration, the karate players move their hands swiftly over the brick, gaining acceleration and producing a proportionate amount of force sufficient to break the bricks.

Conclusion

Newton’s second law of motion clears all ambiguity that surfaces on going through the first law. This law can be applied to study various real-life situations such as car collisions, falling objects, the speed of moving objects, and more. It also allows you to design objects as per the requirements, i.e., when an object requires higher acceleration, it is best to reduce its mass.