What is One Variable Equations?
Be it Math in school or any other competitive exam out there, single-variable equations are a crucial part of them. When dealing with these, you need to know how to set them, use them, and manipulate them the right way. After all, these kinds of equations form the basis of even more complicated equations like those with multiple variables and quadratics.
In today’s post, we will help you deal with the same – one-variable equations. With all the data available in the post, you will be able to tackle these equations perfectly no matter how they are presented to you.
These will also help you deal with more complicated elements in the subject. So, let us find out what linear equations are, how to set them up, and solve them.
What Are Linear Equations in One Variable?
Before we talk about how to solve linear equations in one variable, let us first find out what linear equations are. The kind of equation in which the degree of every variable used is exactly equal to the value one is a linear equation. As for linear equations in one variable, these are the ones that have only one variable in them.
Also, when it comes to the solution to these equations, there is only a single solution to them. When we talk about them based on graphical representation, if you draw them on a graph, you will get a straight line only.
This line further can appear either horizontally or vertically. Now, let us tell you how you can represent this kind of equation.
Representing Linear Equations in One Variable
To know the methods for solving one-variable equations, you must first know how to represent them. Here is how you can denote linear equations in one variable:
a x + b = 0
In this equation, a and b can be any two integers. As for the value of x, it can be any unknown variable and has only one solution. Let us now take an example to help you understand it better.
Here is an example:
If you add four to a certain number and it gives 10, what is that number?
Now, to find out that number in the above example, you need to represent the problem simply. For it, you can determine the number as x. With this, you can write down the problem as:
x + 4 = 10
This expression here is an equation that helps us write the large problem shortly and simply. Also, you can see that the equation x + 4 = 10 has one variable which is x. Also, this variable has the highest power equal to one.
Thus, this equation is also linear in one variable. To get the solution here, you can easily use the other value to find x. Here, it is 6.
In simple words, you can say that when it comes to a linear equation in one variable, there is an equation that has an unknown quantity (say, x) that you have to find. This quantity here can only appear as a multiple of x. It can never, in any case, appear as a function of x (for example x2, x3, x6, sin x, √x, etc.)
Solving One-Variable Equations
Now that you know what linear equations in one variable are, let us move on to the next step in the post–solving linear equations in one variable. After reading this section of the post, you will be able to:
- Recognize simple one-variable equations (linear equations),
- Solve these simple one-variable equations easily, and
- Check if your solution is correct with the substitution method.
Solving One-Variable Equations by Collecting Terms
Let us start by telling you about solving linear equations variables on one side. To solve a linear equation, you need to first bring all the integers on one side and variables on the other. Let us show you how. Suppose, you have to solve this equation:
3x + 15 = x + 25
Here, in this equation, you must know that the equal sign (=) used represents the balance between the two sides – left and right of the equation. It means that the values on the left side are equal to those on the right.
Thus, here you should note that if you do something to the equation on one side, you have to do the same on the other, as well.
For instance, if you add 2 to the right side, make sure to do the same for the left-hand side of the equation, as well. If you fail to do so, you will disturb the balance of the equation.
Now that you know this, let us move on to how to solve the above equation. The first thing you need to do is gather all the variables (here, x) on one side and the numbers on another.
Keeping this in mind, you get:
3x – x = 25 – 15
By solving this, you will get:
2x = 10
Now, to determine the value of x here, simply move 2 to another side. You get:
x = 10 / 2
x = 5
So, the value of x in the equation is 5. You can also check if this answer is right by substituting the answer in the original equation. If by solving it, you get the same value on both sides, your answer is correct. For instance, when you put x = 5 in the equation 3x + 15 = x + 25, you get:
3(5) + 15 = 5 + 25
By solving this, you get:
3(5) + 15 = 30
15 + 15 = 30
30 = 30
Since both L.H.S and R.H.S of the equation are equal (30), your answer is correct. Let us take another example. This time, the equation you need to solve is:
2x + 3 = 6 − (2x − 3)
Here is how you can solve it to get the value of x:
First, you need to remove the brackets that are on the right-hand side of the equation. With this, you get:
2x + 3 = 6 − 2x + 3
Now, let us solve the right side. Here is what you will get:
2x + 3 = 6 + 3 − 2x
2x + 3 = 9 − 2x
Now, you can see that both the x’s are on the opposite side. So, like the first equation, you need to get them on the same side. Also, you have to get all the integers (here, 9, and 3) on another side, as well. Thus, you get:
2x + 2x = 9 – 3
By solving it, you will get:
4x = 6
Now, in order to determine the value of x here, simply move 4 to another side. You get:
x = 6 / 4 = 3 / 2
x = 1 ½
Now that we have our value of x, let us check if it is right by substituting it in the original equation, and finding if we get L.H.S = R.H.S.
The original equation is: 2x + 3 = 6 − (2x − 3), and value of x we just found out is: x = 1 ½
With this data, we get:
2 (1 ½) + 3 = 6 – (2 (1 ½) – 3)
Solving it, we get:
3 + 3 = 6 – 0
6 = 6
Here again, since both L.H.S and R.H.S of the equation are equal (6), you have the right answer. Now, following this, here are some equations for you to solve yourself:
- x + 8 = 12
- 25 − x = 14
- 6x = 2
- 5x + x = 2
- 5 − 3x = −4
Solving One Variable Equation by Removing Brackets and Collecting Terms
After solving linear equations by collecting terms, let us move on to solving one-variable equations by removing brackets and collecting terms. For this, let us solve the following equation:
8 (x − 3) − (6 − 2x) = 2 (x + 2) – 5 (5 − x)
To solve this equation, we will first begin with the brackets. Let us multiply out the brackets keeping any negative signs in mind. With this, we get:
8x − 24 − 6 + 2x = 2x + 4 − 25 + 5x
Now, you have to collect the x terms on one side and the numbers on the other. This will tidy up the equation and help us solve it better. This is what you will get:
8x + 2x – 2x – 5x = 4 – 25 + 24 + 6
Solving this we get:
3x = 9
x = 9 / 3
x = 3
Like earlier, we will again substitute the value of x (x = 3) in the original equation8 (x − 3) − (6 − 2x) = 2 (x + 2) – 5 (5 − x).
With this, we get:
8(3 − 3) − (6 – 2 (3)) = 2 (3 + 2) – 5 (5 − 3)
0 – 0 = 10 – 10
0 = 0
Since both L.H.S and R.H.S of the equation are equal (0), you have the right answer.
Let us take another example. For this, the equation is:
(x + 1) (2x + 1) = (x + 3) (2x + 3) – 14
To solve this, you have to remove all the brackets first. With it, we get:
2×2 + x + 2x + 1 = 2×2 + 3x + 6x + 9 – 14
By solving the equation further, we get:
2×2 + 3x + 1 = 2×2 + 9x – 5
Now, you know that when dealing with a one-variable equation, there should be x to the power of “something” terms in it.
Therefore, we will subtract 2×2 from both sides, as both of them have this term in them.
This will leave us with:
3x + 1 = 9x – 5
Now, like earlier, we will shift all the x’s on one side and numbers on another to solve this equation completely. Doing so, we get:
1 + 5 = 9x – 3x
Solving it, we get:
6 = 6x
1 = x
Thus, the value of x that we get here is 1. Now, we will substitute x = 1 in the original equation (x + 1) (2x + 1) = (x + 3) (2x + 3) – 14. Here is what we will get:
(1 + 1) (2 (1) + 1) = (1 + 3) (2 (1) + 3) – 14
By solving this, we will get:
(1 + 1) (2 + 1) = (1 + 3) (2 + 3) – 14
(2) (3) = (4) (5) − 14
6 = 20 – 14
6 = 6
Here again, since both L.H.S and R.H.S of the equation are equal (6), you have the right answer. Now, following this, here are some equations for you to solve yourself:
- 5(1 − 2x) + 2(3 − x) = 3(x + 4) + 14
- 6 − 4(x + 3) = 2(x − 1)
- 5(3 − x) − 2(4 − 3x) = 11 − 2(x − 1)
Solving Linear Equations with Fractional Coefficients
After solving one-variable equations by removing brackets and collecting terms, let us see how to solve linear equations with fractional coefficients. For it, the example we will take is to solve the following equation:
4(x + 2) / 5 = 7 + 5x / 13
To solve this equation, you first have to deal with the cause of difficulty here. In this case, it is the fractions in the equation. So, we have to remove them to work with whole numbers.
For it, let us multiply both sides by 5 and then multiply them by 13.
We are doing this on both sides since both sides of the equation are equal. So, we get:
(4(x + 2) / 5) * 5 * 13 = (7 + 5x / 13) * 5 * 13
Doing so will remove the fractions, and we will get:
(4(x + 2) / 5) 65 = (7 + 5x / 13) 65
On solving this further, we will get:
52(x + 2) = 455 + 25x
Now, the equation that we have here is much more familiar than the one we had earlier in the question. After this, we are going to follow the same steps as we did earlier in other problems.
It means that we will multiply out the brackets first, and then collect the x’s on one side and numbers on another. Following this, we get:
52x + 104 = 455 + 25x
52x – 25x = 455 – 104
On solving this, we will get:
27x = 351
x = 351 / 27
x = 13
Now, again we will check this answer by substituting x = 13 in the original equation that is:
4(x + 2) / 5 = 7 + 5x / 13
By substituting x = 13 in it, we get:
4(13 + 2) / 5 = 7 + 5 (13) / 13
On solving this, we get:
60 / 5 = 7 + 5
12 = 12
Since both L.H.S and R.H.S of the equation are equal (12), you have the right answer.
Let us take another example. For this, the equation is:
x + 5 / 6 − x + 1 / 9 = x + 3 / 4
In order to solve this, we will first put brackets around the division line as it also acts as a bracket, and eventually, we have to remove this.
So, we will get:
(x + 5) / 6 – (x + 1) / 9 = (x + 3) / 4
After this, you can see that now, there is a need for a common denominator to solve the equation further. For it, we need a number that will divide all the individual denominators (6, 9, and 4) exactly. This is 36. So, let us multiply the equation throughout by 36.
Here is what we will get by doing so:
36 (x + 5) / 6 – 36 (x + 1) / 9 = 36 (x + 3) / 4
On solving this, we will get:
6 (x + 5) / 1 – 4 (x + 1) /1 = 9 (x + 3) / 1
This can also be written as:
6 (x + 5) – 4 (x + 1) = 9 (x + 3)
Now, we will remove the brackets to make the equation simpler, and solve it readily. Here is what we will get:
6x + 30 − 4x − 4 = 9x + 27
After this, like earlier, collect all the x terms on one side and numbers on another. You will get:
6x − 4x – 9x = 27 − 30 + 4
On solving this, we get:
6x – 13x = 31 – 30
– 7x = 1
x = – 1 / 7
After this, again we will check this answer by substituting x = – 1 / 7 in the original equation that is:
x + 5 / 6 − x + 1 / 9 = x + 3 / 4
By substituting x = – 1 / 7 in it, we get:
(– 1 / 7) + 5 / 6 – (– 1 / 7) + 1 / 9 = (– 1 / 7) + 3 / 4
When we solve both these sides, we get:
15 / 21 = 20 / 28
5 / 7 = 5 / 7
Here again, since both L.H.S and R.H.S of the equation are equal (5 / 7), you have the right answer. Now, following this, here are some equations for you to solve yourself:
● 5 + x / 3 = 7
● 1 / 2x − 1 = 5
● 5x + 1 / 2 − x – 2 / 6 = 2x + 4 / 3
● 3 / 4x – 2 = 1 / 3x + 3
● x + 2 / 3 = 1 − 2x / 5
Solving Another Form of Linear Equation in One Variable
After solving linear equations with fractional coefficients, we come to the final kind of linear equation we will solve today. These are those that at first, do not appear to be linear.
However, after some algebraic manipulation, they turn out to be linear equations. The example we will take for it is the following equation:
3 / 5 = 6 / x
To solve this one, we will need a common denominator. Here, the number we need will be divisible by 5 as well as the value x. So, we will choose 5x as the quantity used to multiply both sides of the equation.
Here is what we will get:
3 (5x) / 5 = 6 (5x) / x
In solving this, we will get the linear equation we need to solve:
3x = 30
From here we can readily determine the value of x as:
x = 30 / 3
x = 10
Now, just as we did in all the above examples, let us substitute x = 10 in 3 / 5 = 6 / x, and check our answer:
3 / 5 = 6 / 10
0.6 = 0.6
Since both L.H.S and R.H.S of the equation are equal (0.6), you have the right answer.
Let us take another example. For this, the equation is:
5 / 3x = 25 / 27
To solve this, we will invert each fraction, and multiply both sides by a common denominator 25. Here is what we get:
3x (25) / 5 = 27 (25) / 25
To solve it, we get:
3x (5) = 27
15x = 27
x = 27 / 15
x = 9 / 5
In case, you do not want to flip the fraction, you have to use 27x as the common denominator. So, when you multiply both sides with it, you get:
5 (27x) / 3x = 25 (27x) / 27
On solving this, you get 45 = 25x
From here, x = 45 / 25 = 9 / 5
Here again, let us substitute x = 9 / 5 in the original equation, and check our answer:
The original equation is:
5 / 3x = 25 / 27
When we substitute x = 9 / 5 in it, we get:
5 / 3 (9 / 5) = 25 / 27
Solving this we get:
0.9 = 0.9
Here again, since both L.H.S and R.H.S of the equation are equal (0.9), you have the right answer. Now, following this, here are some equations for you to solve yourself:
● 6x + 2 = 29 − 3x
● 3 / x + 1 = 6 / 5x – 1
● 8 / x = 2
Before we can solve the following example, we must first apply the distributive property. It is easy to make a mistake here, so make sure to distribute the number in front of the parentheses to all of the terms inside.
Example: 3(x+2) −1=x−3(x+1)
Solution:
To begin, distribute the 3 and -3 and collect similar terms.
3(x+2) −1 = x−3(x+1)
3x+6−1 = x−3x−3
3x + 5 = -2x –3
We can now add 2x to both sides. (Remember that subtracting 3x from both sides yields the same result.)
3x+5+2x = −2x−3+2x
5x+5 = -3
From here, we can solve the equation as we did with the other two-step equations.
5x+5−5 = −3−5
5x = -8
5x = 8
5 5
x = -8
5
Summarizing How to Solve One Variable Equation
Based on all the problems we have solved above, here are generic steps to solve linear equations in one variable:
- Firstly, remove brackets, if any, and bring the variable term (x) on one side and constants on another.
- Then, simplify the terms on each side and isolate the variable on one side completely.
- Lastly, simplify and write the answer.
- You can also substitute it in the original equation to check it.
Final Thoughts
So, this is all you need to know about one-variable equations, and how to set them up to solve them efficiently. We hope that after this, you will be able to recognize simple one-variable equations, solve them easily, and also, check if your solution is correct with the substitution method. Good luck!
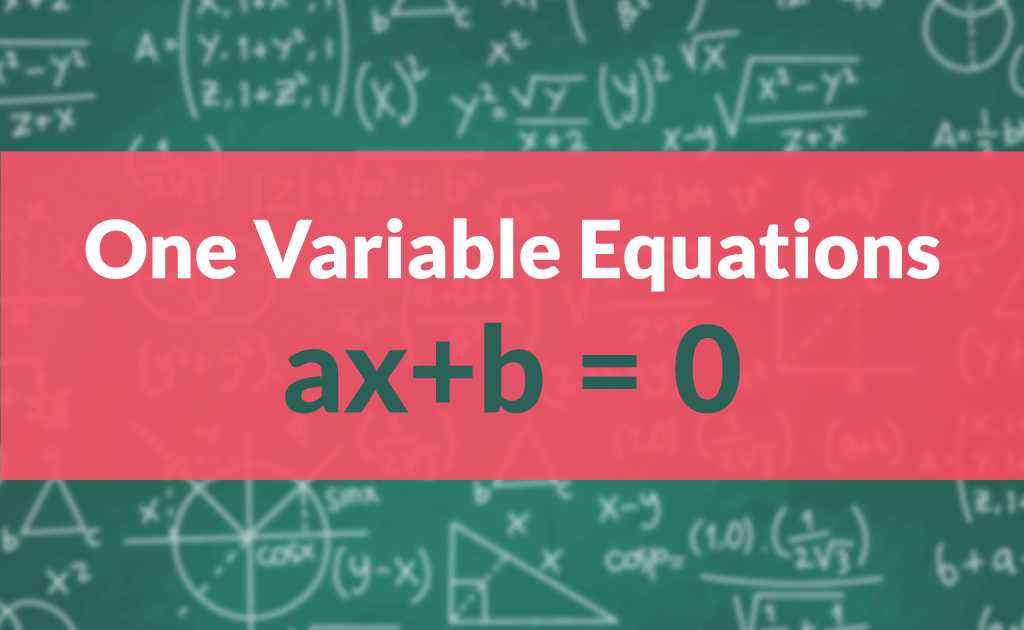
Relevant Articles
Convert Millimeters(mm) to Inches
In mathematics, length is measured in millimeters and inches. Before …
Convert Millimeters(mm) to Inches Read More »
Read More >>How to Choose Greater Than or Equal To?
Greater than or Equal to The greater than or equal …
How to Choose Greater Than or Equal To? Read More »
Read More >>Fibonacci Sequence Formula, Applications, With Solved Examples
Probably most of us have never taken the time to …
Fibonacci Sequence Formula, Applications, With Solved Examples Read More »
Read More >>Volume of a Cone – Formula with Solved Examples
In this article, Let’s explore the volume of a cone. …
Volume of a Cone – Formula with Solved Examples Read More »
Read More >>








Comments: