PEMDAS: What Does It Mean and Why Does It Matter?

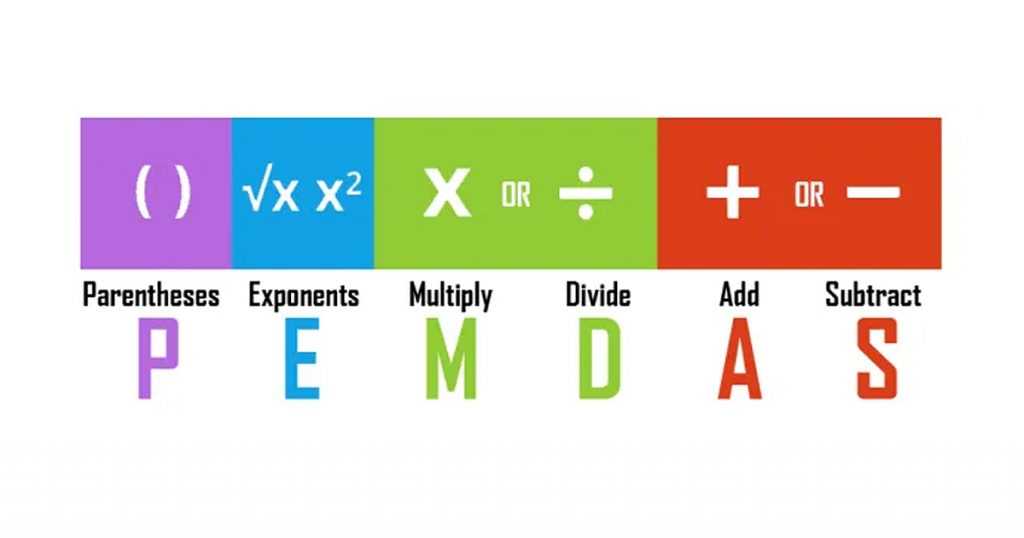
In mathematics, the order of operations is important to solve math problems that have more than one operation like addition, subtraction, multiplication, etc. To learn how to use the order of operations while studying mathematics correctly, students use a common rule called PEMDAS. It is an acronym used to remember the order of operations. But before going anywhere, let us understand what PEMDAS stands for.

PEMDAS Defines the Acronym for the Order of Operations. It stands for:

P: Parentheses – Anything in parentheses must be simplified first.

E: Exponents – The number will be in a square root that must solve after parentheses.

M: Multiplication – After parentheses and exponents, it’s time to solve multiplication.

D: Division – Division of numbers.

A: Addition – The addition of numbers comes after multiplication and division.

S: Subtraction – Subtraction comes after all the equations are solved.

Each letter depicts an operation in math. The order in which letters are organized shows you the order you need to solve different parts of a math problem. If any of these elements are missing (suppose you have a math problem without exponents or multiplication), you need to skip that step(s) and move on to the next one.

However, this process is only a general rule for memorizing the order of operations for math. There are also key nuances to the relationship between addition and subtraction and multiplication and division that must be reminisced with PEMDAS.

Why Does PEMDAS Important and How Can You Use It?

PEMDAS is not new as it has been around for a long time. Many math students learn this mnemonic with the phrase “Please Excuse My Dear Aunt Sally” to remember the order of operations in math. Although many consider it is not a perfect mnemonic for remembering the order of operations, it can be a helpful tool that you can use while solving math problems.

To use PEMDAS correctly, you always do parenthesis or groups first, then perform exponents, multiplication and division, and addition and subtraction.

PEMDAS refers to different scenarios where everything goes through multiple steps in a fixed sequence. Simplifying the answer to mathematical operations is somehow simple when only one operator is involved. But what if there are multiple operators? This is where PEMDAS has a pivotal role.

Let’s Say, for Example; you have to solve 5+2×4.

The problem has only multiplication and addition. So, as per the PEMDAS rule, you should do the multiplication and then addition:

5+2×4

= 5+8

= 13

When you choose addition first and then multiplication, the will be:

5+2×4

= 7×4

= 28

The final answer will be 28.

Let’s Have a Look at Different Problems 8 (6 − 2)² − 8 ÷ 4 + 6

You have one parenthesis (6-2), one exponent (the square root), multiplication, and division in this math problem. So, it would help if you solved parentheses first:

8 (4)² – 8 ÷ 4 + 6

The problem also carries one exponent – the square root. So, now we will solve it:

8×16 – 8 ÷ 4 + 6

Now, we have to perform multiplication and then division (8×16 and 8÷ 4):

128 – 2 + 6

In the final process, we need to do is to perform addition and then subtraction:

128 – 8

= 120

So, the final answer will be 120.

Let’s Check Out Another Problem  8 × 4 − 2 (4 – 2) ÷ 2²

This math problem comprises parentheses, an exponent, multiplication, division, and subtraction.

Start the equation by solving parentheses in first, meaning (4 -2):

8 × 4 − 2 (2) ÷ 2²

Then, solve exponent (the square root):

8 × 4 – 2 (2) ÷ 4

Now, we only have multiplication, division, and subtraction.

8 × 4 − 2 (2) ÷ 4

= 32 – 2 (2) ÷ 4

= 32 – 4 ÷ 4

= 32 – 0

= 32

The final answer will be 32.

One another problem is √25 (6 + 2)² − 18 ÷ 3 (4 − 2) + 3²

This problem might look intricate and boggle your mind when you sit to solve it. But PEMDAS makes it easy and simpler.

First, we need to solve parentheses (6 + 2) and (4 – 2):

√25 (8)² − 18 ÷ 3 (2) + 3²

Then, we should simplify components (8)² and (3)² with √25:

5 (64) − 18 ÷ 3 (2) + 9

Now, we only have multiplication, division, addition and subtraction. So, in first multiplication and division and then addition and subtraction, all from left to right:

5 (64) – 18 ÷ 3 (2) + 9

= 320 – 18 ÷ 3 (2) + 9

= 320 – 6 (2) + 9

= 320 – 12 + 9

= 308 + 9

= 317

So, the final answer is 317.

You can see how easy it is to solve any math problem with the PEMDAS rule. This rule will help you avoid arriving at the wrong answer if you mix up the order of operations. Correctly applying the order of operations using PEMDAS will improve your chance of getting a high score and minimizing mistakes.

The PEMDAS Rule Provides the Accurate Order of Operations for Simplifying any Math Problem with Expressions. In PEMDAS:

• P (parentheses) represents any grouping, including brackets, dashes and braces, and groups implied by radical and fractional expressions. This process comes first, and you should solve all groupings from inside to out.
• E (exponents) stands for both exponents and radicals.
• M (multiplication) and D (division) must be done from left to right. But it would help if you were careful when there are variables and alternate notations for products and quotients.
• An (addition) and S (subtraction) depict that additions and subtractions must be made last, from left to right.

Conclusion

PEMDAS is a very useful mnemonic and a set of rules for memorizing the order of operations when solving math problems. This rule is used to work out complicated multi-operation expressions. Many students remember this mnemonic using “Please Excuse My Dear Aunt Sally.” So, when you come across any math problem that you find complex, use this order of operations and implement them using PEMDAS.