Power of a Lens – Definition and Formula
Power of a Lens
Introduction
All the lenses do not converge or diverge the light rays falling on them by the same amount. Lenses of different kinds and different focal lengths act on a light ray falling on them differently. In this section we will look at the term which measures this mathematically.
Explanation
Power of a lens:
The converging ability of the lens varies inversely as the focal length of a convex lens and the diverging ability of the lens varies inversely as the focal length of a concave lens.
The degree of convergence or divergence of light rays achieved by a lens is expressed in terms of its power.
The power of a lens is equal to the reciprocal of the focal length of the lens.
Where P is the power of a lens and f is its focal length.
This means that, a convex lens of short focal length bends the light rays through large angles by focusing them closer to the optical center.
And a concave lens of very short focal length causes a higher divergence than the one with a longer focal length.
The SI unit of the power of a lens is dioptre (D).
If the focal length of the lens ‘f’ is expressed in meters, then the power is expressed in dioptres.
Therefore, 1 D is the power of a lens with focal length 1 m.
The focal length of a convex lens is positive whereas, the focal length of a concave lens is negative.
Therefore, the power of a convex lens is positive and that of a concave lens is negative.
Questions and Solutions:
- What is the power of the lens used in your spectacles? Calculate its focal length and identify the kind of lens used in it.
Solution:
Suppose the power of the lens in the spectacles is – 2.25 D.
P = 1/f
f = 1/P
f = 1/–2.25
f = – 100/225
f = – 4/9
f = – 0.44 m
f = – 44 cm
As the focal length turned out to be negative the lens used is a concave lens.
- What is the power of the lens whose focal length is 80 cm? Identify the kind of lens used in it.
Solution:
The focal length is given to be 80 cm = 0.8 m
P = 1/f
P = 1/0.8
P = 10/8
P = 1.25 D
As the focal length and the power, both are positive the lens used is a convex lens.
Summary
- The degree of convergence or divergence of light rays achieved by a lens is expressed in
terms of its power. - The power of a lens is equal to the reciprocal of the focal length of the lens.
P= 1 / f , where P is the power of a lens and f is its focal length. - The power of a lens varies inversely as the focal length of the lens.
- The SI unit of the power of a lens is “dioptre” (D). If the focal length of the lens ‘f is
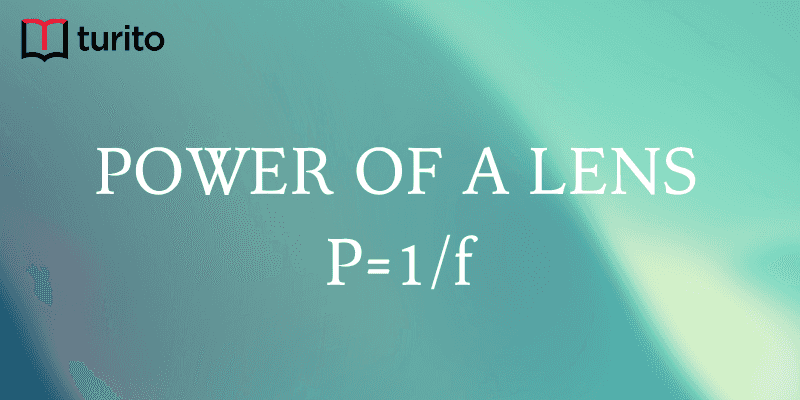
Related topics
Define Position Time Graph and its Types
Key Concepts • Slope of a graph • Position time graph • Slope of s-t graph = Velocity • Types of position time graphs Introduction An object in a uniform motion covers equal distances in equal intervals of time. This also indicates that it moves at a constant velocity. When its position at different instants […]
Read More >>Magnetic Field Lines: Definition, Explanation and Q&A
Key Concepts Magnetic Field Magnetic Field Lines properties of magnetic field lines Uniform and non uniform magnetic lines Introduction Two magnets when placed close to each other attract and stick to each other. However, if we go on increasing the distance between them, the attraction between them reduces gradually to such an extent that they […]
Read More >>The Life Cycles of Stars: Meaning and Example
Key Concepts Stars Analysis of starlight Composition of stars Stars’ temperature Size and mass of stars Stages of life cycle of a star Introduction Stars are huge, shining balls of extremely hot gas (known as plasma) in space. The Sun is our nearest star. During the nighttime, many other stars are visible to the naked […]
Read More >>Mirror Formula
Key Concepts New cartesian sign convention Mirror formula Solving problems using the mirror formula Introduction When dealing with the reflection of light by spherical mirrors mathematically, a set of sign conventions is followed, called the New Cartesian Sign Convention. According to this convention, the pole of a spherical mirror is taken as the origin and […]
Read More >>
Other topics
Comments: