The area of rectangle is one of the most important and popular parameters of a rectangle. In this article, we will cover major theories and mathematical formulas related to the area of a rectangle, like what is the area of a rectangle, the formula of the area rectangle, and its application in daily life.
Do we all know what a rectangle is? First, let us understand some fundamental concepts related to a rectangle. A rectangle is a closed figure made up of 4 sides, just like a square. But, unlike a square, a rectangle doesn’t have all sides equal. A rectangle is a four-sided figure where the opposite sides are equal and parallel. To understand this see the figure below:

You can see that the side marked as A is opposite and equal to the side marked as C. Similarly, the side marked as B is equal and opposite to the side marked as D. Hence, ABCD is a rectangle.
“You can also note that a square is a special case of rectangles with all opposite sides equal.”
We can find rectangles everywhere in our daily life. The notebooks you write on to books you study are rectangles. Some excellent examples of rectangular shapes are the tiles installed in your home, the blackboard teacher uses in the school, the dining table, the shape of your television screen, and much more.
Let us learn some of the mathematical terms and concepts related to a rectangle now:
- A rectangle has only opposite sides as equal and parallel. Two adjacent sides are never equal in the case of a rectangle.
- A rectangle is a parallelogram. This means that the opposite sides of a rectangle are equal and parallel to each other, or opposite sides never cross each other.
- The diagonal of a rectangle divides it into two triangles of equal area.
- The perimeter of a rectangle: The distance covered by the boundary of a rectangle is known as the perimeter of a rectangle. It is mathematically formulated as
P (rectangle) = 2 ( length + breadth)
Now that we are well versed with the basics of a rectangle let us find out the area of a rectangle and the formula of the area rectangle.
What is the Area of Rectangle?
Are we all familiar with the definition of “area” before delving further into the rectangle’s area? The space swept or covered by any closed figure is referred to as the area. The area of a figure is the area that lies inside the boundaries of that figure. This leads us to the conclusion that a rectangle’s area refers to the region it sweeps or covers. In other terms, the area of a rectangle is the two-dimensional region that lies inside its perimeter.
A figure is provided below. The area of the rectangle is indicated by the yellow-covered filled region, and its border is indicated by the red line.

Later in this article, we will see the formula of the area of the rectangle formula. Using that formula, you can even determine the area of the floor of your house, the area of your computer or mobile screen, etc.
How do you Find the Area of the Rectangle?
The number of unit squares that can perfectly fit inside a rectangle gives the area of that rectangle. You might have gotten confused with this definition, right? Don’t worry; let us clarify this for you.
For instance, let us make a rectangle with length = 2 cm and breadth = 3 cm. Let us now try to fit squares of length 1 unit inside this rectangle.
“Unit length: 1 is known as the unit length. The unit may be cm, inches, m, feet, etc. but remember whenever unit length is written, always understand it as 1.”
So squares of unit length mean that the length of each side of the square is one. As we can see in the figure below, 6 squares of unit length can easily fit inside this rectangle therefore, we can say that the area of the rectangle is 6 units. Also, we know that the sides of the rectangle are in cm; therefore, the area of the rectangle changes from 6 units to 6 cm.

We have completed all the conceptual learning in the area of rectangle. Next, let us study and derive the area of the rectangle formula.
Area of Rectangle Formula
The formula which we are going to learn now is one of the simplest formulas in mathematics. Despite being simple it is one of the most powerful formulas too. The area was found using this formula only from the table you are studying to the dwelling room.

The area of the rectangular formula is given as:
Area = length x width or length x breath
The product of the length and breadth of a rectangle gives us the formula for the area of that rectangle. Let the size of the rectangle be ‘a’ and breath be ‘b’; therefore, area ‘A’ is written as
A = a x b (square units)
Example: The length of a rectangular roof is 12 m, and the breadth is 7 m. Find the area of wood that would be used to cover the entire roof.
Solution: Given length ‘a’ = 12
breadth ‘b’ = 7
Area of wood required to cover the entire roof = Area of the roof
A (roof) = a x b
= 12 x 7
= 84 m2
Now that we have learned what the formula for the area of a rectangle is, let us learn how to calculate it.
Steps on how to Calculate the Area of Rectangle?
The steps on how to calculate the area of a rectangle are mentioned below. If you follow these steps correctly, you will never get errors in your solutions.
Step 1: Write down the dimensions of the given rectangle from the questions.
Step 2: Put the values in the area of the rectangle formula, i.e., length x width.
Step 3: Multiply the values and get the product.
Step 4: Write the result in square units
To further understand how to calculate the area of a rectangle, consider the following example. We’ll calculate the area of a rectangle with a length of 20 units and a width of 5 units.
Step 1: Given the length of the rectangle = 20 units, breadth of the rectangle = 5 units
Step 2: Area of rectangle formula = length x breadth
Step 3: 20 x 5 = 100
Step 4: The area of the rectangle is 100 square units.
Proving Area of Rectangle?
Throughout the entire article, we have been doing the area of a rectangle as ‘length × breadth’. But ever wondered why this is the formula? In this section, let us derive the area of a rectangle formula.
See the figure mentioned below. We can see a rectangle KLMN with a diagonal KM. This diagonal has divided the rectangle into two triangles of equal areas. Therefore the area of the rectangle = 2 x the area of 1 triangle.

Let us take the triangle KMN. The base KN of the triangle is the length of the rectangle, say ‘a, and MN the breadth of the rectangle is the height of the triangle, say ‘b’. Since KMN is a right-angled triangle (because the adjacent sides of a rectangle are perpendicular to each other)
We know the area of a triangle is = ½ x base x height
Therefore Area of KMN = ½ x a x b
Also, the area of the rectangle = 2 x (area of KMN)
= 2 x ½ x a x b
= a x b
Hence proved that the area of a rectangle is always its length x width.
Let us now suppose that we are not given the dimensions of the length of any rectangle; instead, we are given the length of any one of the diagonals and the breadth. How do we find out the area? We will find out in the next section of this article.
Area of Rectangle by Diagonal
As mentioned earlier, the diagonal divides a rectangle into two right-angled triangles. We shall use the Pythagoras theorem to determine a formula to find the area of a rectangle using diagonals.
According to Pythagoras theorem :
(Hypotenuse)2 = (Base)2 + (Perpendicular height)2
In this case:
Let diagonal be ‘d’, length be ‘l’ and breadth be ‘b’.
(Diagonal)2 = (Length)2 + (Breadth)2
(d)2 = (l)2 + (b)2
(l)2 = (d)2 – (b)2
l = √(d)2 – (b)2
Substituting the value of l in the main formula
Area of the rectangle = l x b
= {(d)2 – (b)2} x b
So the area of a rectangle when the length of one diagonal and the dimension of breadth are given is {(d)2 – (b)2} x b = {(Diagonal)2 – (Breadth)2} x Breadth
Therefore, you can use either of the methods to find the area of rectangle depending on the data you are given.
Solved Examples for Area of Rectangle
Example 1: A rectangular farm has a length and breadth of 80 yards and 60 yards, respectively. Find the farm area.
Solution:
The farm is 80 yards long and 60 yards wide.
Area of the farm A is: A = l w
= 60 yards x 80 yards
= 4800 square yards
As a result, the farm has a 4800 square yard space.
Example 2: A rectangular screen has a length of 15 cm. Its surface area is 180 square centimetres. Find its width.
Solution:
180 square centimetres is the size of the screen.
15 cm is the screen’s length.
Area of a rectangle = length x width.
Therefore, the area/length=width
Consequently, the screen’s width is equal to 180/15, or 12 cm.
Frequently Asked Questions
1. What is the Area of Rectangle in Geometry?
Ans. Area of a rectangle is a simple formula that you can use to find the area of a rectangle. It uses the length and width of a rectangle, which are its sides.
2. What is the Formula for the Area of Rectangle?
Ans. The formula for the area of a rectangle is A = l * w, where l is the length and w is the width.
3. What is the Unit of Area of Rectangle?
Ans. The unit of area of a rectangle is square meters.
4. Why Do We Calculate the Area of Rectangle?
Ans. The area of a rectangle is calculated by multiplying the width of the rectangle by its length.
5. How Can We Find the Area of Rectangle Using its Diagonal?
Ans. You can find the area of a rectangle using its diagonal. Just divide the number of square units by the number of diagonal units. For example, 4×5 has an area of 20 and a diagonal of 10, so we’d divide 20 by 10 to get 2, which is our answer.
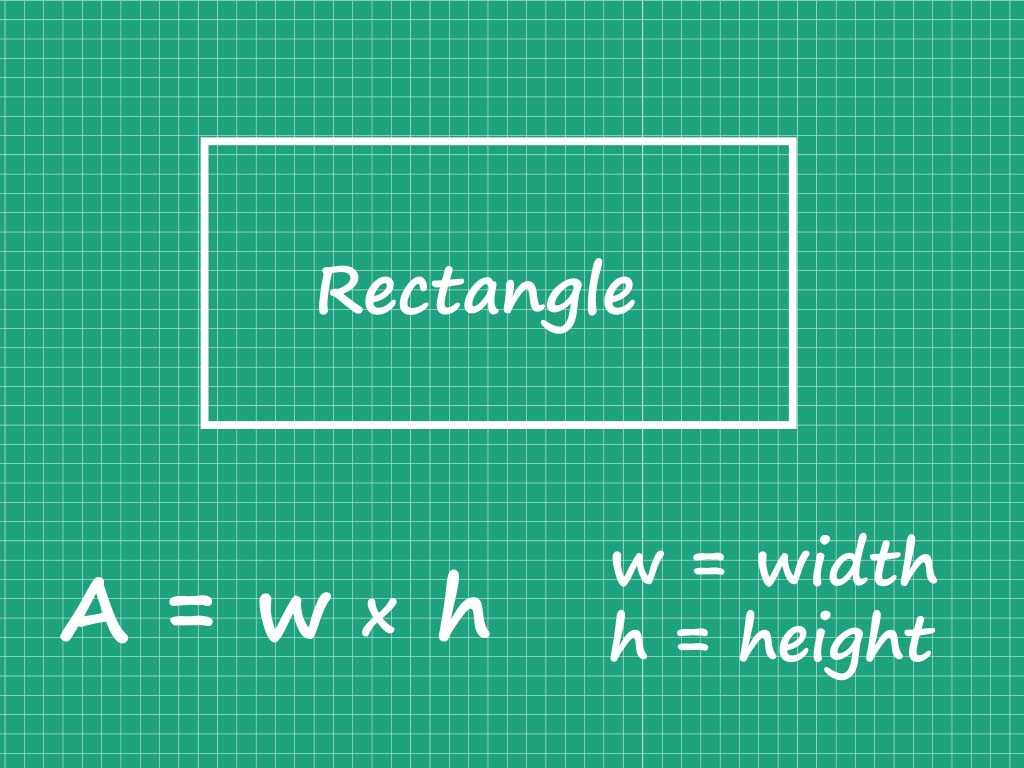
Related topics
Addition and Multiplication Using Counters & Bar-Diagrams
Introduction: We can find the solution to the word problem by solving it. Here, in this topic, we can use 3 methods to find the solution. 1. Add using counters 2. Use factors to get the product 3. Write equations to find the unknown. Addition Equation: 8+8+8 =? Multiplication equation: 3×8=? Example 1: Andrew has […]
Read More >>Dilation: Definitions, Characteristics, and Similarities
Understanding Dilation A dilation is a transformation that produces an image that is of the same shape and different sizes. Dilation that creates a larger image is called enlargement. Describing Dilation Dilation of Scale Factor 2 The following figure undergoes a dilation with a scale factor of 2 giving an image A’ (2, 4), B’ […]
Read More >>How to Write and Interpret Numerical Expressions?
Write numerical expressions What is the Meaning of Numerical Expression? A numerical expression is a combination of numbers and integers using basic operations such as addition, subtraction, multiplication, or division. The word PEMDAS stands for: P → Parentheses E → Exponents M → Multiplication D → Division A → Addition S → Subtraction Some examples […]
Read More >>System of Linear Inequalities and Equations
Introduction: Systems of Linear Inequalities: A system of linear inequalities is a set of two or more linear inequalities in the same variables. The following example illustrates this, y < x + 2…………..Inequality 1 y ≥ 2x − 1…………Inequality 2 Solution of a System of Linear Inequalities: A solution of a system of linear inequalities […]
Read More >>Other topics







Comments: