Universal Law of Gravitation – Introduction and Examples

In this article, we’ll explore the universal law of gravitation. Let’s begin

Introduction

By the late 17th century, Kepler and other astronomers described the orbits of the planets and the Moon well. Still, there was no single mathematical model to explain and predict them until Newton developed his model for universal gravitation.

Sir Isaac Newton said all things fall towards the Earth. He was puzzled by the problem that everything falls when released. It is believed that the sight of a falling apple made Newton wonder if the force that caused the apple to fall might extend to the Moon or even beyond.

Newton discovered that not only objects falling and the Moon but all objects in this universe attract each other, and the same force is responsible for this attraction. He called it a gravitational force. To understand this concept, let’s do one activity.

Activity

When a small object is tied and whirled around, the object moves in a circular path and changes its direction of motion at every point. We see that the force which is causing it to move in a circular path is always directed toward the center of the path. The force which is pulling the object toward the center of the circular path is known as the centripetal force.

The tension in the string will provide the necessary centripetal force. When the string breaks, the object moves along the straight line tangentially to the circular path.

Similarly, Newton concluded that the Moon revolves around the Earth in a circular orbit, and at each point of its orbit, the Moon is attracted by the Earth instead of moving in a straight line.

The necessary centripetal force is provided by the gravitational force exerted by the Earth.

Explanation

Inverse Relationship

In the year 1666, Newton studied Kepler’s work on planetary motion. He found that the magnitude of the force F on a planet due to the Sun varies inversely with the square of the distance r between the center of the planet and the Sun.

F α 1/r²

This force acts in the direction of the line connecting the centers of the two objects.

Proof:

The gravitational force follows the inverse square law; for example, if the weight of an apple on the surface of the Earth (radius = d) is 1N, as we increase the distance as 2d, 3d, 4d, and 5d, the weight of the apple becomes (1/4)N, (1/9)N, and (1/16)N.

Example:

Similarly, suppose the force between two bodies is 80N when they are at a distance of 0.1m. If we keep increasing the distance between the two masses, as shown in the table, we get a graph between force and distance, as shown in the figure

Direct Relationship

Based on his third law of motion and experimental findings, Newton concluded that the gravitational force of attraction between two objects must be proportional to the product of their masses.

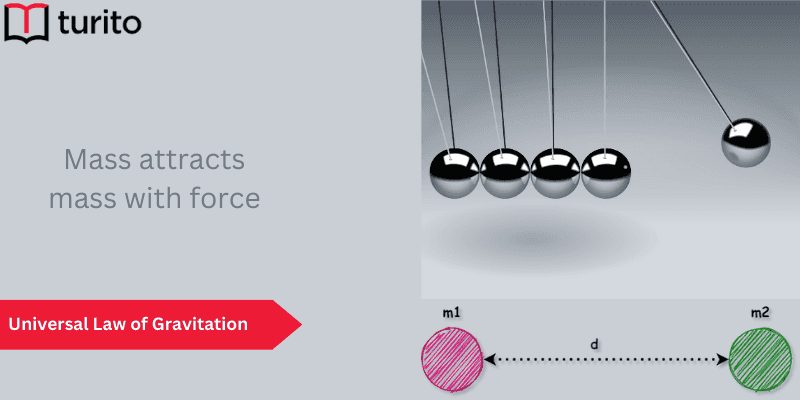
If A and B are two bodies with masses m₁ and m₂, separated by a distance d. The force F between two objects is given by,

F α m₁ x m₂

Thus. the gravitation force acting between two bodies depends on the product of the mass of the bodies and the distance between the two bodies. If we increase the masses, the force increases; if we increase the distance between the bodies, the gravitational force decreases.

The universal law of gravitation in mathematical form:

The figure below describes how the universal law of gravitation is derived mathematically.

F α m1————-(i)

F α m2————(ii)

From the above equation, we can rewrite them as the following:

If we remove the proportionality sign, we get proportionality constant G as the following:

The above equation is the mathematical representation of Newton’s universal law of gravitation.

Hence, G = Fr²/ m₁ m₂

SI Unit of G is: Nm² kg^-2

Value of G = 6.673 × 10^-11 Nm² kg^-2 (was found out by Henry Cavendish (1731- 1810))

The proportionality constant G is also known as the Universal Gravitational Constant.

Question: Why is Newton’s law of gravitation called Universal law?

Every object in the universe attracts every other object with a force proportional to the product of their masses and inversely proportional to the square of the distance between them.

The force is along the line joining the centers of two objects. The law applies to all bodies, whether the bodies are big or small, whether they are celestial or terrestrial. Hence, it is universal.

Newton’s Law of Gravitation in Vector Form

Newton’s third law tells the force that one object exerts on another object will experience an equal and opposite force due to interaction.

F_{a on b}  = – F_{b on a}

Forces are equal in magnitude but opposite in direction.

Newton’s law of gravitation shows the same thing when written in vector form.

The gravitational force of the Sun on Earth is:

The negative sign indicates that the force points back toward the Sun. The gravitational force of Earth on the Sun is :

This has the same magnitude as F_{S on E} but points in the opposite direction, towards Earth, so we can say that  F_{S on E} = -F_{E on S}, and both have magnitude

Nature of Gravitational force

• Gravitational force is a non-contact force.
• Gravitational force is always attractive in nature.
• Gravitational force acts because of mass.
• Gravitational force is a long-range force.
• It is always directed towards the center of mass.
• Gravitational force does not depend upon the medium between the objects.
• Gravitational force is the weakest fundamental force in nature.

Importance of Gravitational Force

The most crucial aspect of Newton’s mathematical model was its universality – Newton’s Law of Universal Gravitation can be applied to all objects with mass, from atoms to planets to galaxies.

• Gravitational force binds us to the Earth
• Gravitation causes tides due to the Moon and Sun on Earth
• The Moon revolves around the Earth because of gravity
• Planets move around the Sun because of gravity

Numerical-1:

Calculate the gravitational force that the Sun exerts on Earth. You will need the following data:

The mass of Earth = 5.97 x 10²⁴ kg, the mass of the Sun = 2.00 x 10³⁰kg, and the mean radius of Earth’s orbit around the Sun = 1.50 x 10¹¹m.

Solution-1:

M_E = 5.97 × 10²⁴ kg

M_S = 2.00 × 10³⁰ kg,

r = 1.50 × 10¹¹ m

Numerical-2

Calculate the gravitational force of attraction between the Moon and the Earth. For example, given the mass of the Earth is 5.972 × 10²⁴ kg, the mass of the Moon is 7.35× 10²² kg, and the distance between them is 384,400 km.

Solution-2:

Given that,

m₁ = 5.972 × 10²⁴ kg

m₂ = 7.35× 10²² kg,

r = 384,400 km

G = 6.673 x 10^-11 Nm²/kg²

r = 3.84 x 10⁵ km = 3.84 x 10⁸ m

F = G (m₁m₂/r²)

F = (6.673 x 10^-11)(5.972 × 10²⁴ x 7.35× 10²²)/ (3.84 x 10⁸)²

= 19.86 x 10¹⁹ N

F =1.986 x 10²⁰ N 

The gravitational force of attraction between the Moon and the Earth is approximately F =1.986 x 10²⁰ N, which is huge. So that is why it is apparent.