Add Fractions with Unlike Denominators – Complete Guide

Add fractions with different denominators is an advanced concept to learn after having sound knowledge about adding two fractions with the same denominator. It sounds hard but adding fractions with different denominators is easy. It is among the topics which are covered in elementary schools. This enhances the calculation capacity of the students. This concept is important because the students are often confused between adding like and unlike fractions. In this article, we will learn about how to add fractions with different denominators with a lot of examples.

We must be clear about what fractions with different denominators are before we learn about their addiction. Add Fractions with different denominators are known as, unlike fractions. For example, ¾ and ½ are unlike fractions. They are unlike because their denominators are different 4 and 2. 

Are 3/4 and 3/7 like fractions?

No! 3/4 and 3/7 are not like fractions. We can see that their numerators are the same, that is 3. But for the fractions to be like, their denominators must be equal, not their numerators. Thus the fractions given are not like fractions but unlike fractions. 

Let us first learn how to add fractions with the same denominators to understand the concept of addition of unlike fractions.

In Addition when the Denominators are the same

Like fractions are the easiest to add. It is exactly like adding two whole or non-fractional numbers. The method to add the like fractions is given below: 

Step 1: The first step is correctly writing the fractions with the ‘+’ symbol between them.

Step 2: We know that the denominator is the same in these fractions; therefore, the fractions, in addition, will yield the same denominator. 

Step 3: You have to add the numerators and note the result.

Step 4: Pin down the answer. Write the result of the addition p as the numerators, put a dash, and write q, which is the value of the denominator. The value of q is the same as the addends.

See the example below to understand the above steps.

Example: Add 2/11 with 3/11

Solution: Carefully following the steps mentioned above, we start the addition:

Step 1: Writing the numerators in proper form for addition, i.e., 2/11 + 3/11

Step 2: Since we are adding like fractions, the denominator of the outcome will be 11. 

Step 3: Now, we add the numerator terms to find the numerator of the result. 2 + 3 = 5.

Step 4: Finally, we write the resulting fractions as 5/11.

Here we cannot further simplify the fractions as 5 and 11 are not common multiples of any other number. Hence the answer is 5/11.

We are now well equipped with the knowledge about adding two fractions with the same denominators. Let us now learn about how we add fractions having different denominators.

Add Fractions with Different Denominators

The addition of fractions with not the same denominator is much tougher than the addition we studied in the above topic. The procedure of adding unlike fractions involves a lot of tips and tricks, which must be at your fingertips. Many students are often confused and make errors while adding, unlike fractions. 

Remember that LCM or Least Common Multiple is the key to solving the questions related to the addition of unlike fractions. At the same time, adding, unlike fractions, you need to find the LCM of the denominators. This is done to make a common denominator for the fractions. We can easily add the fractions with the steps mentioned in the above section when we have the same denominator. Look at the steps below to understand this method:

Step 1: The foremost step is to note the unlike fractions properly with the ‘+’ sign.

Step 2: Try to deduce the denominator for the resultant fraction by finding the LCM of the denominators. 

Step 3: Now, accordingly, multiply the numerators with the value by dividing the LCM by the individual denominator. Add the respective numbers to get the value of the resultant numerator.

Step 4: The last step is to pin down the result in the fractional form p/q. Where p is the total of the numerator and q is the denominator (LCM). 

Example: Add the unlike fractions 7/3 and 12/9

Solution: We will solve this using the stepwise approach mentioned above:

Step 1: Writing the inaccurate fractions form: 7/3 + 12/9

Step 2: Since they are unlike fractions, we have to find the LCM of 3 and 9. For this case, 9 is the LCM because both 3 and 9 are multiples. 

Step 3: Now, the next step is to multiply the numerators accordingly. For 7/3, 3 divides 9 3 times. Hence we will multiply 7 by 3, which gives 21. Similarly, for 12/9, 9 divides itself 1 time; hence 12 is multiplied by 1, which yields = 12 x 1. Now we add 21 with 12= 21 + 12 = 33. 

Step 4: Therefore, the resulting fraction is 33/9. It can be further simplified because 33 and 9 are multiples of 3. Thus the resulting fraction is 11/3. 

The LCM is taken to make them, unlike fractions into like fractions. The rest of the procedure remains the same. It is not essential to find the LCM of the denominators while adding or subtracting, unlike fractions. Any standard multiple will work fine. So we can get a common multiple by multiplying the two denominators. Although this produces larger-looking numbers, it may be reduced to its simplest form. For example, if the numbers are 3 and 9. Their LCM = 9, but we can take any value like 18, 27, 36, etc. Since we are dealing with the least common multiple, we shall consider the smallest value.

Can we add a Fraction with 0?

While adding a fraction with a whole number, we shall always consider making the whole numbers into their fractional form. Since we know that 0 is the smallest whole number, hence to add any fraction with 0, we must first change 0 into fractional form. This can be done by placing ‘1’ in the denominator’s place. 

The addition of any fractions with zero is an example of the addition of unlike fractions. The steps involved in the addition of fractions with zero are:

Step 1: Write the whole number zero as 0/1.

Step 2: Since the denominator in 0/1 is 1. The denominator after the addition will be the same as the denominator of the other number being added to 0.

Step 4: Add the numerators, which will be (x + 0) = x.

Step 5: Write the fraction in the form x/d. Where ‘x’ is the sum of the numerators and ‘d’ is the denominator of the number other than 0.

From the concept learned above, we can see that any fraction gives the fraction itself when added with 0. 0 + a/b = a/b.

Simplifying Negative Number Operations 

Use these guidelines when adding and subtracting positive and negative numbers when working with fractions, whole numbers, or decimals. 

Original 

	Operation	Operand Sign
Adding A Positive Number	+	+
Adding A Negative Number	+	–
Subtracting A Positive Number	–	+
Subtracting A Negative Number	–	–

Can we add a number by the Reciprocal of 0?

No! The reciprocal of 0/1 is given as 1/0. According to mathematics, 1/0 doesn’t exist. 1/0 is not defined. Hence we can say that any fraction added with the reciprocal of zero is not defined.  

Examples 

Add the following fractions. →

1) 3/10 + 13/11

Solution: We are all aware of the basic steps from the examples mentioned above. So let us directly begin the calculation.

3/10 + 13/11

The LCM of the denominators is 10 x 11 = 110.

We now know that 3 must be multiplied by 11, and 13 must be multiplied by 10 to get the desired numerator. Therefore,

3 x 11 = 33, and 13 x 10 = 130. Thus the numerator is 130 + 33 = 163.

The fraction after the addition of 3/10 and 13/11 is 163/110.

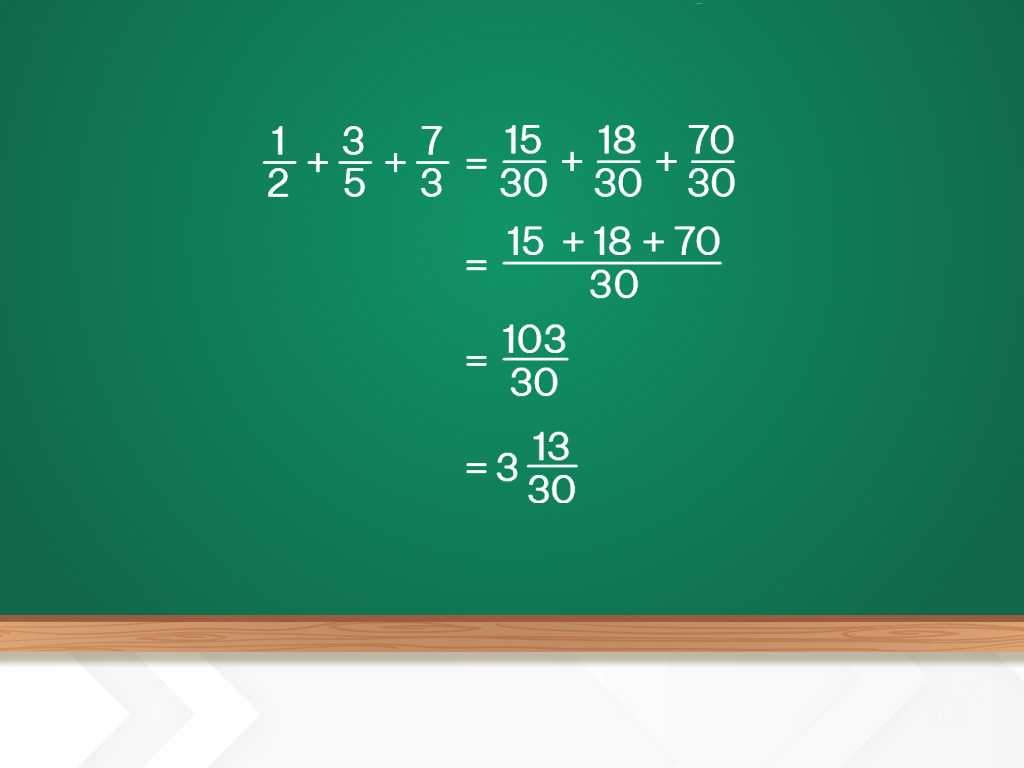
2) 1/2 + 8/3 + 11/10

Solution: The LCM, in this case, must be considered three denominators. Hence we need to find the LCM of 2, 3, and 10. 

The LCM comes out to be 30 because it is the least number that can be divided by 2 (15 times), 3 (10 times), and 10 (3 times). We know that ½ will be multiplied by 15, 8/3 will be multiplied by 10, and 11/10 will be multiplied by 3.

Thus the numerator becomes: 1 x 15 + 8 x 10 + 11 x 3 = 15 + 80 + 33 = 128.

Thus the resultant fraction is 128/30 → 64/15

Let us Summarize

Use the following procedures to add unlike fractions:

a) Convert the given fractions to like fractions by incorporating the LCM of the denominator.

b) Keep the denominator the same when adding the numerators.

c) Never add the numerators and denominators of unlike fractions separately. This will result in an incorrect result. Follow the guidelines outlined in each section of this article.

Frequently Asked Questions

1. How do I add fractions with uncommon denominators?

You can add fractions with uncommon denominators by:

Converting the fractions to a common denominator.

Adding the numerators of each fraction, then subtracting the resulting numerator from the denominator of each fraction.

2. How do you add two fractions with a different denominator?

It’s easy to add two fractions with different denominators. Just multiply the numerators and denominators together, then add the numbers from the numerator and the numbers from the denominator.

Example: 5/12 + 4/13 = 5 x 4/13 + 12 = 24/13

3. Do you add the denominator when you add fractions?

Yes, when you add fractions, you should include the denominator. This is because, to perform the addition, it is necessary to know how many pieces of the total amount are being added together.

4. How do you make denominators the same as two or more fractions?

To make the denominators of two or more fractions equal, you need to multiply each term by a common factor.

For example, let’s say we have 1/2 + 3/4. To make these denominators equal, we can multiply both terms by 2: 2(1/2) = 2 and 2(3/4) = 6. Now our numerators are 1 and 4, so the sum is 5.

5. What are the steps to add fractions?

1. Add the numerators of each fraction

2. Add the denominators of each fraction

3. Simplify if necessary