Additive Identity is one of the addition’s properties. The process of adding two or more numbers together is called addition. It is the total of all the numbers, in other words.
Mathematical operations play a key role in helping us do calculations swiftly and efficiently. We all know that numbers have values. But did you know that they could also have properties? Properties of numbers define the way that they function in arithmetic operations. But before we learn about these properties of numbers, let’s go over the types of numbers first to see what kinds of numbers have which properties.
Types of Numbers
Given below are the various types of numbers that you must know to perform additive operations:
- Natural Numbers: They are also known as counting numbers. They consist of positive integers from 1 to infinity. The set of natural numbers is denoted as “N” and includes the numbers 1, 2, 3, 4, 5, etc.
- Whole Numbers: They are also called non-negative integers. It does not include any fractions or decimals. “W” denotes the set, a, and the collection of whole numbers containing 0,1, 2, 3, 4, 5, and so on.
- Integers: These include all whole numbers and comprise a negative set of natural numbers as well. “Z” represents integers, and the integers are …-3, -2, -1, 0, 1, 2, 3…
- Real Numbers: All positive and negative integers, including fractional and decimal numbers, are called natural numbers. Represented by the symbol “R,” real numbers do not include imaginary numbers.
- Rational Numbers: These numbers can be written as a ratio of one number over another number. This means that rational numbers are any number that can be written in the form of p/q. The rational number is represented by the letter “Q.”
- Irrational Numbers: These numbers cannot be written as the ratio of one number over another number. The symbol “P” represents it.
- Complex Numbers: Complex numbers are numbers that include both real and imaginary numbers. They are represented by “C” as “a + bi,” where a and b are real numbers and “i” is an imaginary number.
- Imaginary Numbers: Imaginary numbers are complex numbers that can be written as the product of a real number and the imaginary number. Imaginary numbers are represented as “i.”
Properties of Numbers
Have you ever wondered how you can add up the numbers easily? What makes them get the same value even if you add them in multiple ways, such as 2 + 3 + 4 = 9, and 3 + 2 + 4 is also equal to 9. The properties of numbers are important to know, especially in arithmetic functions. They are listed below:
- Closure property
This property holds for the addition and multiplication of whole numbers. In addition, the closure property states that the sum of any two whole numbers will always be a whole number. If a and b are any two whole numbers, a + b will also be a whole number.
Example: 14 + 2 = 16, 9 + 8 = 17
In multiplication, it follows that the product of any two whole numbers will always be a whole number.
Example: 14 x 2 = 28, 9 x 8 = 72
This property also holds for the addition and multiplication of all real numbers. In addition, the sum of two numbers is always the same, regardless of the order in which they are added. In multiplication, the product of two numbers is always the same, regardless of the order in which they were multiplied.
Example: 1+ 6 = 7 or 6 + 1 = 7
1 x 6 = 6 or 6 x 1 = 6
This property follows that when three or more numbers are added, the sum will remain the same regardless of the order in which the numbers are grouped and added. It also follows that the product will remain the same regardless of the order in which the numbers are grouped and multiplied.
Example: 2 + (5 + 9) = 16 or (2 + 5) + 9 = 16
2 x (5 x 9) = 90 or (2 x 5) x 9 = 90
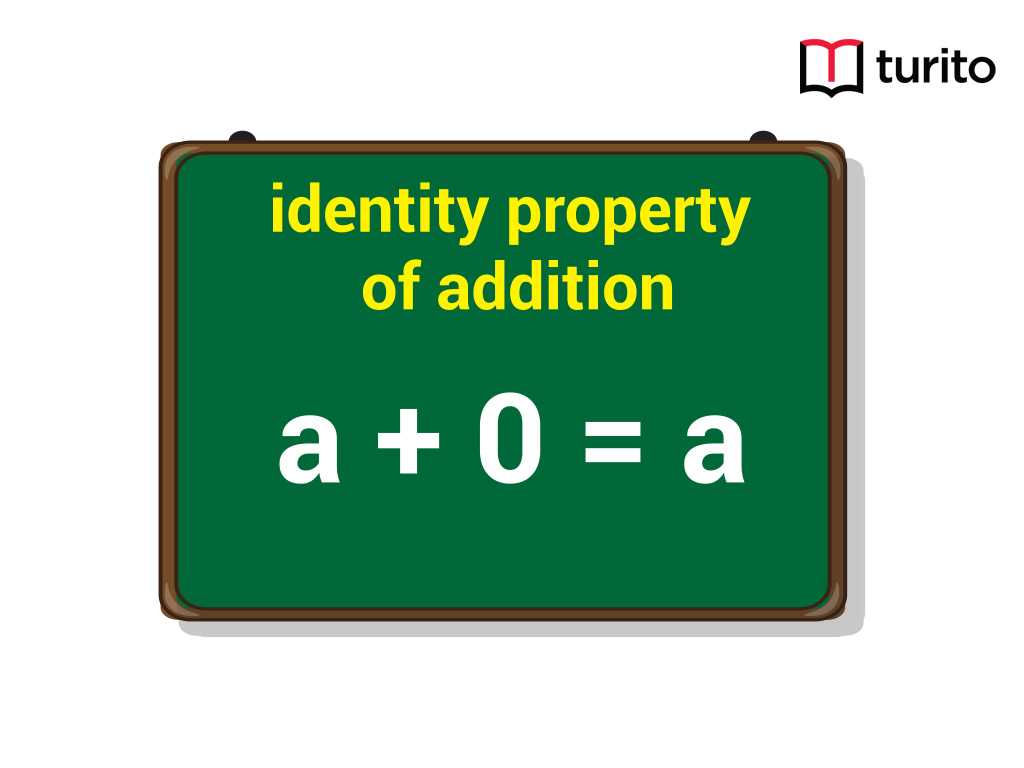
- Identity Property
This property follows for both addition and multiplication of whole numbers.
- What is the identity property of addition?
In addition, the identity property states that when zero is added to any number, the result is the number itself and does not change its value. Instead, it keeps its identity. Hence, the number 0 is known as the identity element or the additive identity in this property. This property can be used in any number, such as real numbers, integers, complex numbers, rational numbers, etc. However, it does not apply to natural numbers since natural numbers start from 1, and 0 is not a natural number. For any select set of numbers such as integers, rational numbers, and complex numbers. The additive identity is 0. This can be represented as:
a + 0 = a, 0 + a = a
Where a represents any number.
- Multiplicative identity property:
In the case of multiplication, the identity element of the additive identity is 1, and not 0. This is because any number that is multiplied by 0 will always give 0. The multiplicative identity property states that any number that is multiplied by the number 1 will always give that number as the product. Here, the property applies to natural numbers as well, since it starts from 1. This can be represented as:
A × 1 = a = 1 × a, where a is any real number.
This article will cover an in-depth explanation of the identity property of addition.
Fun fact: The additive identity of addition has another name! Guess what it is! It’s also known as the identity property of zero.
Identity Property of Addition Applications in Everyday Life
The Identity Property of Addition is majorly used by various scientists, engineers, doctors, and financial professionals in everyday life. Out of them, here are a few important applications that will help you understand why you should be thorough with the concept of additive property.
- Financial management: If you know only how to add and subtract, you will perform long mathematical calculations. By knowing the properties of mathematical operations, you can speed up your mind calculations, which will help you manage finance in everyday life.
- Home improvement: If you do not know how to calculate your purchased items or how much quantity you need of that particular item, you will purchase limitless quantities that will be spoiled over time.
- Fitness and health: Knowing additive properties and other properties will help you count your calorie intake and calorie burn. This way, you can manage your health, stay fit and progress toward your goals.
- Outdoor landscaping: You can renovate your home or even do gardening by applying simple mathematical operations. You will know how much soil you need or how much water you need to put in the plant pots. Knowing additive properties will help you create a healthy home and decrease stress by enhancing your memory.
Identity Property of Addition Example
Following are some solved examples using the additive identity property.
Identity property of addition example:
Q1. There are 6 children playing a game in the park. No other children joined the game till the end. How many children were playing in the park?
There were 6 children playing in the park. Since no other children joined the game, there was no change in the number of kids in the park.
So, 6 + 0 = 6. There were 6 children playing in the park.
Q2. A flock of 20 birds sat on a wall. There were no other birds that joined them. How many birds are sitting on the wall?
There are 20 birds on the wall. Since no other birds joined them, the number of birds remained the same.
So, 20 + 0 = 20. There are 20 birds sitting on the wall.
WORKSHEET
Use this worksheet to practice your skills in the identity property of addition:
- Which of the following represents the identity property of addition?
- 5+4 = 10 b. 12 + (-12) = 0 c. 8 + 0 = 8 d. 4+4 = 8
- Which of the following is the correct representation of the identity property of addition?
- 1 = 3
- 2 + 3 = 3 + 2
- 42 + (−42) = 0
- 75 + 0 = 75
- Fill in the missing numbers
- 234 + ____ = 234
- 0 + _____ = 92314
- 4798 + 0 = _____
- 9832 + ____ = 9832
- 34658 + 0 = _____
- 0 + _____ = 45793
- 28736 + 0 = ______
- _____ + 0 = 274
- 5078 + _____ = 5078
- 45 + 0 = ______
- Identify which of the four additive properties have been used in each of the following equations:
- a) (3 x 4) x 1 = 3 x (4 x 1)
- b) 2764 + 0 = 2764
- c) 7 + 9 = 9 + 7
Now, there you have it: the additive identity property of numbers. As mentioned earlier, this property is just one of many. As your skills in mathematics and particularly in algebra broaden, so will your knowledge of the different applications of these properties. These are just the stepping stones that will serve as a strong foundation for your journey in algebra.
Frequently Asked Questions
1. What is the additive identity of a number?
Ans. The additive identity of a number is the number is that, when added to any other number, results in that same number. For example, 0 is the additive identity for the set of integers, because adding 0 to any number will result in that same number (0 + 3 = 3).
2. Why is the additive identity a multiplicative absorbing element?
Ans. The additive identity is a multiplicative absorbing element because it is the only element that has no effect on the value of a given expression. In other words, when you add the additive identity to any expression, you get back the same expression.
3. Does the additive identity annihilate ring elements?
Ans. The additive identity annihilates ring elements, which means that it is the additive identity for a ring. It is also the multiplicative identity for a ring, because the product of a number by zero is always zero.
4. How do you find the additive identity of a matrix?
Ans. To find the additive identity of a matrix, add all the elements of the matrix together and then make sure that you have a zero in that position.
5. What are some examples of additive identity?
Ans. Additive identity is the value that, when added to a number, gives you back the original number. For example, 5 + 0 = 5, and -5 + 0 = -5.
Related topics
Addition and Multiplication Using Counters & Bar-Diagrams
Introduction: We can find the solution to the word problem by solving it. Here, in this topic, we can use 3 methods to find the solution. 1. Add using counters 2. Use factors to get the product 3. Write equations to find the unknown. Addition Equation: 8+8+8 =? Multiplication equation: 3×8=? Example 1: Andrew has […]
Read More >>Dilation: Definitions, Characteristics, and Similarities
Understanding Dilation A dilation is a transformation that produces an image that is of the same shape and different sizes. Dilation that creates a larger image is called enlargement. Describing Dilation Dilation of Scale Factor 2 The following figure undergoes a dilation with a scale factor of 2 giving an image A’ (2, 4), B’ […]
Read More >>How to Write and Interpret Numerical Expressions?
Write numerical expressions What is the Meaning of Numerical Expression? A numerical expression is a combination of numbers and integers using basic operations such as addition, subtraction, multiplication, or division. The word PEMDAS stands for: P → Parentheses E → Exponents M → Multiplication D → Division A → Addition S → Subtraction Some examples […]
Read More >>System of Linear Inequalities and Equations
Introduction: Systems of Linear Inequalities: A system of linear inequalities is a set of two or more linear inequalities in the same variables. The following example illustrates this, y < x + 2…………..Inequality 1 y ≥ 2x − 1…………Inequality 2 Solution of a System of Linear Inequalities: A solution of a system of linear inequalities […]
Read More >>Other topics







Comments: