Any polygon with equal sides and angles is said to be regular. Considering this, the method used to determine the area of regular polygons is based on the formulas assigned to each polygon.
A polygon is a plane figure described by a finite number of straight-line segments connected to form a closed polygonal chain (or polygonal circuit). The bounded plane region, the bounding circuit, or the two together may be called a polygon in Euclidean Geometry.
Some Important Terms and Information on Polygon:
- Sides – the segments of a polygonal circuit
- Vertices – the meeting point of two sides of a polygon
- Body – the interior of a polygon
- “Gon” refers to the sides of a polygon. A quadrilateral is a 4- gon.
- A polygon is a 2-dimensional figure.
- A regular polygon’s apothem (sometimes abbreviated as apo) is a line segment from the center to the midpoint of one of its sides.
- Interior Angle: The angle formed at a polygon vertex that lies inside the region enclosed by the polygon.
- Center of a Regular Polygon: point on the interior of a polygon from which all polygon vertices are equidistant.
- Congruent Polygons: Polygons whose sides are all congruent
- Exterior Angle: The angle formed at a vertex of a polygon that lies outside the region enclosed by a polygon.
The length of the sides, and diagonals, and calculating the angles, medians, perimeters, and areas of regular polygons are the curriculum of mensuration and geometry.
Some of the Popular Polygon Names (as per No of Sides) with Their Properties:
| Name | Sides | Properties |
| monogon | 1 | Not generally recognized as a polygon, although some disciplines such as graph theory sometimes use the term. |
| digon | 2 | Not generally recognized as a polygon in the Euclidean plane, although it can exist as a spherical polygon. |
| triangle (or trigon) | 3 | The simplest polygon which can exist in the Euclidean plane. Can tile the plane. |
| quadrilateral (or tetragon) | 4 | The simplest polygon can cross itself; the simplest polygon which can be concave; the simplest polygon can be non-cyclic. Can tile the plane. |
| pentagon | 5 | The simplest polygon can exist as a regular star. A star pentagon is known as a pentagram or pentacle. |
| hexagon | 6 | Can tile the plane. |
| heptagon (or septagon) | 7 | The simplest polygon is such that the regular form is not constructible with a compass and straightedge. However, it can be constructed using a neusis construction. |
Polygons Can Be Classified by Their Types of Convexity
- Convexity means that any line drawn through the polygon (that is not tangent to a side or corner) meets its boundary exactly twice. As a result, none of its interior angles are greater than 180°. Any line segment with endpoints on the boundary, on the other hand, passes through only interior points between its endpoints.
- Concave: Non-convex and straightforward. At least one interior angle is greater than 180°.
- Simple: the polygon’s boundary does not cross itself. Convex polygons are all simple.
- A star polygon is a polygon that regularly intersects itself. A polygon cannot be both a star and shaped like a star. The difference between this one and the following is to be kept in mind while we try to find the area of the regular polygon.
- Star-shaped: From at least one point, the entire interior is visible without crossing any edges. The polygon must be simple and can be either convex or concave. Convex polygons are all star-shaped.
- Non-convex: A line that meets its boundary more than twice can be found. A line segment exists between two boundary points that pass outside the polygon.
- Simple: the polygon’s boundary does not cross itself. Convex polygons are all simple.
- Concave: Non-convex and straightforward. At least one interior angle is greater than 180°.
- Self-intersecting: the polygon’s boundary crosses itself. The term complex is sometimes used in contrast to simple, but this can confuse the concept of a complex polygon as one that exists in the complex Hilbert plane and has two complex dimensions.
By Aspects of Equality & Symmetry
- Equiangular: It means that all of the corner angles are the same.
- Equilateral: This means that all of the edges are the same length.
- Regular: equilateral and equiangular in shape.
- Cyclic: all four corners are located on a single circle known as the circumcircle.
- Another way to define regularity is that a polygon is regular if it is both isogonal and isotoxal, or equivalently if it is both cyclic and equilateral. A regular star polygon is a non-convex regular polygon.
- Isotoxal or edge-transitive: All sides share the same orbit of symmetry. In addition, the polygon is equilateral and tangential.
- Tangential: This means that all sides of a circle are tangent.
- Isogonal or vertex-transitive: all corners are in the same orbit of symmetry. In addition, the polygon is cyclic and equiangular.
How to Find the Area of a Regular Polygon?
The areas of regular polygons are described by the area enclosed by the sides/boundary of the polygon. Subsequently, it could be said that to find the area of the regular polygon, one needs to calculate the region it occupies.
Difference Between Perimeters and Areas of Regular Polygons
Perimeter and area are often confused. However, their only similarity is that area and perimeter are not dependent on the interior angles of the polygon; rather, they depend on the sides’ length. The basic differences need to be understood first to eradicate the confusion between these two measurable identities.
| Criteria | Perimeter of Polygon | Area of Polygon |
| Definition | It is defined as the total length of the boundary of the polygon which can be obtained by adding the length of all its sides. | It is defined as the region of space enclosed by any polygon. |
| Formula | The perimeter of the Polygon = Length of Side 1 + Length of Side 2 + …+ Length of side N (for an N-sided polygon) | The perimeter of the Polygon = Length of Side 1 + Length of Side 2 + …+ Length of side N (for an N sided polygon) |
| Unit | meters, centimeters, inches, feet, etc. | (meters)2, (centimeters)2, (inches)2, (feet)2, |
Formula to find the area of the regular polygon
Formulae for some of the regular known polygons are given below
- Area of triangle = (1/2) × base × height
We can also find the area of a triangle if the length of its sides is known by using Heron’s formula, which is, area = √s(s−a)(s−b)(s−c)s(s−a)(s−b)(s−c), where s = Perimeter/2 = (a + b + c)/2, a, b, and c are the length of its sides.
- Area of rectangle = length × width
- Area of parallelogram = base × height
- Area of trapezium = (1/2) × (sum of lengths of its parallel sides or bases) × height
- Area of rhombus = (1/2) × (product of diagonals)
In a nutshell, how to find the area of each regular polygon:
| Name of the Polygon | Area Formula |
| Triangle | 1/2 × base × height |
| Square | side2 |
| Rectangle | length × width |
| Pentagon | 5/2 × side length × distance from the center of sides to the center of the pentagon 1/4×√5(5+2√5)×(side)2 |
| Rhombus | 1/2 × product of diagonals |
| Hexagon | (3√3)/2 × distance from the center of sides to the center of the hexagon |
How Do You Find the Area of a Regular Polygon If the Number of Its Sides Is Known?
Areas of regular polygons = (number of sides × length of one side × apothem)/2, where the length of apothem is given as the l/(2tan(180/n)) where l is the side length and n is the number of sides of the regular polygon.
- In terms of the perimeter of a regular polygon, the area of a regular polygon is Area = (Perimeter × apothem)/2, in which perimeter = a number of sides × length of one side.
Example: find the area of the regular polygon with five sides and each side is 10cm long
- As we have understood in the discussion above, all we need is the length of the side to solve this problem. The formula that we will apply would be – 1/4×√5(5+2√5)×(side)2
- Hence 1/4×√5(5+2√5)×(10)2= 172.05 square cm.
Example: Find the area of the regular polygon with three sides and with a 6 cm base and 10cm height l
- The triangle formula for finding area will be applied here since it is a 3gon :
1/2 × base × height
- Hence ½ x base x height = ½ x 6 x 10 = 30 square cm
With these examples, finding the area of each regular polygon becomes much easier with the implementation of the basic formulae given. Other formulae for advanced-level studies also talk about the interior and exterior angles and irregular polygons. The basics of finding the areas of regular polygons are covered here.
Frequently Asked Questions
1. What is the Area of Polygon Definition?
Ans. The area of a polygon is the measurement of the area it encloses. Because polygons are closed-plane shapes, their area is the space they occupy in a two-dimensional plane. The area of any polygon is always measured in square units.
2. How do Find the Area of Polygons?
Ans. To find the area of a polygon, you need to know how many sides it has and what shape it is.
The area of a polygon is equal to one-half the product of its perimeter and the length of one side. Here’s an example: calculate the area of a triangle with sides of 3, 7, and 5 units long. The perimeter equals 15 units long, so:
Area = ½ × 15 × (3 + 7 + 5) = 0.75 × 15 = 10.25 units²
3. How do you find the Area of Polygons with Vertices?
Ans. The area of a polygon is determined by multiplying the lengths of all its sides. The formula for finding the area of a polygon with n sides is:
Area = Sides^2
4. How do Find the Area of Regular Polygons?
Ans. To find the area of a regular polygon, you need to know how many sides it has and how many angles those sides make with each other, as well as whether or not it contains any interior angles that are not right angles. The formula for finding the area of a regular polygon is πr² where r is the radius of its inscribed circle and n is the number of sides.
5. What is the Formula to Calculate the Area of Regular Polygons?
Ans. The formula for calculating the area of a regular polygon is A = (n/2) * L * R, where n is the number of sides in the polygon, L is the length of one side of the polygon, and R is the radius of an inscribed circle.
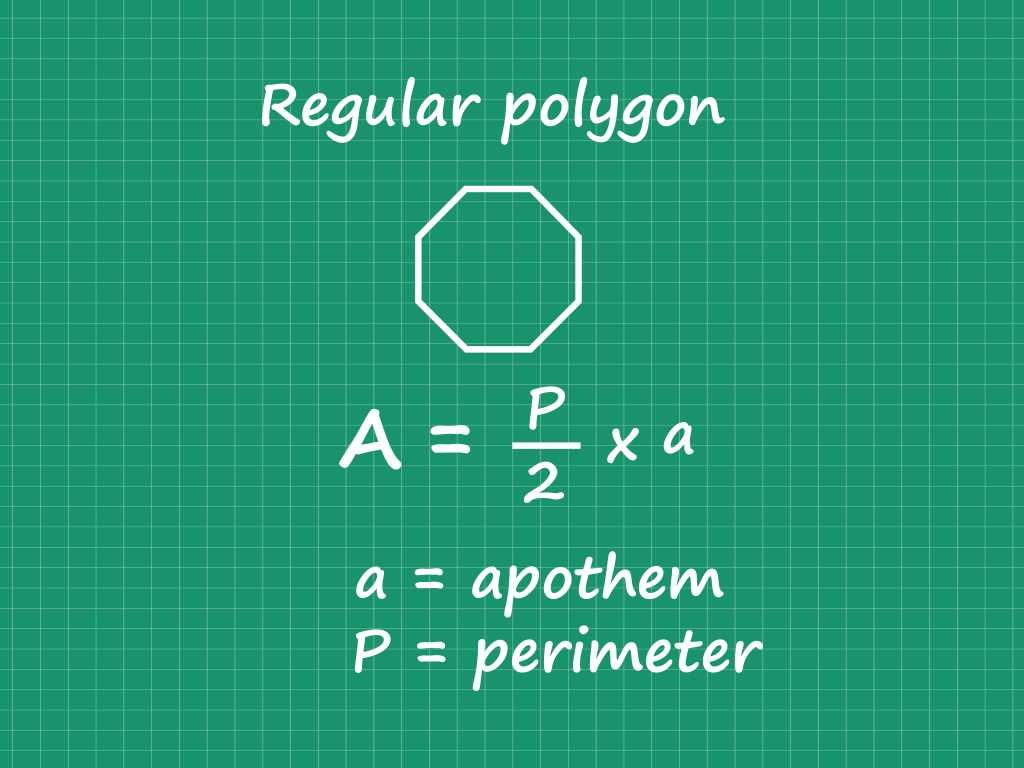
Related topics
Addition and Multiplication Using Counters & Bar-Diagrams
Introduction: We can find the solution to the word problem by solving it. Here, in this topic, we can use 3 methods to find the solution. 1. Add using counters 2. Use factors to get the product 3. Write equations to find the unknown. Addition Equation: 8+8+8 =? Multiplication equation: 3×8=? Example 1: Andrew has […]
Read More >>Dilation: Definitions, Characteristics, and Similarities
Understanding Dilation A dilation is a transformation that produces an image that is of the same shape and different sizes. Dilation that creates a larger image is called enlargement. Describing Dilation Dilation of Scale Factor 2 The following figure undergoes a dilation with a scale factor of 2 giving an image A’ (2, 4), B’ […]
Read More >>How to Write and Interpret Numerical Expressions?
Write numerical expressions What is the Meaning of Numerical Expression? A numerical expression is a combination of numbers and integers using basic operations such as addition, subtraction, multiplication, or division. The word PEMDAS stands for: P → Parentheses E → Exponents M → Multiplication D → Division A → Addition S → Subtraction Some examples […]
Read More >>System of Linear Inequalities and Equations
Introduction: Systems of Linear Inequalities: A system of linear inequalities is a set of two or more linear inequalities in the same variables. The following example illustrates this, y < x + 2…………..Inequality 1 y ≥ 2x − 1…………Inequality 2 Solution of a System of Linear Inequalities: A solution of a system of linear inequalities […]
Read More >>Other topics







Comments: