Key Concepts
- Area of a Rectangle
- Rectangles and Squares
Prior Knowledge:
There are 5 groups of oranges, and each group has 5 oranges.
How many oranges are there altogether?

Answer:
Given 5 groups of oranges, and each group has 5 oranges.

From the above figure,
5 groups of 5
= 5 × 5
= 5 + 5 + 5 + 5 + 5
= 25
∴∴
There are 25 oranges altogether.
13.1 Area of a Rectangle
Area:
The area of a figure is the amount of surface covered by the figure.

How do we find the area?
Let us consider a squared paper

In this squared paper, we make a rectangle like the following figure.

To find the area, we count the squares inside the rectangle.
Number of squares = 15
So, the area is 15 square units.
Here, the area will be equal to the number of squares.
Area = 15 square units
What does square units mean?
In our figure,

Unit means the size of 1 square
Unit can be anything like cm, m, km
Now, square units mean.
cm × cm = cm2
m × m = m2
km × km = km2
While calculating the area by counting squares, we follow these rules:
- If the area is less than half square, ignore it (take it 0)
- If the area is more than half square, take it 1 square unit
- If the area is exactly half square, take 12 square unit
Example 1:
Find the area of the squared figure.

From the above figure, we count the squares.
Number of squares = 25
So, the area = 25 square units
Example 2:


Find the area of the circle.
Find the area
of the circle.
So, the area = 4 + 8 + 2
= 14 square units
Example 3:
Find the area of the pentagon.


So, the area = 4 + 4 + 0
= 8 square units
13.2 Rectangles and Squares
Rectangles and squares are two of the most common shapes.
Here are a few examples of rectangular objects:

Examples of square objects:

Area of a Rectangle
To know the area of the rectangle, we count the number of squares it covers.
Another way is to multiply the length of the sides.
In a rectangle, opposite sides are equal.
The length of the sides that go from left to right is called width.
The length of the sides that go from top to bottom is called height or length.

To find the area of a rectangle, multiply the width by the height.
Area = width × height
Let us use this formula to find the width of the green rectangle.
First, know the width and height.

The width is 3 square units, and the height is 2 square units.
Now, multiply the width by height.
3 × 2 = 6 square units
If 1 square unit is 1 square meter, then 6 square units is 6 square meters (6 m²).
Area of a Square
A square has 4 equal sides.
This means that the length of the sides is the same.
To find the area of a square, multiply the length of 1 side by itself.

What is the area of this square?
The length of each side is 3.
3 × 3 = 9 square units
The area of the square is 9 square units.
If 1 square unit is 1 centimeter, then 9 square units is 9 square centimeter (9 cm²).
No Grids:
If there are no grids with squares to help you, you can still figure out the area of a shape.
To find the area of a rectangle,
length of width × length of height
To find the area of a square,
Multiply the length of any side by itself.
Perimeter
Perimeter is the length or distance around a shape.

How to find perimeter?
To find the perimeter of a shape, add the lengths of all the sides
Example 1:
The shapes below have the same perimeter, but a different area.
What is the area of the rectangle B?

From the above shapes, we have the height and the width of rectangle A. We can use these to find its perimeter.
We do that by adding the length of all its sides.
4 + 4 + 3 + 3 = 14 meters
i.e., the perimeter of rectangle B is also 14 meters.
We cannot figure out the area of B yet because the length of one side is still missing.
How do we find the missing side?
We know that the width is 2 meters.

Since opposite sides are equal, we double that to get the sum of two sides.
2 + 2 = 4
Now, we subtract the sum we got from the total perimeter.
14 – 4 = 10
This means that the sum of the two unknown sides is 10 m.
We divide it by 2, to get the length of each unknown side.
10 ÷ 2 = 5
Now we know that the height of rectangle B is 5 meters

Let us see if this is correct by comparing its perimeter with rectangle A’s perimeter.
5 + 2 + 5 + 2 = 14 meters
Now, we got the missing side of the rectangle B.
Let us find the area.
To find the area we multiply length and breadth of rectangle B.
∴∴
Area of the rectangle B = 2 × 5 = 10 square meters.
Example 2:
The rectangles below have the same area, but different perimeter.

What is the perimeter of rectangle A?
To find the perimeter of a rectangle, we need to know its height and width.
From the above shapes, we have width of the rectangle A, the height is missing.
How to find the height of rectangle A?
We know that the area of the two rectangles is equal.
First, we need to find the area of a rectangle B.
Area of a rectangle B = height × width
= 5 × 6 = 30 in2
∴∴
Area of the rectangle A = area of the rectangle B = 30 in2.
Now, we have to find the perimeter of the rectangle A.
We know that the width of rectangle A is 3 inches, and its area is 30 in2
A = H × W
30 = H × 3
H = 30 ÷ 3 = 10 inches
The missing side is 10 inches

∴∴
The perimeter of the rectangle A = 3 + 3 + 10 + 10 = 26 inches.

What we have leanrt:
- Understand the meaning of area
- Compare the area and perimeter of two figures
- Find the area of figures to solve real world problems
- Choose the appropriate tool and units of length to measure area
- Measure the area of surfaces of objects and places
Exercise
- Find the area of the shaded region.

2. Find the area of the shaded region.

3. A high school track is shaped like a rectangle with a semi-circle ( half a circle ) on each end. The rectangle has a length of 105 meters and a width of 68 meters. Find the area of enclosed by the track. Round your answer to the nearest hundredth.

4. Find the area of the shaded region. Round your answer to two decimal places.
Width – 17 feet
Length – 23 feet

5. Find the surface area and volume of rectangular solid with length 15yd, width 7yd, and
height 8yd.
6. A rectangular crate has a length 14 in, width 6 in, and height 10 in. Find its volume as surface
area.
7. What is the perimeter of this shape?

8. George wants to put a small fence around his garden. The garden is 17 feet long and 9 feet wide.
How much fencing does he need to buy?
9. If a regular pentagon has a side length of 7 inches, what is its perimeter?
10. The perimeter of a rectangular ink pad is 20 centimetres. It is 6 centimetres wide. How long is it?
Concept Map:


Perimeter of different shapes formulas:


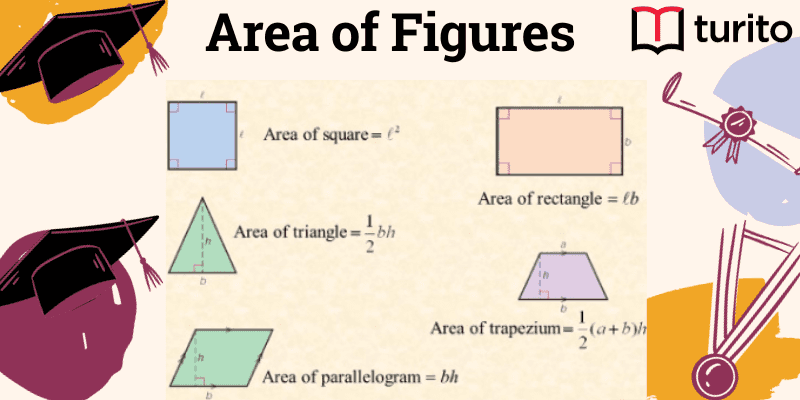
Related topics
Addition and Multiplication Using Counters & Bar-Diagrams
Introduction: We can find the solution to the word problem by solving it. Here, in this topic, we can use 3 methods to find the solution. 1. Add using counters 2. Use factors to get the product 3. Write equations to find the unknown. Addition Equation: 8+8+8 =? Multiplication equation: 3×8=? Example 1: Andrew has […]
Read More >>Dilation: Definitions, Characteristics, and Similarities
Understanding Dilation A dilation is a transformation that produces an image that is of the same shape and different sizes. Dilation that creates a larger image is called enlargement. Describing Dilation Dilation of Scale Factor 2 The following figure undergoes a dilation with a scale factor of 2 giving an image A’ (2, 4), B’ […]
Read More >>How to Write and Interpret Numerical Expressions?
Write numerical expressions What is the Meaning of Numerical Expression? A numerical expression is a combination of numbers and integers using basic operations such as addition, subtraction, multiplication, or division. The word PEMDAS stands for: P → Parentheses E → Exponents M → Multiplication D → Division A → Addition S → Subtraction Some examples […]
Read More >>System of Linear Inequalities and Equations
Introduction: Systems of Linear Inequalities: A system of linear inequalities is a set of two or more linear inequalities in the same variables. The following example illustrates this, y < x + 2…………..Inequality 1 y ≥ 2x − 1…………Inequality 2 Solution of a System of Linear Inequalities: A solution of a system of linear inequalities […]
Read More >>Other topics







Comments: