Constant of Proportionality – Definition With Examples

Do you think ratio and proportionality are similar to each other? They are not! Any relation that is constantly in the same ratio is referred to as proportionality. The quantity of mangos in a tree, for instance, is proportionate to the number of trees in the garden. The number of mangos present in every tree will be the ratio of proportionality. Before diving deep into the topic, let us learn about proportion.

The mathematical comparison of two numbers is known as proportion. How do you represent proportionality? The symbols “::” or “=” represent proportions. Proportion says if two sets of supplied numbers increase or decrease in the same ratio, the ratios are directly proportional to one another. When two ratios are equal, they are said to be in proportion. For example, the time for a bus to run at 60 kilometers per hour is equal to the time taken for the same bus to 360 kilometers in 6 hours. This can be seen as 60 km/hr = 360 km / 6. (Both the left-hand and right-hand sides of the expression are equal).

 Now that we have a basic idea about proportionality and have also revised the concept of proportions, let us study the constant of proportionality, inverse proportionality, and the law of multiple proportions.

Constant of Proportionality

 When two or more parameters are directly or indirectly proportional to one another, their relationship is expressed as a = kb or a = k/b, where k indicates how the two variables are related. This k is known as the proportionality constant.

The constant of proportionality is the ratio of two proportional values at a constant value. Two variable values have a proportional relationship when either their ratio or their product gives a constant. The proportionality constant’s value is determined by the proportion between the two specified quantities. This relation can be of two types direct variation and inverse variation. 

Direct Variation: The standard equation for direct variation is a = kb, which means that if ‘b’ increases, so do ‘a’, and if ‘b’ decreases, then ‘a’ also decreases. The best example of direct proportionality is the cost of products. The cost increases when demand increases and vice versa. It is expressed as a ∝ b. 

 Inverse Variation: The indirect proportionality equation is b = k/a, which means that as ‘b’ rises, ‘a’ falls, and the inverse is also true. This can be best illustrated by taking the example of a moving vehicle. The velocity of a moving vehicle (b) is proportional to its time to reach a certain distance (a), represented as b ∝ 1/a.

 In both circumstances, k is the same. The coefficient of proportionality is the value of this constant. The unit rate is another name for the proportionality constant. 

Application of Constant of Proportionality?

Have you ever imagined the use of proportionality in real life? In mathematics, we utilize the constant of proportionality to calculate the rate of change while also determining whether we are dealing with direct or inverse variation. Assume that the price of 4 oranges is $40. It automatically strikes our mind that one orange costs $5. The Constant of Proportionality for the price of orange has been determined to be 5.

 Likewise, if we wish to draw a picture of the Statue of Liberty while sitting in front of it on a piece of paper and gazing at the real monument in front of us, we must keep the length, height, and width of the structure proportional. To achieve the required result, we must first determine the proportionality constant. We may then draw the monument with proportional measurements based on this. For example, if the statue’s height is 93 meters, then to replicate it on our drawing sheet, we must draw it about 93 millimeters. In the same way, we will draw every component that can be done by applying the proportionality constant.

 Dealing with proportional relationships helps you to tackle a variety of real-world issues, including:

• Changing the proportions of ingredients in a recipe
• Finding odds and probabilities of events, for example, is a method of quantifying chance.
• Scaling a graphic for architectural and drawing purposes
• Calculating price mark-ups in terms of percent increase or percent reduction
• Product discounts depending on unit rate

Law of Multiple Proportions

According to the law of multiple proportions, the combination of 1 element with the definite amount of the second element is always a whole number. This law has many applications in Physics and Chemistry.

For example, the mass of oxygen in carbon dioxide (CO₂) is 32, and carbon monoxide (CO) is 16. Thus the mass of oxygen is in the ratio 32:16 or on simplification 2:1.  

Finding The Constant of Proportionality?

Determining the constant of proportionality is extremely easy. Earlier, we learned about what inverse and direct variation are. We will apply this knowledge to find the value of the constant of proportionality ‘k’. 

 For direct proportion: To find the value of ‘k’ in a direct proportionality, use the formula b = ka. From this formula k = b/a.

 For indirect proportion: To determine the value of ‘k’ in indirect proportionality, use the formula b = k/a. From this formula, k = ba.

 Let us learn how to determine ‘k’ with the help of some examples:

Example 1: The variables’ a’ and ‘b’ are directly proportional. Find the value of the constant of proportionality if a = 7 and b = 49, 

 Solution: Given that b = 49 and a = 7

‘b’ is directly proportional to ‘a’. Therefore the formula we will use is b = ka.

k = b / a

k = 49/7

k = 7.

Thus the constant of proportionality for this question is 7. 

Example 2: 6 students can complete the assignment in 3 hours. The teacher added 3 more students to the group to finish the assignment early. Find how much time it will take for the new group to complete the assignment.

 Solution: Given that six students can complete the assignment in 3 hours in case 1. 

The number of students increases by 3 in case 2. Now the total number of students is 6 + 3 = 9. 

Suppose the number of students increases, and the number of hours to complete the work will decrease. Hence we can say that this is an indirect relation. 

We will use the relation b = k/a → k = ab 

For case 1 

k = 6 x 3 = 18

For case 2 

k = ab

18 = 9 x b 

b = 18/9 

 Thus 9 students take 18/9 = 2 hours to complete the given work. 

How to Identify The Constant of Proportionality?

Hitherto, we have learned how to find the constant of proportionality in problems. But, what if we are provided with tables and graphs and are asked to find the constant of proportionality just by looking at them. Well, don’t worry. This section will explain how to identify the constant of proportionality in tables and graphs.

Number of hours  = a	1	2	5	7
Number of words written = b	200	400	1000	1400

From the table above, you can infer that as the number of hours increases, the number of words also increases. Hence, this is a direct relation. We can say that the number of words written ∝ the number of hours. Thus to determine ‘k’, we use b = ka.

k = b/a → 200/1 or 400/2 or 1000/5 and so on.

k = 200

Thus the constant of proportionality is 200.

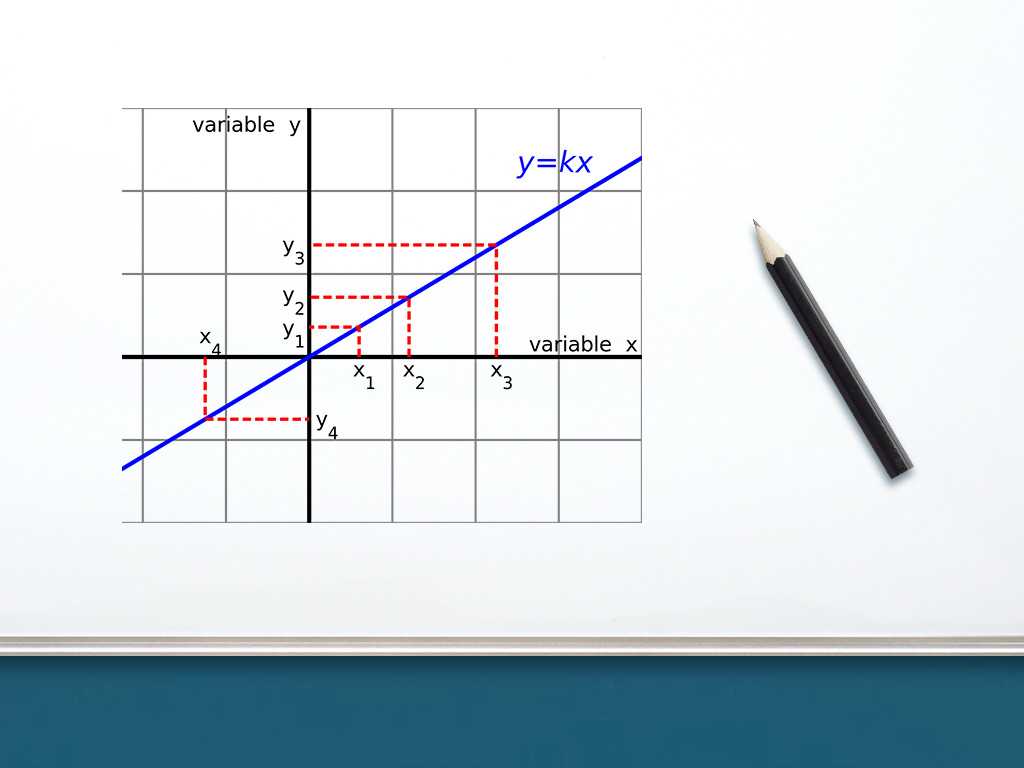
When you plot the values of the above table on a graph, you get a straight line that passes through the origin. This displays a proportional relationship between the two values as a direct variation. The slope of the line drawn for two proportional constants, a and b, on a graph is the constant of proportionality under the direct proportion condition.

If you have an indirect variation for the values in a table, you don’t get a straight-line graph. The inverse variation graphs are curvy, like a parabola or hyperbola. Usually, a graph of inverse variation has the starting point with the maximum value, which decreases to the minimum value and vice versa. 

Note: To determine whether the two quantities are proportional, we must calculate the ratio of the two numbers for each of the given values. They have a proportional relationship if their ratios are equivalent. The relationship between the ratios is not proportional if they are not all equal.

Frequently Asked Questions

1. How do you explain proportionality?

Ans. Proportionality is a concept that explains how two variables relate to each other. For example, if you have a variable A and a variable B, and A changes by 10%, then B should change by 10%. In other words, the change in A is proportional to the change in B.

2. What is the Constant of Proportionality?

Ans. The constant of proportionality is the value that determines the slope of a linear graph. The constant of proportionality is a constant because it remains the same throughout the entirety of a graph.

3. What is the purpose of proportionality?

Ans. The purpose of proportionality indicates that two quantities or variables are related in a linear manner. If one quantity doubles in size, so does the other; If one of the variables diminishes to 1/10 of its former value, so does the other.

4. How do you find the proportionality?

Ans. To find the proportionality, you need to divide both sides of the equation by the same number. This will make it easier to solve one of the variables in the equation.

5. What is the Constant of Proportionality in a Graph

Ans. A constant of proportionality is a constant factor that does not change the slope of a line. A graph can have more than one constant of proportionality, and a straight line will represent each one.