Key Concepts

• Fraction

• Decimal number

• Repeating decimal

• Non-repeating/terminating decimal

Real Numbers: Rational Numbers as Decimals

Introduction to Decimal Numbers: 

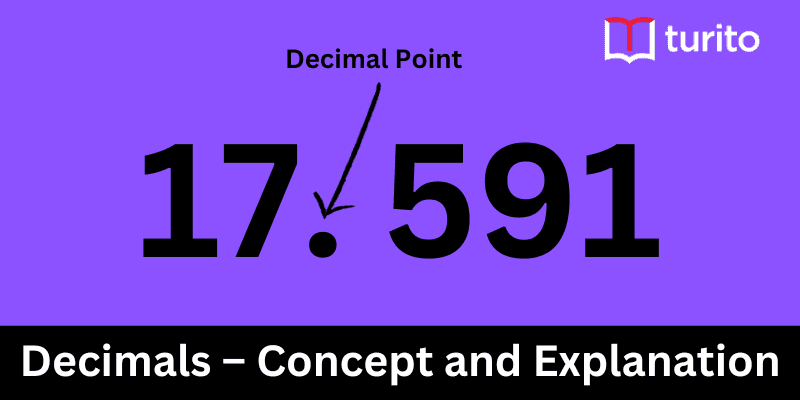
A decimal number can be defined as a number whose whole number part and the fractional part is separated by a decimal point.  

The dot in a decimal number is called a decimal point.  

The digits following the decimal point show a value smaller than one. 

Understanding the parts of a decimal number: 

Representation of decimal numbers on a scale: 

Decimal numbers in everyday life: 

Writing decimals as fractions: 

To convert a decimal to a fraction, we write the decimal number as a numerator and its place value as the denominator. 

Example 1: 0.07 

1.1.1 Writing repeating decimals as fractions 

Steps to writing a repeating decimal as a fraction: 

Begin by writing x = the repeating number. 

Multiply both sides of the equation by a power of 10, which will move the decimal to the right of the repeating number. 

Subtract the equation from step 1 from the equation in step 2. 

Solve the resulting equation. 

Example: 

Write 

0.₇⁻

as a fraction. 

Step1: Begin by writing x = the repeating number. 

x=0.7−

Step 2: Multiply both sides of the equation by a power of 10, which will move the decimal to the right of the repeating number. 

The repeating number is seven. To move the decimal to the right of the 7, you need to multiply by 10. This gives you the following: 

10x= 7.7−

Step 3: Subtract the equation from step 1 from the equation in step 2. 

 10x= 7.7−

−   x=0.7−9x=7

Step 4: Solve the resulting equation. 

9x=7

  x=79

1.1.2 Repeating decimals with non-repeating digits as fractions 

A mixed repeating decimal is a decimal that does not repeat until after the tenths place. The value 

712

=0.583− is an example of this. To write a mixed repeating decimal, you will use the same steps as before. 

Begin by writing x = the repeating number. 

Multiply both sides of the equation by a power of 10, which will move the decimal to the right of the repeating number. 

Subtract the equation from step 1 from the equation in step 2. 

Solve the resulting equation.  

Example 

Write

0.86−

as a fraction. 

Step 1:  Begin by writing x = the repeating number. 

x= 0.86−

Step 2: Multiply both sides of the equation by a power of 10, which will move the decimal to the right of the repeating number. 

The repeating number is six. To move the decimal to the right of the 6, you need to multiply by 100, which gives you the following: 

100x= 86.6−

Step 3: Subtract the equation from step 1 from the equation in step 2. 

100x= 86.6−

−    x=0.86−   99x=85.8−    x=0.86-   99x=85.8

Step 4: Solve the resulting equation. 

  99x= 85.8  99x= 85.8

       x=85.8   99       x=85.8   99

Since your answer has a decimal in the fraction, you must multiply the numerator and denominator by a power of ten, producing an equivalent fraction with no decimals. Multiplying the numerator and denominator by 10 gives you your answer: 

       x=858990       x=858990

We can reduce this fraction to 

429/495=143/165=13/15

1.1.3 Decimals with multiple repeating digits as fractions  

Let us see how to simplify a repeating decimal like 0.63636363… 

Step-1: Let x = recurring number 

X = 0.63 

Step-2: Two digits (63) are repeating.  

So, multiply both sides by 10², i.e., 100. 

100x = 100

××

0.63… 

100x = 63.63 

Step-3: Subtract x from left side and 0.63… from right side 

100x – x = 63.63 – 0.63 

99x = 63 

Step-4: Solve for x 

99x = 63 

X = 63/99 

X = 7/11

Exercise:

Exercise:

1. Rewrite as a simplified fraction
   0.2 ̅= ?
2. Rewrite as a simplified fraction
   2.6 ̅=?
3. Rewrite as a simplified fraction
   1.83 ̅=?
4. Convert the fraction 4/3 into decimals. If necessary, use a bar to indicate which digit or group of digits repeat.
5. Convert the fraction 7/6  into decimals. If necessary, use a bar to indicate which digit or group of digits repeat.
6. Convert the fraction 10/9  into decimals. If necessary, use a bar to indicate which digit or group of digits repeat.
7. Convert the fraction 8/7  into decimals. If necessary, use a bar to indicate which digit or group of digits repeat.
8. Convert the fraction 5/3  into decimals. If necessary, use a bar to indicate which digit or group of digits repeat.
9. Convert the fraction 7/3 into decimals. If necessary, use a bar to indicate which digit or group of digits repeat.
10. Convert the fraction 11/6 into decimals. If necessary, use a bar to indicate which digit or group of digits repeat.
11. Convert the fraction 8/3 into decimals. If necessary, use a bar to indicate which digit or group of digits repeat.
12. Convert the fraction 13/6 into decimals. If necessary, use a bar to indicate which digit or group of digits repeat.
13. Convert the fraction 11/3 into decimals. If necessary, use a bar to indicate which digit or group of digits repeat.

What we have learned:

1.1 About decimal numbers, parts of a decimal number, representation of decimal numbers on a scale, application of decimal numbers in everyday life, writing decimals as fractions and How to write repeating decimals as fractions.

1.2 How to write repeating decimals with non-repeating digits as fractions.

1.3 How to write decimals with multiple repeating digits as fractions.

Concept Map: