Equation and Graph of a Circle

The Equation and graph of a circle are the only shapes with no edges. This makes them unique and a good topic of discussion. Many concepts were discovered when the circles were drawn on a graph. The circle equation is required to draw it on the graph. In this article, we shall learn how to equation and graph of a circle, the equations, and the concepts needed.

Before understanding the concept of the equation and graph of the circle, let us learn some important terms required to understand this article’s topics. First, let us understand the concepts of circles. 

A circle is a closed figure which has no sides. It is like a curveball. The parameters inside a circle are radius, chords, perimeter, and area. The entire circle is drawn around the radius.

When we plot any circle in a graph, we require two to four parameters. The radius of a circle is the most essential for plotting it. The distance from the center of the circle to any point on the circumference is always equal. Thus we can say that a circle is a uniform figure.

Table of Content 

Equation of a Circle 

Center Radius form 

How to Graph a Circle Equation 

Frequently Asked Questions 

Equation and Graph of a Circle

We require two things if we want to equation and graph of a circle on the cartesian plane. They are mentioned below:

1. The coordinates of the center point, and 

2. The radius of a circle. 

Since we know that a circle is a set of different data points equidistant from a given point (the center of the circle), the equation of the circle will be around this point. The equation will be formed around the radius of the circle. The next parameter we need to complete the equation of any circle is the point from which the circle passes. Any point on the perimeter of the circle may suffice. 

From the above points, we now have basic information about the requirements for the equation of the circle. The standard equation of a circle is formulated as:

(x – k)² + (y – m)² = r². 

In this equation, k and m are some random points from which a circle is passing. ‘r’ is the radius of the desired circle. This form is also known as the centre-radius form because it is based on the radius of a circle.

Center-Radius Form

The center-radius form is the most applied form of finding the equation of a circle. As discussed above, this form revolves around the radius of the desired circle. Using this method, you can always test the equation required to graph a circle. The four attributes of this method are listed below:

• From the formula, we can see that the x and y (which are the variable terms) must be squared.
• No term in the expression remains negative. Every term is positive due to the squaring of each term. That is the reason why each term is written in parentheses.
• The value of the radius is also squared. Since measurements can not have negative values and radius is a measurement, it is understood that the radius must be positive. 
• The center point or the point from which the circle passes, say (h, k).

To form the equation ‘h’ is placed with the x variable and k is placed with the k variable, (x – h)² and (y – k)². The sum of these two terms must be equal to the square of the radius. This is formulated as:

  (x – h)² + (y – k)² = r² 

Here r² is a constant. This value is never found to be invariable. The only values that change in this equation are the values of h and k. They depend on the point from where the circle passes. 

We are now aware of the equation of the circle with a particular radius. Let us look at an example to understand this thoroughly.

Example 1: The radius of the circle is given as 5 m and a point from where the circle passes is given as (3, 4). Find the equation of the circle.

Solution: Given radius ‘r’ = 5

                                     h = 3

                                     k = 4

The equation of the circle is given as 

(x – h)² + (y – k)² = r²

(x – 3)² + (y – 4)² = 5²

(x – 3)² + (y – 4)² = 25

Example 2: A circle passes through points A (5, 3) and B(2, 9). Find the radius and the equation of the circle.

Solution: First, let us try to deduce the radius of the circle.

We know the formula for the equation of circles is:

(x – h)² + (y – k)² = r²

We are also given two points, the x coordinates are 5 in A and 3 in B. Likewise, the y coordinates are 3 in A and 9 in B. So placing the values we will get 

(5 – 2)² + (3 – 9)² 

(3)² + (-6)²

9 + 36

45

Hence r² = 45

Therefore, the radius of the circle is r = 45 

Now that we know the value of the radius, taking any point will make the equation of the circle.

When we take point A (5, 3) the equation is (x – 5)² + (y – 3)² = 45.

When we take point B (2,9) the equation is (x – 2)² + (y – 9)² = 45.

Both the equations are correct for the circle passing through A (5,3) and B (2,9) having a radius of 45.

How to Equation and Graph of a Circle

We have learned how to equate a circle in the above sections. From here onwards, we shall look at the methods to draw or depict these circles and their equations on a graph. 

Drawing a circle on a graph is very easy. A circle is simply a curve passing through the x and y axes. We need to know the following parameters to draw a circle on the graph.

• The value of the point (center point) from which the graph has to be made. 
• The radius of the circle. We must be aware of the size of the circle we intend to make.

Let us learn sketching of circular graphs in the next section:

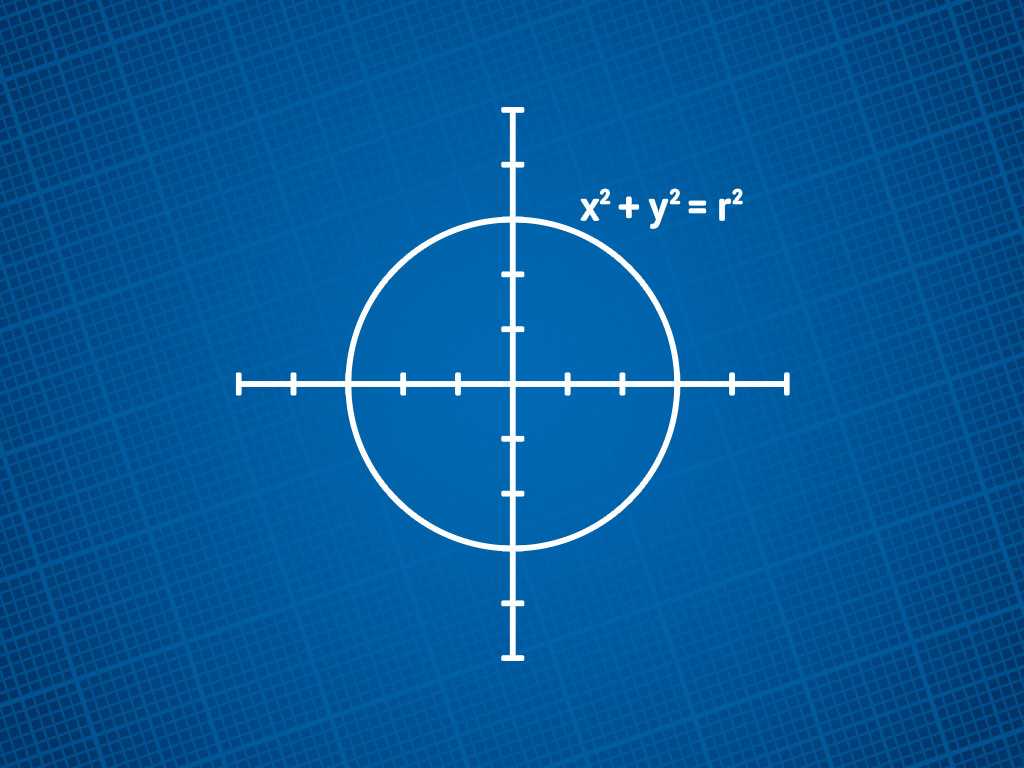
When the Origin is the Center

This is the easiest case when learning how to graph a circle. When a circle is at the origin of a cartesian plane, the variable part of the equation is eliminated. The value of ‘h’ and ‘k’ equal zero. The equation thus formed is:

(x – h)² + (y – k)² = r²

(x – 0)² + (y – 0)² = r²

x² + y² = r²

Let us assume a radius of 2 cm. Then the circle which shall be made will pass through (0, 2) and (0, -2) on the x-axis. On the y-axis, the circle will cover (2, 0) and (-2, 0). The circle’s center will remain (0,0) which is the origin. This is how you draw a graph when the circle’s center is the origin. See the figure below for a visual representation of the graph.

When the Center is Other than Origin

We can have multiple cases when the center of the circle is not the origin but a point at any quadrant of the cartesian plane. Let’s say, for example, we want to draw a graph at (3,8), which lies in the first quadrant of the cartesian plane. When we have such conditions, we use the standard formula 

(x – h)² + (y – k)² = r^2.

Here the point (h, k) acts as the circle’s centre point. The graph in this case can intersect the x-axis and y-axis at any point depending on the radius of the circle. 

Steps to draw a circle on a cartesian plane

Step 1: Locate the point (h,k) on the graph.

Step 2: Take your compass and adjust it according to the required radius.

Step 3: Place the tip of the compass on the point and draw a circle.

Step 4: The desired circle has been plotted. 

Let us understand this with the help of an example:

Example: Plot the graph of a circle having a radius of 4 cm from point B (6, -2). 

Solution: Let us solve this example using the steps mentioned above

Step 1: Mark the point (6, -2) on the cartesian plane. We find that the point lies in the 4th quadrant. Mark this point as A.

Step 2: Take your compass and set the radius as 4 cm.

Step 3: Place the compass on point A and draw a circle.

Hence the circle with a radius of 4 cm passing through point A (6, -2) has been formed.

The equation of this circle will be:

(x – 6)² + (y + 2)² = 4² 

Note when the values are negative the (x – h) or (y – k) changes to (x + h) or (y + k) respectively. This is because when we change the value from h to -h the equation becomes positive as 2 negatives when multiplied giving a positive result.

( x – (-h)) = (x + h).

Frequently Asked Questions

1. How do you solve a circle graph?

 To solve a circle graph, first look at the circle graph and see where the center of the circle is. Then count how many degrees are in each section of the circle graph. Then you can convert degrees to radians and multiply by pi to find out how many radians there are in each section.

2. How do you graph a circle on a calculator?

To graph, a circle on a calculator, first use the trace feature to draw a line from one point to another. Then use the zoom feature to zoom in on the center and trace around it.

3. How to draw a circle graph?

 First, draw a circle of the diameter of your paper. Then, draw lines from the center point of the circle to each corner of the paper. Next, place ticks on each line at regular intervals (10 cm). Finally, use your compass to make a mark at each tick point.

4. How do you graph the radius of a circle?

To graph the radius of a circle, you first need to draw a line from your origin (0,0) to the point (x,y) on the circle. Then, draw another line from your origin (0,0) to any point on that same circle. The distance between those two points will be equal to the radius (r).

5. How to find the center of a circle on a graph?

 To find the center of a circle on a graph, draw two perpendicular lines through opposite points of the circle. The intersection point will be the center of your circle.