Exponential functions are mathematical functions. These are widely used in many real-world situations, such as finding exponential decay or exponential growth. The exponential function decides whether an exponential curve will grow or decay. Here is all about the exponential function formula, graphs, and derivatives. Also, check out examples of exponential functions and important rules for solving problems.
Exponential Function Definition
An exponential function is a mathematical function that is commonly used in real-world applications. It is primarily used to compute investments, model populations, and so on. This article will teach you about the formulas, rules, properties, graphs, derivatives, exponential series, and examples.
What is An Exponential Function?
Exponential functions are mathematical functions in form f (x) = ax.. Here “x” is a variable, and “a” is a constant. The constant ‘a’ is the function’s base, and its value should be greater than 0.
The Natural Exponential Function
The most common exponential function base is the Euler’s number or transcendental number, e. The value of e is approximately equal to 2.71828.
f(x) = ex
Exponential Function Formula
If ‘a’ is any number such that a>0 and a≠1, then the exponential function formula is:
f(x) = ax
Where the variable x occurs as an exponent.
It is a real number.
If x is negative, the function is undefined for -1 < x < 1.
The following exponential function examples explain how the value of base ‘a’ affects the equation.
- If the base value a is one or zero, the exponential function would be:
f(x)=0x=0
f(x)=1x=1
Thus, these become constant functions and do not possess properties similar to general exponential functions.
- If the base value is negative, we get complex values on the function evaluation.
a = −4 the function would be, f(x) = (−4)x ⇒ f(1/2) = (−4)½ = √−4
So, we avoid 0, 1, and negative base values because we want only real numbers to arise from the evaluation of exponential functions.
Exponential Functions Examples
Some examples of exponential functions are:
- f(x) = 2x+3
- f(x) = 2x
- f(x) = 3e2x
- f(x) = (1/ 2)x = 2-x
- f(x) = 0.5x
Properties of an Exponential Function
A function’s exponential graph represents the exponential function properties.
The exponential function y = 2x.
The function y = 2x graph is shown below.
What is the Derivative of the Exponential Function
The derivative of exponential function f(x) = ax, where a > 0 is the product of exponential function ax and the natural log of a. This can be represented mathematically in terms of the integration of exponential functions as follows:
f'(x) = ax ln a
When we plot a graph of the derivatives of an exponential function, it changes direction when a > 1 and when a < 1.
Now we can also find the derivative of exponential function ex using the above formula. Where e is a natural number called Euler’s number. It is an important mathematical constant that equals 2.71828 (approx).
So, ex ln e = ex (as ln e = 1)
Hence the derivative of exponential function ex is the function itself, i.e., if f(x) = ex
Then f'(x) = ex
Exponential Function Graph
An exponential function graph helps in studying the properties of exponential functions. The following graph of exponents of x shows that as the exponent increases, the curve gets steeper. Also, the rate of growth increases.

Mathematically, this means that for x > 1, the value of y = fn(x). Thus, the value of y increases on increasing values of (n).
So, we can conclude that the polynomial function’s nature depends on its degree. On increasing the degree of any polynomial function, the growth increases.
When a> 1, y = f(x) = ax
Thus, for a positive integer n, the function f (x) grows faster than that of fn(x).
The exponential function with base > 1, i.e., a > 1 can be written as y = f(x) = ax. The set of entire real numbers will be the domain of the exponential function. Moreover, the range is the set of all the positive real numbers.
If ax = b and a > 1, the logarithm of b to the base is x. As a result, Loga b = x if ax = b. This is referred to as a logarithmic function.
Graphs of Exponential Functions Examples
The graph of an exponential function is an increasing or decreasing curve with a horizontal asymptote. The following graph of the basic exponential function y=ax will provide a clear understanding of the properties of exponential functions.
When a>1, the graph strictly increases as x. The graph will pass through (0,1) regardless of the value of a because a0 =1. We can note from this graph that the entire graph lies above the x-axis. This is because the range of y is all positive real numbers.

When 0 < a <1, the graph strictly decreases. Still, all the values will be above the x-axis. This is because the range of y=a^x is all positive real numbers.

From the above graphs, we can conclude the following:
- The graph passes through (0,1) irrespective of the base value.
- When a>1, the graph increases as x. Thus, it is concave up.
- When 0<a<1, the graph decreases as x. It is concave up.
- The graph lies above the x-axis.
- The x-axis is the horizontal asymptote for the graph.
Integration of Exponential Functions
The following formulas from integration help find the integral of the exponential function.
∫ ex dx = ex + C
∫ ax dx = ax / (ln a) + C
Rules of Exponential Functions
Some important exponential rules are given below. The following rules are applicable for all the real numbers x and y when a> 0 and b>0. These rules are vital for solving problems on exponential functions.
- Rule of Product
When the base is the same, the exponents will get added upon the multiplication of the bases. The example illustrates the rule.
ax ay = ax+y,
e.g., 52 x 53 = 52+3
⇒ 55 = 3125
- Rule of Quotient
When the base is the same number, the exponents will be subtracted from the division of the bases.
ax/ay = ax-y,
e.g., 54 x 52 = 54-2
⇒ 52 = 25
- Power rule
When power has an exponent, the base will be the same, and the exponents will multiply.
(ax)y = axy,
e.g. (52)3 = 52×3
⇒ 56 = 15,625
- Power of a Product
When two different bases have the same exponents as power, the bases will multiply, and the product will have the same power.
axbx=(ab)x
⇒ 22 32 = (2 x 3)2
⇒ 62 = 36
- Power of a fraction rule
When a fraction is raised to a power, both the denominator and numerator will have the same power/exponent.
(a/b)x= ax/bx
⇒ (6/2)2 = 62 /22
⇒ 36/4 = 9
- Zero exponents rule
Any number to the power zero is equal to 1.
a0=1
20 = 1
- Negative Exponent Rule
A number with a negative exponent can be written as 1 divided by the number which is raised to the exponent without the negative sign. So, the negative power turns positive in the denominator.
a-x= 1/ ax
5-2 = 1/52
⇒ 1/25
Applications of Exponential Functions
We use exponential functions in real-world applications to study various growth patterns and decline rates. Every quantity that decays or grows by a fixed percent at specific regular intervals possesses either exponential decay or exponential growth. Some common applications include plotting bacterial growth/decay, population growth and decline, and more.
Exponential Growth
Exponential Growth refers to an increase in quantity over time which is very slow at first and then increases rapidly. So, the rate of change increases over time. The rapid growth is an “exponential increase.” The adjacent exponential growth curve shows the exponential increase in population over time. The following formula defines exponential growth:
y = a ( 1+ r )x
where r is the growth percentage.

Exponential Decay
Exponential Decay is just the opposite of exponential growth. We widely use exponential growth and decay to study bacterial infections. Exponential decay refers to a decrease in quantity over time which is very rapid at first and then slows down. So, the rate of change decreases over time. The rapid decline is an “exponential decrease.” The following formula defines exponential decay:
y = a ( 1- r )x,
where r is the decay percentage.

Solved Problem
| Example 1: Simplify the following: (2p3)3 / 3 (p2)3 Solution: 23 p3×3 / 3p2×3 ⇒ 8 p9 / 3p6 ⇒ 8p9-6 /3 ⇒ 8p3 /3 |
Frequently Asked Questions?
1. How to Build an Exponential Function?
To build an exponential function, you need to know what the base is and then use the power property. The base is whatever number you’re going to multiply by itself over and over again to get your final answer. For example, if you want to build a function that represents 2^x where x is 5, then your base is 2 and your power is 5. If you have a function f(x) = x^2 + 11x + 24, then the base is 2 because it’s the number being multiplied by itself to get your final answer (the exponent on x).
2. How do you Solve an Exponential Function?
Solving an exponential function is pretty similar to solving linear functions.
To solve an exponential function, you need to isolate the variable on one side of the equation and then solve it, just like you would with a linear function.
For example, suppose we have the following equation:
2x^2 + 3x = 5.
We can solve this by subtracting 3x from both sides of the equation:
2x^2 + 3x – 3x = 5 – 3x
Now we can isolate x by dividing both sides by 2:
2(2)(3) + 6 = 5(3) + 6
And finally, we get our answer:
6 = 10
3. What are the Basic Concepts of Exponential Functions?
Exponential functions are a way of representing data that changes over time.
They are often used to describe the growth or decay of something over time, such as population growth or radioactive decay.
Exponential functions show how quickly something increases or decreases over time.
4. How do you Simplify an Exponential Equation?
You can simplify an exponential equation by factoring out the term that is raised to the power of one. For example, if you have the following equation:
y = 2x^3 + 4x^4 – 5x^2
You could factor out a common factor of 2 from both terms:
y = 2(2x^3 + 4x^4) – 5(2x^2)
5. How do you Solve an Exponential Equation Using a Log?
To solve an exponential equation using a log, first, rewrite the expression as a logarithm. Then, use the properties of logs to solve for the variable.
Example: Solve for x in equation 5x = 10^5.
First, write out the logarithmic form of the equation: log(5) = 10^5.
Now, use the properties of logs to solve for x: log(5)/log(10) = 5/x = 10^5/x = 5.
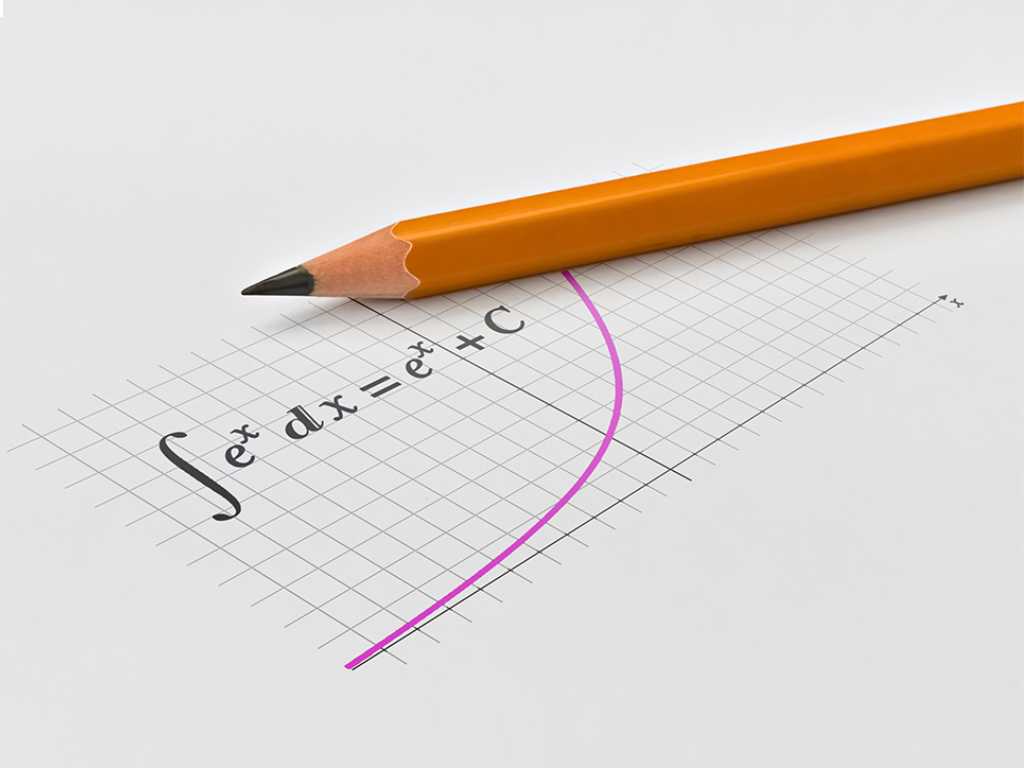
Related topics
Addition and Multiplication Using Counters & Bar-Diagrams
Introduction: We can find the solution to the word problem by solving it. Here, in this topic, we can use 3 methods to find the solution. 1. Add using counters 2. Use factors to get the product 3. Write equations to find the unknown. Addition Equation: 8+8+8 =? Multiplication equation: 3×8=? Example 1: Andrew has […]
Read More >>Dilation: Definitions, Characteristics, and Similarities
Understanding Dilation A dilation is a transformation that produces an image that is of the same shape and different sizes. Dilation that creates a larger image is called enlargement. Describing Dilation Dilation of Scale Factor 2 The following figure undergoes a dilation with a scale factor of 2 giving an image A’ (2, 4), B’ […]
Read More >>How to Write and Interpret Numerical Expressions?
Write numerical expressions What is the Meaning of Numerical Expression? A numerical expression is a combination of numbers and integers using basic operations such as addition, subtraction, multiplication, or division. The word PEMDAS stands for: P → Parentheses E → Exponents M → Multiplication D → Division A → Addition S → Subtraction Some examples […]
Read More >>System of Linear Inequalities and Equations
Introduction: Systems of Linear Inequalities: A system of linear inequalities is a set of two or more linear inequalities in the same variables. The following example illustrates this, y < x + 2…………..Inequality 1 y ≥ 2x − 1…………Inequality 2 Solution of a System of Linear Inequalities: A solution of a system of linear inequalities […]
Read More >>Other topics







Comments: