Doesn’t it get complicated when you try to solve a lengthy equation or expression and end up doomed while doing calculations? That’s when factoring expressions come in handy.
Factor expressions, also known as factoring, mean rewriting the expression as the product of factors. For example, 3x + 12y can be factored into a simple expression of 3 (x + 4y). In this way, the calculations become easier. The terms 3 and (x + 4y) are known as factors. Hence, an equation can have an end number of factors, depending on the length of the expression.
Factoring expressions is one of the most used mathematical operations while performing any calculation. Even if you wish to ease or quickly perform the regular, day-to-day calculations, you can use factoring expressions. For instance, multiplying 300 with 15 might sound tricky. The calculation gets swift if you do the multiplication of factors, such as multiplying 300 with 10 and 300 with 5 and then adding the terms. Hence, how do you factor an expression judges your problem-solving ability.
How to factor an expression?
Does your exam question ask you to factor in the given terms completely? Or is the question asking you to reduce the given term in simpler forms? Whatever be the question, the solution is only – factor expressions.
Below are a few methods that will help you learn how to factor an expression.
- Factoring numbers
The simplest way is to factor in the given numbers. For example, the number 10 can be factored into 5 and 2. You can include 1 because it is a factor as well. All-natural numbers will have 1 as the common factor whenever you are factoring expressions. However, negative numbers will also have 1 as the common factor, followed by -1 as one of the factors as well.
The natural numbers may have more than one factor. For instance, 100. It has factors – 5, 10, 20, 50, 25, 2, 4, and 1. So, to know which factors a number has, try dividing the number by the smallest factor, i.e., 2. Every even number will have a common factor – 2. If the number is odd, start dividing the number with the greater number after 2.
- Factoring the GCF from an expression
How can you simplify the mathematical terms to make the calculations easier? If your answer is by breaking or factoring them into smaller units, it’s correct. The greatest common factor, known as the GCF, is the highest factor that can be taken commonly in the given term. For example, in the term 34xy + 17y, 17y is the GCF. Hence, after taking out that term, the factors of the equation will become 17y (2x +1).
- Factoring binomials
Binomials are the terms that have degree 2. These can be factored in using various identities and methods.
The first method is by using identities. If you know basic algebraic identities, such as a2 + b2 + 2ab = (a + b)2, and similar ones, then you can make the factors of binomial equations.
If you are wondering how to factor an expression that does not match the identities, then the other method to find factors of binomial coefficients is to make them perfect squares. You have to add and subtract a common term so that it can be factored further using algebraic identities.
- Factoring trinomials
Factoring trinomials is the same as factoring binomial coefficients. Trinomial is an expression having degree 3. The first step to factor trinomial expressions is to take out the GCF. Then you can check whether it can be deduced into a binomial expression or not. If yes, then use the factoring binomial method. If not, then factor them using three-degree identities, such as a3 + b3 = (a + b) (a2 – ab + b2), and many others.
- Factoring equations by substitution
If the equational function is too complex, you can substitute the powered terms with a simpler term that can help you find the factors without much difficulty. For example, in the expression 9x4 + 45x2 + 14, you can substitute m = 3x2. This way the new equation will become, m2 + 15m + 14. And now, this can be easily factored in. But do not forget to replace the substituted term with the original term. You only have to change the term, and no further calculation or simplification is necessary.
Must-remember identities:
- a2 − b2 = (a + b) (a − b)
- a2 + 2ab + b2 = (a + b) (a + b)
- a2 − 2ab + b2 = (a − b) (a − b)
- a3 + b3 = (a + b) (a2 − ab + b2)
- a3 − b3 = (a − b) (a2 + ab + b2)
- a3 + 3 a2b + 3 ab2 + b3 = (a + b)3
- a3 − 3 a2b + 3 ab2 − b3 = (a − b)3
- Using the quadratic formula
The quadratic equation can be written in the form ax2 + bx + c. This equation represents a two-degree polynomial. To solve such equations and take out their factors, you can use the quadratic formula:
x = (-b ± √(b2 – 4ac))/2a
This quadratic formula will give you the roots for the quadratic expression. After finding the roots, you can equate them to zero to get the factors.
Let us understand this method with an example.
You have to find the factors of the quadratic equation 2x2 – 5x -12 = 0.
Let us use the quadratic formula to factor expressions.
x = (-b ± √(b2 – 4ac))/2a
Here, b = -5, a = 2, c = -12. Therefore,
x = (-(-5) ±√ ((-5)2 – 4 (2) (-12))/2 (2)
= 5 ± 25 – (-96)4 = 5 ± 114
= 16/4 or -6/4, which can be simplified as 4 or -3/2.
To find the factors of the following expression, equate the roots to zero. Hence, the factors will be (x – 4) (x + 3/2). The second factor can also be written as (2x + 3) when you will be equating the roots to zero; the denominator will also be equated to zero. Hence, there will be no difference between the previous term and the term with the denominator.
Therefore, the final factors of the expression 2x2 – 5x -12 = 0 are (x – 4) (2x + 3).
Importance of factoring expressions
In arithmetic, the greatest common factor and least common multiple plays a crucial role in simplifying a particular expression. These are used to solve questions based on percentage, ratio, and proportion and are involved in knowing the factors of the various numbers. Thus, knowing and learning factoring expressions is very useful in solving a problem.
In practical life, factorization makes computers and electronic devices safer. Subjects, such as cryptography use factorization techniques to make unhackable encryptions. Factorization is also used in forensics and other fields of medical sciences to solve mysterious cases. Thus, learn the fundamentals and concepts of factoring expressions right from the beginning.
Let us understand how to factor an expression from the examples given below.
Example 1: Factorize the expression x4 – 16.
Solution: The possible factorization for the number 4 in the term x4 could be 2. Hence, we can rewrite the expression as (x2)2 – 16.
What could be the factor of 16 in terms of multiplication? 4 x 4? Hence, the term becomes,
(x2)2 – 42. Since we know the identity, a2 – b2 = (a + b) (a – b), therefore, the factorization of the term will be (x2 + 4) (x2 − 4).
Further factoring the term with the minus sign, we get, (x2 + 4) (x + 2) (x − 2), which is the required factor of the given expression in the question.
Example 2: Factor the term 3y4 – 24yz3.
Solution: All you have to do is remove the common factor “3y” that can be seen in the given term: 3y4 − 24yz3 = 3y (y3 − 8z3).
After that, the difference of cubes will be: 3y4 − 24yz3 = 3y (y3 − (2z)3)
= 3y (y − 2z) (y2 + 2yz + 4z2), which is the factor form of the given equation.
Example 3: Write the factors of the given equations:
- 12x2 – 15xy
- x2 + x – 6
- 4a2 – 9b2
Solution: The factors of the following equations can be found by taking out the common terms. If you observe the expressions, you will see you can take out a number and a coefficient in common. Hence, using this methodology, the factors of the above-mentioned expressions can be written as:
- 3x (4x – 5y)
- (x + 3) (x – 2)
- (2a + 3b) (2a – 3b)
Example 4: Factorize the given equations:
- 36x2y2 – 15xy
- 8x3 – 32x5
- 14m2n5 – 28mn2 + 7m5n
- 5a(b + 3c) – 5m(b + 3c)
Solution: The factors for the given terms can be found by taking out the greater common factor. Hence, the factors will become:
- 3xy (12xy – 5)
- 8x3 (1 – 4x2)
- 7mn (2mn4 – 4n + m4)
- 5 (b + 3c) (a – m)
Problems for practice:
Question: For the given expressions below, find the factors.
- 9x2 y2 + 3xy
- x + x2 + x3
- 16x2y2 – 8x2y + 9y
- 2x2yz + 2xy2z + 4xyz
- 30xy – 12x + 10y– 4
- z – 19 + 19xy – xyz
- 100x2 – 80xy + 16y2
- 4x2 + 12x + 5
- 18x2 – 39x + 20
- 6x2 – 19x – 7
- 18x3 +3x2 – 6x
- x2 + 4x – 5
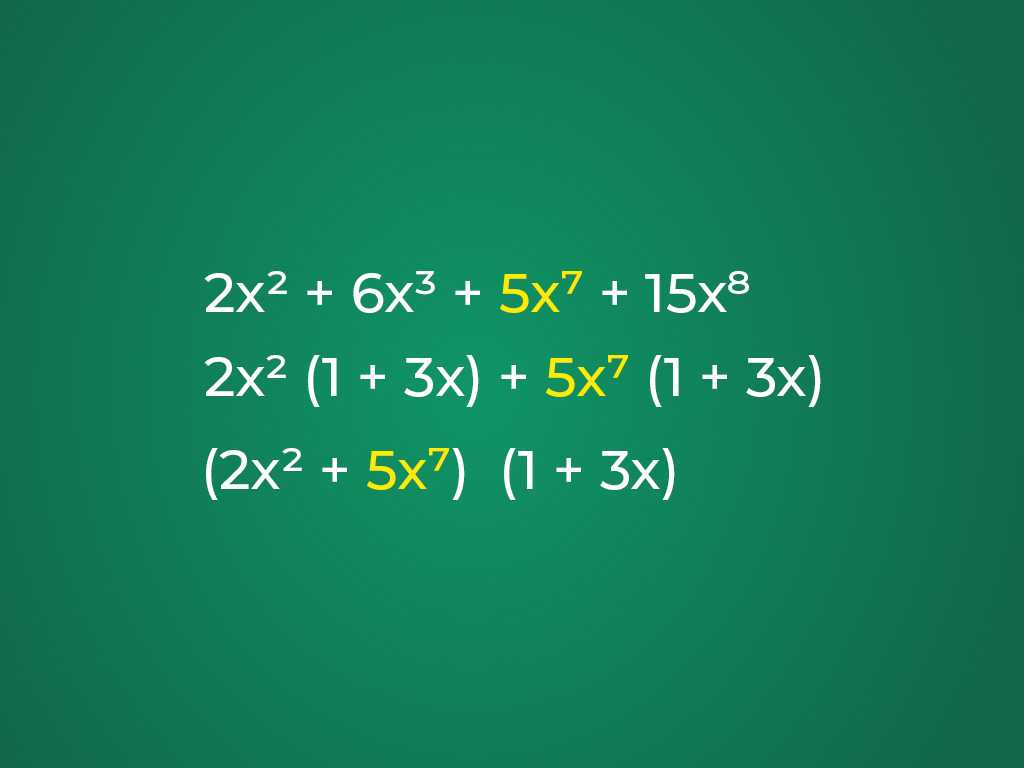
Related topics
Addition and Multiplication Using Counters & Bar-Diagrams
Introduction: We can find the solution to the word problem by solving it. Here, in this topic, we can use 3 methods to find the solution. 1. Add using counters 2. Use factors to get the product 3. Write equations to find the unknown. Addition Equation: 8+8+8 =? Multiplication equation: 3×8=? Example 1: Andrew has […]
Read More >>Dilation: Definitions, Characteristics, and Similarities
Understanding Dilation A dilation is a transformation that produces an image that is of the same shape and different sizes. Dilation that creates a larger image is called enlargement. Describing Dilation Dilation of Scale Factor 2 The following figure undergoes a dilation with a scale factor of 2 giving an image A’ (2, 4), B’ […]
Read More >>How to Write and Interpret Numerical Expressions?
Write numerical expressions What is the Meaning of Numerical Expression? A numerical expression is a combination of numbers and integers using basic operations such as addition, subtraction, multiplication, or division. The word PEMDAS stands for: P → Parentheses E → Exponents M → Multiplication D → Division A → Addition S → Subtraction Some examples […]
Read More >>System of Linear Inequalities and Equations
Introduction: Systems of Linear Inequalities: A system of linear inequalities is a set of two or more linear inequalities in the same variables. The following example illustrates this, y < x + 2…………..Inequality 1 y ≥ 2x − 1…………Inequality 2 Solution of a System of Linear Inequalities: A solution of a system of linear inequalities […]
Read More >>Other topics







Comments: