Key Concepts
- Expressing fractions in tenths as decimals.
- Expressing fractions in hundredths as decimals.
- Comparing and ordering decimals.
- Number patterns.
Introduction:
- In this chapter, we will learn to express fractions as equivalent fractions with a denominator 10.
- Simplifying fractions with a denominator of 10.
- Rounding numbers to the nearest ten.
- Knowing fractions and mixed numbers.
Fractions:
Fractions are represented as numerical values and can be defined as the parts of a whole.
Parts of Fraction:
All fractions consist of a numerator and a denominator.
- The denominator indicates how many parts the whole has been divided into. It is placed in the lower part of the fraction.
- The numerator indicates how many sections of the fraction are represented. It is placed in the upper part of the whole.
Example:

Example:

Understanding Tenths:
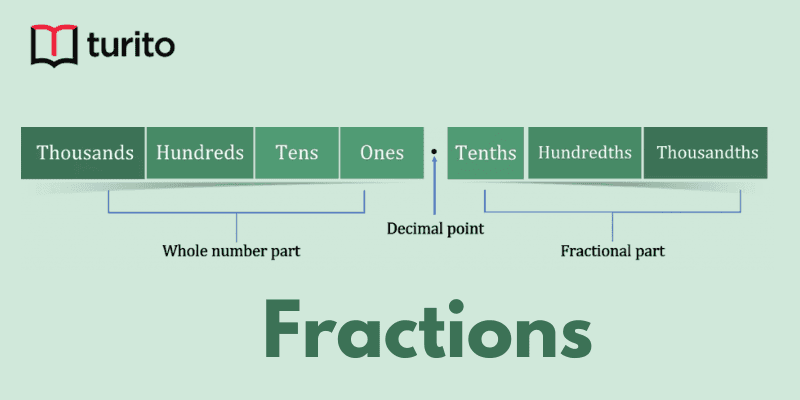
Decimals: A decimal number can be defined as a number whose whole number part and the decimal point separates the fractional part. The dot in a decimal number is called a decimal point.

Read and write tenths in decimal and fractional forms.
Tenths: The first digit to the right of the decimal point is one out of 10 equal parts of a whole.
Example:

Example: 0.6 means six-tenths or ![]()
Decimal form: A decimal is a fraction written in a particular form.
Example:

E.g., Instead of writing ![]()
You can express the fraction as 0.5.
Decimal point: A point used to separate the whole number part from the fractional part of the decimal number.
Example:

Example: 34.9
Here, 34 = Whole number part
9 = Fractional part
. = Decimal
Expanded form: The expanded notation, also called expanded form, for decimals is the same as the integer expanded form. A decimal can be written as the sum of all the place values.
E.g., (a) Write 317.29 in an expanded form.
Sol:

Place value chart:

Example: Write the place value of the digits 2 and 4 in the number 326.471
Sol:

Place value of 2 = Tens = 20
Place value of 4 = Tenths = 0.4
Understanding Hundredths:
Hundredths: Divide one whole into 100 equal parts or one-hundredth. In the decimal form, each part has a value equal to 0.01.
Example: Express the fraction in decimal?

Place holder zero: The zero is called a place holder. It is not worth anything on its own, but it changes the value of other digits.
Look at the number 502.

Sol: 52 = five tens and two units

The zero place-holder is keeping the 5 and 2 in their correct places:

Examples:
(a) Express as a decimal.
Sol: ![]() = 0.05
= 0.05
(b) Express 15 hundredths as decimal.
Sol: ![]() = 0.15
= 0.15
(c) Express 1![]() as decimal
as decimal
Sol:

1![]() = 1 one and 2 tenths 5 hundredths
= 1 one and 2 tenths 5 hundredths
= 1 one and 25 hundredths
= 1.25
Comparing Decimals:
Compare and order decimals.
Comparing Decimals: Comparing means examining the differences between numbers, quantities, or values to decide if it is greater than, smaller than, or equal to other quantities.
Example: Compare 4.27 and 4.65 using a number line.
Sol:

Example:
(a) Compare 0.6 and 0.8
Sol: 0.6 = 6 tenths
0.8 = 8 tenths
Because 8 tenths > 6 tenths
0.8 > 0.6
(b) Compare 0.317 and 0.341
Sol: 0.317 = 0.3 + 0.01 + 0.007
= 3 tenths + 1 hundredths + 7 thousandths
0.341 = 0.3 + 0.04 + 0.001
= 3 tenths + 4 hundredths + 1 thousandths
3 tenths = 3 tenths
Now, compare the next digit
1 hundredths < 4 hundredths
Thus, 0.317 < 0.341
Ordering: Arranging things in relation to each other according to a particular sequence or a pattern.
Example:
(a) Order 9.34, 83.9, 21.4, 0.96 from smallest to largest.
Sol: 0.96, 9.34, 21.4, 83.9
(b) Order 7.93, 5.94, 0.93, 28.7 from largest to smallest.
Sol: 28.7, 7.93, 5.94, 0.93
Complete number patterns
Number pattern is a pattern or sequence in a series of numbers by using a specific rule or pattern.
Example: (a) Complete the pattern 1.5, 1.9, 2.3, 2.7, 3.1, 3.5, 3.9, ____, ____
Sol:

(b) Find the next two numbers in the pattern 6.8, 6.4, 6, 5.6, 5.2, 4.8, 4.4.
Sol:

What have we learnt:
- Introduction of decimals.
- How the place value of a digit changes when we multiply or divide by ten.
- How to read, write, and model fractions with 10 and 100 in the denominator.
- How to compare decimals from the least to greatest and from the greatest to least.
- Ordering of decimals.
Related topics
Addition and Multiplication Using Counters & Bar-Diagrams
Introduction: We can find the solution to the word problem by solving it. Here, in this topic, we can use 3 methods to find the solution. 1. Add using counters 2. Use factors to get the product 3. Write equations to find the unknown. Addition Equation: 8+8+8 =? Multiplication equation: 3×8=? Example 1: Andrew has […]
Read More >>Dilation: Definitions, Characteristics, and Similarities
Understanding Dilation A dilation is a transformation that produces an image that is of the same shape and different sizes. Dilation that creates a larger image is called enlargement. Describing Dilation Dilation of Scale Factor 2 The following figure undergoes a dilation with a scale factor of 2 giving an image A’ (2, 4), B’ […]
Read More >>How to Write and Interpret Numerical Expressions?
Write numerical expressions What is the Meaning of Numerical Expression? A numerical expression is a combination of numbers and integers using basic operations such as addition, subtraction, multiplication, or division. The word PEMDAS stands for: P → Parentheses E → Exponents M → Multiplication D → Division A → Addition S → Subtraction Some examples […]
Read More >>System of Linear Inequalities and Equations
Introduction: Systems of Linear Inequalities: A system of linear inequalities is a set of two or more linear inequalities in the same variables. The following example illustrates this, y < x + 2…………..Inequality 1 y ≥ 2x − 1…………Inequality 2 Solution of a System of Linear Inequalities: A solution of a system of linear inequalities […]
Read More >>Other topics







Comments: