Intersecting Lines – Definition and Properties

An Intersecting line is straight and is considered to be a structure with negligible broadness or depth. Because of the indefinite length of a line, it has no ends. However, if it does have an endpoint, it is considered a line segment. One can identify it with the presence of two arrows, one at both ends of the line. Now that the concepts of a line and a line segment are clear, it will be easy to understand more about intersecting lines. 

Definition of Intersecting lines 

In a plane, intersecting lines are those that cross or meet. On the other hand, two or more lines are said to be non-intersecting if they never intersect. Let’s learn more about non-intersecting and intersecting lines in this article. 

Intersecting lines are formed when two or more lines cross each other in a plane. The intersecting lines share a common point, known as the point of intersection, which exists on all intersecting lines. 

Meaning of Intersection 

Intersection refers to a location or area where two or more things (such as streets) come together intersecting, the set of elements shared by two or more sets the collection of points that are shared by two geometric configurations. 

What are the two types of lines?

In geometrical terms, a line is a one-dimensional structure that is equal to the distance between two points. A line is usually straight and might not have an endpoint.

In case a line has a starting point and an endpoint, it is termed a line segment. It has a definite length and does not extend infinitely. 

On the other hand, a line is an unending figure that one cannot draw or perceive. Now, talking about line segments, the lines that have definite lengths, can be of two types –

• Non-intersecting lines

Non-intersecting lines refer to the lines that do not meet or touch each other. It means these lines do not intersect and are therefore known as non-intersecting lines.

A perfect example of non-intersecting lines can be two parallel lines next to each other. These parallel lines may extend forward or backward. However, they are always at a distance from each other, without a point of intersection.

• Intersecting lines

One can determine whether a given figure is of an intersecting line or not only if there is more than one line. It is not possible to show intersections with only one line.

Intersecting lines have a point of intersection. This point of intersection can be one or more than one depending upon the number of intersecting lines.

To know what intersecting lines are, read the sections below to understand.

What are intersecting lines?

In theory, a line is defined as a figure that only has one dimension. Apart from this, lines have an infinite length which is generally immeasurable. However, to portray a line, a line segment is used. Even though a line segment looks like a line, it has two endpoints. It signifies that a line segment can be measured and has an endpoint. 

Coming on what are intersecting lines? Two given line segments are said to be intersecting lines when they have a point of intersection. 

A point of intersection is referred to the point where two or more line segments meet. These lines are not parallel to each other and hence intersect when drawn forward. It is seen that when two non-parallel line segments are drawn finitely, they cross each other at some point. This single point is where the intersection takes place.

What are the properties of intersecting lines?

To understand more about intersecting lines, one can start by knowing the geometric properties of intersecting lines.

Following are the properties of intersecting lines:

• Two or more line segments are needed to form a point of intersection.
• It is possible that the intersection between two or more line segments only has one point of intersection.
• The line segments should at least have one point where they cross the path of each other. This particular point is the point of intersection.
• The point of intersection is a common point among all the line segments that intersect.
• Two Intersecting line segments can only have one point of intersection.
• No matter what the angle is, two straight lines cannot meet at more than one point.
• Two intersecting lines cannot be parallel to each other.
• The line segments that meet at more than one point do not usually have a straight path.
• Two lines can only have more than one point of intersection when either of the lines is curved.
• The point of intersection usually forms an angle between the two intersecting lines.
• Two lines intersect at a point where the angle is between 0°-180°.

How to find the intersection of two lines?

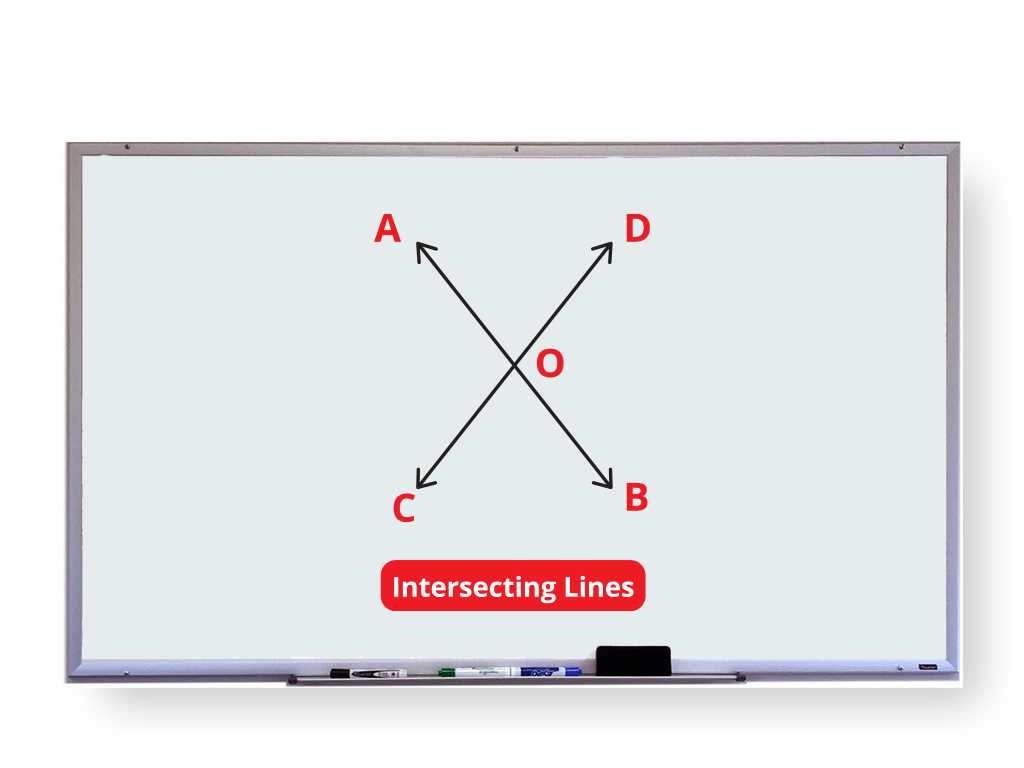
To understand how to find the intersection of two lines, one has to take a look at two non-parallel lines. Given below is an image of two non-parallel lines that intersect at a given point.

One can easily distinguish the intersecting point of two lines just by looking at them. When two line segments cross paths with each other, they are considered to be two intersecting lines. It helps in understanding how to find the intersection of two lines.

The intersection of two lines depends upon the following factors:

• The lines should be non-parallel to each other.
• The lines at the given point of intersection should be straight.
• The point of intersection should make two lines intersect at a point with an angle between the two intersecting line segments.
• If two non-parallel lines do not have a point of intersection, it does not mean they are non-intersecting. It means when these two line segments are drawn forward, they will meet at a certain point, even if it is a long distance.

What does the intersection of two lines look like?

It is quite simple to identify whether the given figure of two line segments intersect or not. Two intersecting lines might resemble the letter T or V. In most cases, two intersecting lines form an X when they cross each other.

This depiction signifies that two intersecting lines meet at a single point only. By understanding the above image, it is an effortless task to identify intersecting lines the next time you see it.

It is possible for two non-parallel line segments to not cross each other. However, they can still have a point of intersection like the letter V.

Two intersecting lines look similar to the letter T when they are perpendicular to each other. When two lines are set to be perpendicular to each other, it means they form a 90° angle at the point of intersection. It means perpendicular lines are also intersecting lines that only form right angles.

How do identify whether two lines will intersect each other or not?

From the above explanations, it is clear that parallel lines do not intersect each other. However, even the slightest tilt of either of the lines can result in an intersection.

Two given line segments might seem parallel, but they still can have a point of intersection when drawn forward or backward.

In some cases, even if there are two non-parallel line segments, they are considered to be non-intersecting lines because they have a large distance between them. It is irrelevant to say two lines cannot intersect each other just because they are far from each other. These lines might take a long distance to cross the path of each other. However, they cannot be called non-intersecting lines.

Two lines will intersect each other if –

• They are non-parallel to each other.
• They are perpendicular to each other.

How can two lines not intersect each other?

Certain criteria determine the intersection of two lines. If two lines do not satisfy these criteria, they are not regarded as intersecting lines. Following are the causes that determine when two given line segments do not cross each other.

Two lines cannot intersect each other when drawn forward if they have:

• Endpoints
• An infinite distance between them
• They are parallel to each other
• They are non-parallel but have definite lengths and do not cross each other
• Are non-parallel line segments with a space between them

What are some real-life examples of intersecting lines?

The intersection of lines is a common example of everyday life. Even though one might not focus on them, they are surrounded by lines of intersection.

For example, a crossroad is a location where two roads meet at a common point. This example signifies that the two roads are non-parallel to each other. Apart from this, these two roads form a particular angle at their intersection point.

Another example is a pair of scissors. The two knives of scissors are like the two intersecting lines. When held apart, the two knives form an angle when two lines intersect at a point of intersection.

Examples of intersecting lines 

Example: Give real-world examples of non-intersecting and intersecting lines. 

The following are two examples of intersecting lines: 

1. Crossroads are formed when two straight roads intersect at a common point. 
2. Scissors: A pair of scissors has two arms that form intersecting lines. 
3. Clock: Intersecting lines are formed by two needles passing through a common point in the clock. 
4. A, T, L, M: Some letters in the English alphabet are also examples of intersecting lines. 
5. Notebook Edges: The adjacent edges of a notebook intersect at a right angle. As a result, it is a case of intersecting lines. 

Here are two examples of non-intersecting lines: 

1. Scale: A ruler’s opposite sides are non-intersecting lines. 
2. Rails of a Railway Track: Rails of a railway track that are parallel to each other are non-intersecting lines. 
3. Cricket wickets are arranged in such a way that they never intersect; thus, they are an example of a non-intersecting line. 
4. Electric Tower Wires: Because the wires in electric towers are all the same length, they are a perfect example of non-intersecting lines. 
5. A ladder is an example of a non-intersecting line because its two sides never intersect. 

Conclusion

Intersecting lines are a convenient way to study lines and their point of intersection. The above details thoroughly explain the properties and requirements of two intersecting lines. Apart from this, the point of intersection allows one to understand the angle formation and how right angles are formed between two perpendicular lines. Various areas of science deal with the intersection and angle formation between two non-parallel lines.