A numerical expression comprises two words, ‘Numerical’, which means related to numbers, and ‘Expression’, which indicates the operations on the numbers. In this article, we shall thoroughly discuss what is meant by the term numerical and how to simplify the numerical expression. Numerical Expressions are among the most basic yet most important mathematical concepts. Every pupil should have a firm grasp on solving numerical expressions. This article also entails a lot of examples and solved problems based on numerical expressions for students to understand them clearly. We usually look at a numerical face when we look at a problem with numbers. Without further ado, let’s jump straight to the topic.
What Do You Understand by a Numerical Expression?
Definition of a Numerical Expression: The term numerical refers to something that involves numbers. We know that expression is nothing but just a phrase. Thus, numerical expression is a phrase involving numbers. In mathematics, a numerical expression is a set of numbers that have been written together by utilizing arithmetic operators addition, subtraction, multiplication, and division.
There are different forms in which the number can be expressed, such as word form and numerical form. A numerical expression is a mathematical statement involving only numbers and one or more operation symbols. Thus if someone gives you an expression, for example, x + 2 – 3 + 8 = 11, and says that it is a numerical expression, you have to deny that. This is not a numerical expression as it has a variable ‘x’ present. Numerical expressions are made of pure numbers. No variables or words are present in them. Students often get confused in differentiating between a numerical expression and a non-numerical expression.
Numerical Expression Example
A numerical expression is formed by combining numbers, including various mathematical operators. There is no limit to the number of operators that a numerical expression may contain. Some numerical expressions use only one operator between two numbers, whereas some may have more than one.
See the table below:
| Numerical Expression | Non-Numerical Expression |
| Consists of only numbers or integers. | Contains numbers as well as variables such as x, y, a, b, k, l, etc. They are therefore termed algebraic expressions. |
| For example: 10 + 23, 34 x 12, etc | For example: 3x – 2y + 11 – 22 = 0, 2k = 22, etc. |
Remember that the only requirement for a numerical expression is that it contains numbers and arithmetic operators in between them. There can be one or more operators in a numerical expression. For instance,
2 + 17 is a numerical expression with one operator that is addition.
11 + 43 x ( -4) is a numerical expression with three operators addition followed by multiplication and subtraction.
Now, the question arises can squares, square roots, cubes, cube roots, and other exponential numbers be represented as numerical expressions? Let us find out.
For instance, let’s say someone gives us 172 and asks us whether this is a numerical expression or not? You should reply with, ‘yes, this is a numerical expression’. When we look carefully, 172 can be split into two parts and re-written as 17 x 17 – 117. Hence, we get a phrase with two pure numbers with the multiplication operator between them, making 172 a numerical expression.
Similarly, 63 = 6 x 10 + 3.
Also √49 = 7 x 7. Therefore we can say that a power, consisting of a base and exponential, is a numerical expression.
So far, we must be clear about the definition of a numerical expression and how we can identify them. Let us now look at some examples of numerical expressions:
Numerical expressions having single arithmetic operators:
21 + 13 ( for addition )
78 – 43 ( for subtraction)
6 x 32 ( for multiplication )
22 ÷ 2 ( for division )
Numerical expressions having two or more arithmetic operators
63 x 192 – 32
4 x 9 + 93 / 3
45 / 5 – 2 x 4 + 33
How to Write a Numerical Expression from Word Problems in Math
Any word problem in Math is solved by first converting it into a numerical expression. In the word problems, try to visualize what the question is saying. Note down all the numbers present in the problem and one-by-one assign operators between them to form a numerical expression. Look at the examples below to understand them in a better way:
Example 1: Samyak is a farmer with 20 watermelons from his farm to give to his neighbors. He gives five watermelons to Rudransh and six to Mohit in the morning. In the evening, Gaurav, another farmer in that village, gifts four watermelons to Samyak. At the end of the day, how many watermelons does Samyak have?
Solution: Let us read the statements one by one and note down all the numbers we can find.
At first, Samyak had 20 watermelons. He gave 11 watermelons in the morning (5 to Rudransh and 6 to Mohit). In the evening, Gaurav gave 4 watermelons to Samyak.
Therefore the numerical expression for this problem will be:
= 20 – 5 – 6 + 4
= 20 – 11 + 4
= 24-11
= 13
Thus Samyak has 13 watermelons left with him at the end of the day.
Example 2: 100 students are divided into 5 major races on a school’s sports day. After the first round of each race, ten students were eliminated. After the second round, 7 more students were eliminated from each race. Find the number of students present for each race in the final round.
Solution: Let us read the statements one by one and note down all the numbers we can find.
The total number of students in the school’s main event is 100. They are divided into 5 major races. After 1 round, ten students are removed from each race. After the second race, 7 more students are removed. This can be expressed in numerical form as:
= 100 / 5 – 10 – 7
= 20 – 10 – 7
= 20 – 17
= 3
Therefore with the help of numerical expression, we find that three students make it to the final round of each race.
Simplification of Numerical Expressions
The BODMAS and the PEMDAS rule simplify a numerical expression with two or more operations. These rules state that this pattern should be followed to solve any numerical expression.
The priority is given to operations such as brackets followed by order of powers or roots. The arithmetic operators are divided first, multiplication second, addition third, and subtraction fourth. You can switch the places of addition and subtraction according to your choice.
The word BODMAS stands for:
B → Brackets ( Parentheses (), Curly brackets {}, or square brackets [] )
O → Order of powers or roots (also the value of trigonometric and logarithmic functions)
D → Division
M → Multiplication
A → Addition
S → Subtraction
And PEMDAS stands for:
P → Parenthesis
E → Exponents
M → Multiplication
D → Division
A → Addition
S → Subtraction
This is the exact way we must approach the solutions to any numerical problem.
Hitherto, we have learned everything we need to know about numerical expressions. After reading this article, students must be confident in numerical expressions and related topics like its definition, how to write a numerical expression, the difference between numerical and algebraic expressions, and how to solve a numerical expression. Let us look at some more examples and problems related to this chapter below. We recommend you solve them by yourself first. If you are stuck somewhere, you can always visit this article to look for stepwise solutions to each problem.
Practice Questions
Simplify the Following Numerical Expressions
- Solve 20 + 3 − (44 ÷ 4) × 6
Using the BODMAS rule:
First, we shall solve the brackets
20 + 3 − (11) × 6
Now we multiply the terms 11 and 6
20 + 3 – 66
Now we need to perform an addition
= 23 – 66
= – 43
Thus the answer is – 43.
- Find the Value of [(41 + 8) − 11 + ( 27 ÷ 9 )] x 4
Using the BODMAS rule:
First, we shall solve the parentheses (41 + 8) and (27 ÷ 9)
[ 49 – 11 + 3 ] x 4
Now we solve the expression inside square brackets
[ 52 – 11 ] x 4
[ 41] x 4
Finally, now we will multiply the remaining terms
41 x 4 = 164
Thus the answer is 164.
- Evaluate the Numerical Expression (10² x 3 – 2) + 9 x 11
According to PEMDAS:
First, you need to solve the parenthesis (10² x 3 – 2)
In the parentheses, you will first solve the order, i.e., the number with the power
(100 x 3 – 2)
Now multiplication within parenthesis followed by subtraction
= 300 – 2
= 298
Now the expression becomes 298 + 9 x 11
To solve this, first, multiply, then add
298 + 99
= 397
Thus the value after simplification of the numerical expression is 397.
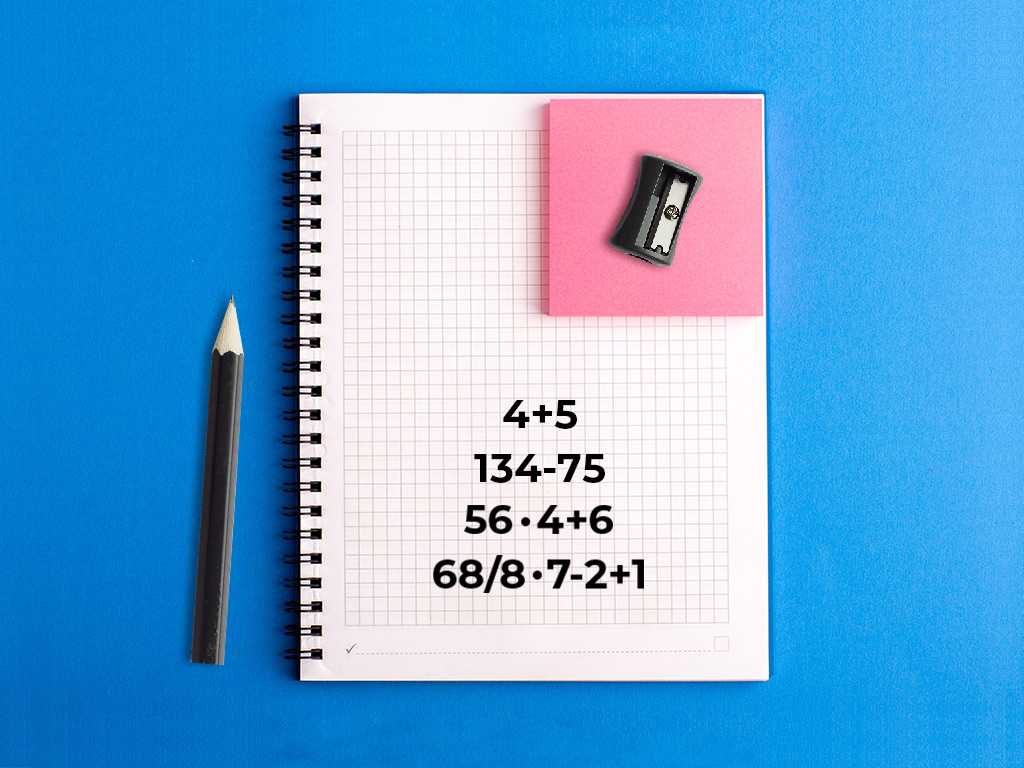
Related topics
Addition and Multiplication Using Counters & Bar-Diagrams
Introduction: We can find the solution to the word problem by solving it. Here, in this topic, we can use 3 methods to find the solution. 1. Add using counters 2. Use factors to get the product 3. Write equations to find the unknown. Addition Equation: 8+8+8 =? Multiplication equation: 3×8=? Example 1: Andrew has […]
Read More >>Dilation: Definitions, Characteristics, and Similarities
Understanding Dilation A dilation is a transformation that produces an image that is of the same shape and different sizes. Dilation that creates a larger image is called enlargement. Describing Dilation Dilation of Scale Factor 2 The following figure undergoes a dilation with a scale factor of 2 giving an image A’ (2, 4), B’ […]
Read More >>How to Write and Interpret Numerical Expressions?
Write numerical expressions What is the Meaning of Numerical Expression? A numerical expression is a combination of numbers and integers using basic operations such as addition, subtraction, multiplication, or division. The word PEMDAS stands for: P → Parentheses E → Exponents M → Multiplication D → Division A → Addition S → Subtraction Some examples […]
Read More >>System of Linear Inequalities and Equations
Introduction: Systems of Linear Inequalities: A system of linear inequalities is a set of two or more linear inequalities in the same variables. The following example illustrates this, y < x + 2…………..Inequality 1 y ≥ 2x − 1…………Inequality 2 Solution of a System of Linear Inequalities: A solution of a system of linear inequalities […]
Read More >>Other topics







Comments: