Parallel and Perpendicular Lines With Word Problems

Parallel and Perpendicular Lines

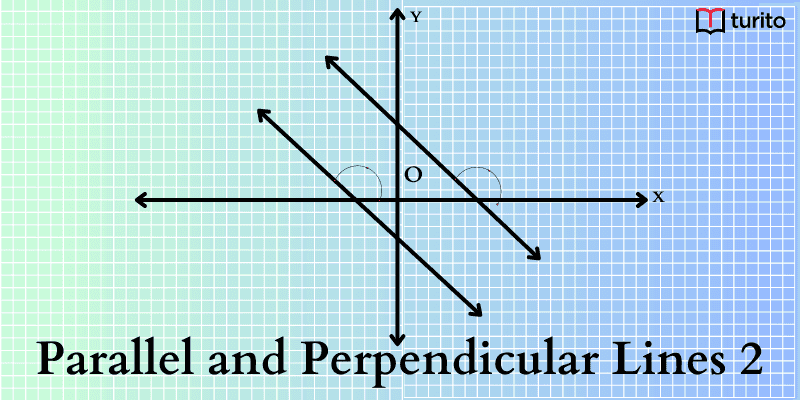
Parallel Lines:

Parallel lines are lines in a plane that are always the same distance apart. Parallel lines never intersect.

Real-life Example:

Perpendicular Lines:

Perpendicular lines are lines that intersect at a right (90-degree) angle.

 

Real-life Example:

Classify Lines

Example1:   

Are the graph of the equations y= -1/7 x-5 and y=-1/7 x-1 parallel, perpendicular, or neither?

Sol:

Step1:

Identify the slope of each line.

 y= -1/7 x-5                   y=-1/7 x-1

m₁ = -1/7                      m₂ = -1/7                                 

Step2:

Compare the slope of the lines.

The slopes of the lines are -1/7 and –1/7.          

The slope is the same.

The graph of the equations and y= -1/7 x-5 and y=-1/7 x-1 are parallel.

Example2:   

Is the graph of the equations x+2y=7 and -2x+y=3 parallel, perpendicular, or neither?

Step1:

Identify the slope of each line.

First line:                                                     

x+2y=7         

Divided both sides by 2.

y= -1/2 x 

m=-1/2                                                           

Second line:           

  y =2x +3     

  m =2

The slopes of the lines are – 1/2 and 2.

Step2:

Compare the slope of the lines.

The slopes of the lines – 1/2  and 2 are opposite and reciprocals

perpendicular.

Solve Real Word Problems

Example:

Architecture, An architect uses software to design the ceiling of a room. The architect needs to enter an equation that represents a new beam. The new beam will be perpendicular to the existing beam, which is represented by the red line. The new beam will pass through the corner represented by the blue point. What is the equation that represents the new beam?

Sol:

Step1:

Use the slope formula to find the slope of the red line that represents the existing beam.

m =4-6/6-3

= = – 2/3

The slope of the line that represents the existing beam is = – 2/3.

Step2:

Find the opposite reciprocal of the slope from step 1.

The opposite reciprocal is -2/3  is 3/2

Step3:

Use the point-slope form to write an equation. The slope of the line that represents the new beam is 3/2.

It will pass through (12, 10)

An equation that represents the new beam is

y-10 = 3/2x-12

Exercise

1. Are the graphs of the equations parallel, perpendicular, or neither?

a. x-3y =6 and x-3y =9

b. y=4x+1 and y=-4x -2

2. How many types of lines are there?

3. Determine that the lines are parallel, perpendicular, or neither.

2x-3y =9 and 6y-4x=1

4. Identify the below shape as parallel, perpendicular, or neither.

5. Identify the lines as parallel or perpendicular.

     2x+5y =7

     4x+10y =18

6. How do you identify parallel lines and perpendicular lines?

7. Do parallel lines meet?

8. What are some examples of perpendicular lines in real life?

9. When put on a coordinate plane, Main Street has the equation y = – 1/3 x-4 Park Street is perpendicular to Main Street and goes through the point (3, -5). Write the equation for Park Street in slope-intercept form.

10. You and your friend have gift cards to a shopping mall. Your card has $20 on it, and your friend’s card has $30 on it. For every month that you don’t use your card, its value decreases by $2. Write two equations modeling your and your friend’s gift cards, then determine if the lines are parallel or perpendicular.

       Concept Map:

What We Have Learned:

• Understand parallel and perpendicular lines
• Classify lines
• Solve real word problems
• Identify parallel and perpendicular lines in real life.