Blog Post

Grade 1

Grade 2

Grade 3

Grade 4

Grade 5

Grade 6

Grade 7

Grade 8

Grade 9

Grade 10

Grade 11

Grade 12


<h4 class="wp-block-heading">Piecewise Function</h4>



<h4 class="wp-block-heading">1. Understand Piecewise-Defined Functions&nbsp;</h4>



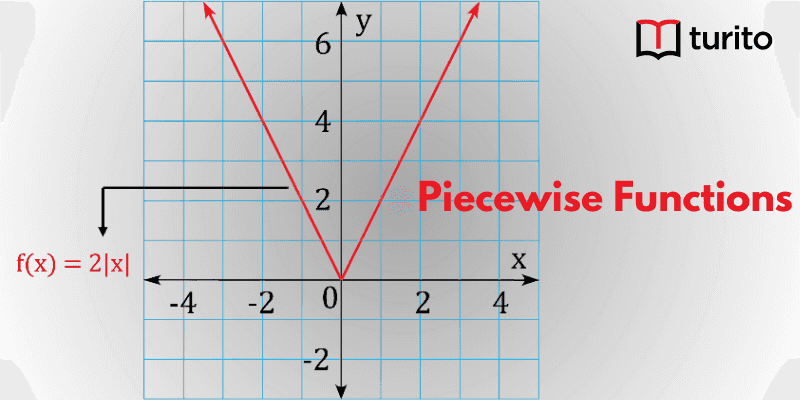
<p><strong>How is f(x) = 2|x| related to a linear function?&nbsp;</strong>&nbsp;</p>



<p>Inspect the <a href="https://www.turito.com/ask-a-doubt/Maths-the-graph-of-the-function-f-defined-by-f-x-1-4-x-4-2-5-is-shown-the-graph-passes-through-the-points-10-a-and-q35d3b6" data-type="link" data-id="https://www.turito.com/ask-a-doubt/Maths-the-graph-of-the-function-f-defined-by-f-x-1-4-x-4-2-5-is-shown-the-graph-passes-through-the-points-10-a-and-q35d3b6">graph of the function</a> f(x) = 2|x|. The graph has two pieces that meet at the vertex. Each piece is part of a line.  </p>



<p>Find the rule for each piece of the function.&nbsp;&nbsp;</p>



<p>When x ≥0, the rule is f(x) = 2x.&nbsp;&nbsp;</p>



<p>When x &lt; 0, the rule is f(x) = -2x.   </p><div class="fillWidthInMob"><a loading='lazy' class="showAdInAllRes"  data-toggle="modal" data-target=#homeLeadForm data-heading="Get Expert Guidance" href="#"><img class="img-fluid" alt="parallel" src=https://a.storyblok.com/f/128066/1100x230/00b28b4876/11-applying-for-collages_leaderboard-1100x230.jpg /></a></div>


<div class="wp-block-image">
<figure class="aligncenter size-full is-resized"><img loading="lazy" decoding="async" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/linear-function.webp" alt="Linear Function" class="wp-image-48222" style="width:462px;height:348px" width="462" height="348" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/linear-function.webp 575w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/linear-function-300x226.webp 300w" sizes="auto, (max-width: 462px) 100vw, 462px" /></figure></div>


<p>You can write this <a href="https://www.turito.com/learn/math/step-function-grade-10" data-type="link" data-id="https://www.turito.com/learn/math/step-function-grade-10">function</a> in terms of its pieces, each defined for a given domain.  </p>


<div class="wp-block-image">
<figure class="aligncenter size-full"><img loading="lazy" decoding="async" width="447" height="217" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/function-in-terms.webp" alt="Function in terms" class="wp-image-48223" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/function-in-terms.webp 447w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/function-in-terms-300x146.webp 300w" sizes="auto, (max-width: 447px) 100vw, 447px" /></figure></div>

<div class="wp-block-image">
<figure class="aligncenter size-full is-resized"><img loading="lazy" decoding="async" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/piecewise-defined-function.webp" alt="piecewise-defined function" class="wp-image-48224" style="width:450px;height:341px" width="450" height="341" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/piecewise-defined-function.webp 686w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/piecewise-defined-function-300x227.webp 300w" sizes="auto, (max-width: 450px) 100vw, 450px" /></figure></div>


<p>The function is a <em>piecewise-defined</em> function. A <strong>piecewise-defined function </strong>has different rules for different intervals of its domain.  </p>



<p>The function f(x) = 2|x| has two pieces over two intervals of the domain. For each interval, the piece is a linear function.&nbsp;&nbsp;</p>



<h4 class="wp-block-heading">2. Graph Piecewise-Defined Function&nbsp;</h4>



<ol class="wp-block-list">
<li><strong>What is the graph of:</strong>&nbsp;</li>
</ol>


<div class="wp-block-image">
<figure class="aligncenter size-full"><img loading="lazy" decoding="async" width="349" height="148" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Graph-Piecewise.webp" alt="Graph Piecewise" class="wp-image-48225" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Graph-Piecewise.webp 349w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Graph-Piecewise-300x127.webp 300w" sizes="auto, (max-width: 349px) 100vw, 349px" /></figure></div>


<p>Graph each piece of the function for the given domain.  </p>


<div class="wp-block-image">
<figure class="aligncenter size-full is-resized"><img loading="lazy" decoding="async" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/piece-of-the-function.webp" alt="Piece of the function" class="wp-image-48226" style="width:426px;height:338px" width="426" height="338" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/piece-of-the-function.webp 656w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/piece-of-the-function-300x238.webp 300w" sizes="auto, (max-width: 426px) 100vw, 426px" /></figure></div>


<ol class="wp-block-list" start="2">
<li><strong>Over what part of the domain is the function increasing? Decreasing?</strong>&nbsp;</li>
</ol>



<p>The function<em> f </em>increases when x ≤ 2 and decreasing when x > 2.  </p><div class="fillWidthInMob"><a loading='lazy' class="showAdInAllRes"  data-toggle="modal" data-target=#homeLeadForm data-heading="Get Expert Guidance" href="#"><img class="img-fluid" alt="parallel" src=https://a.storyblok.com/f/128066/1100x230/00b28b4876/11-applying-for-collages_leaderboard-1100x230.jpg /></a></div>



<h4 class="wp-block-heading">3. Analyse the Graph of a Piecewise-Defined Function&nbsp;</h4>



<p><strong>Cheyenne’s mother is reviewing the monthly water bills from the summer. Each monthly bill includes a graph like the one shown, which reflects the different rates charged for water based on usage.&nbsp;</strong>&nbsp;</p>



<p><strong>Several relatives visited Cheyenne’s family in July and their water bill is more than doubled. Assuming that the water consumption did not double that month, what is the possible explanation for the increase? </strong> </p>


<div class="wp-block-image">
<figure class="aligncenter size-full is-resized"><img loading="lazy" decoding="async" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Gallons-of-Water.webp" alt="Gallons of Water" class="wp-image-48227" style="width:319px;height:319px" width="319" height="319" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Gallons-of-Water.webp 520w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Gallons-of-Water-300x300.webp 300w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Gallons-of-Water-150x150.webp 150w" sizes="auto, (max-width: 319px) 100vw, 319px" /></figure></div>


<p>The graph shows three tiers of pricing for water consumption: from 0 to 5000 gal, from 5001 to 10,000 gal, and more than 10,000 gal.&nbsp;&nbsp;</p>



<p>The large increase in bill probably resulted from the usage increasing across one boundary of the domain, from Tier 1 to Tier 2, or Tier 2 to Tier 3.&nbsp;&nbsp;</p>



<p>At the Tier 1- Tier 2 boundary 5,000 gal of usage results in a bill of $5 while using slightly more water results in a bill of at least $10.&nbsp;&nbsp;</p>



<h4 class="wp-block-heading">4. Apply a Piecewise-Defined Function&nbsp;&nbsp;</h4>



<p><strong>A gym owner wants to purchase custom wristbands for marketing promotion. She thinks she will need about 75 bands. Her assistant insists that ordering over 100 wristbands will be less expensive than ordering 75. How can the assistant convince the gym owner? </strong> </p>


<div class="wp-block-image">
<figure class="aligncenter size-full is-resized"><img loading="lazy" decoding="async" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/custom-wristbands.webp" alt=" custom wristbands" class="wp-image-48228" style="width:445px;height:273px" width="445" height="273"/></figure></div>


<p><strong>Formulate:&nbsp;</strong>&nbsp;</p>



<p>Write a rule to represent each price point.&nbsp;&nbsp;</p>



<p>Let x = number of wristbands&nbsp;</p>



<p>Let f(x) = total cost  </p>



<figure class="wp-block-image size-full"><img loading="lazy" decoding="async" width="768" height="163" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Formulate.webp" alt="Formulate" class="wp-image-48229" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Formulate.webp 768w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Formulate-300x64.webp 300w" sizes="auto, (max-width: 768px) 100vw, 768px" /></figure>



<p>Write a piecewise-defined function to represent the situation.  </p>


<div class="wp-block-image">
<figure class="aligncenter size-full"><img loading="lazy" decoding="async" width="474" height="114" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/piecewise-defined-function-1.webp" alt="piecewise-defined function" class="wp-image-48230" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/piecewise-defined-function-1.webp 474w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/piecewise-defined-function-1-300x72.webp 300w" sizes="auto, (max-width: 474px) 100vw, 474px" /></figure></div>


<p><strong>Compute:&nbsp;</strong>&nbsp;</p>



<p>Evaluate the function for f(75) and f(101).&nbsp;&nbsp;</p>



<p>f(75) = 75 + 10 = 85&nbsp;&nbsp;</p>



<p>f(101) = 0.5(101) = 50.5&nbsp;&nbsp;</p>



<p>The cost for 75 wristbands is $85 and the cost for 101 wristbands is $50.5.  </p>


<div class="wp-block-image">
<figure class="aligncenter size-full is-resized"><img loading="lazy" decoding="async" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Compute.webp" alt="Compute" class="wp-image-48231" style="width:347px;height:338px" width="347" height="338" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Compute.webp 364w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Compute-300x293.webp 300w" sizes="auto, (max-width: 347px) 100vw, 347px" /></figure></div>


<p><strong>Interpret:&nbsp;</strong>&nbsp;</p>



<p>The gym owner will spend less if she orders more than 100 wristbands.&nbsp;&nbsp;</p>



<h4 class="wp-block-heading">Questions&nbsp;&nbsp;</h4>



<p><strong>Question 1</strong>&nbsp;</p>



<p><strong>Express f(x) = -3|x| as a piecewise-defined function.&nbsp;&nbsp;</strong>&nbsp;</p>



<p><strong>Solution:</strong> </p>


<div class="wp-block-image">
<figure class="aligncenter size-full"><img loading="lazy" decoding="async" width="289" height="414" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Question-1.webp" alt="Question 1" class="wp-image-48232" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Question-1.webp 289w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Question-1-209x300.webp 209w" sizes="auto, (max-width: 289px) 100vw, 289px" /></figure></div>


<p>Here is the graph of f(x) = -3|x|.&nbsp;</p>



<p>If x ≥ 0, f(x) = -3x&nbsp;&nbsp;</p>



<p>If x &lt; 0, f(x) = 3x.   </p>


<div class="wp-block-image">
<figure class="aligncenter size-full is-resized"><img loading="lazy" decoding="async" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/The-graph.webp" alt="The graph" class="wp-image-48233" style="width:368px;height:171px" width="368" height="171" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/The-graph.webp 560w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/The-graph-300x139.webp 300w" sizes="auto, (max-width: 368px) 100vw, 368px" /></figure></div>


<p><strong>Question 2</strong>&nbsp;</p>



<p><strong>Graph the following function: </strong> </p>


<div class="wp-block-image">
<figure class="aligncenter size-full is-resized"><img loading="lazy" decoding="async" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Question-2.webp" alt="Question 2" class="wp-image-48234" style="width:380px;height:160px" width="380" height="160" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Question-2.webp 618w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Question-2-300x126.webp 300w" sizes="auto, (max-width: 380px) 100vw, 380px" /></figure></div>


<p><strong>Solution: </strong> </p>


<div class="wp-block-image">
<figure class="aligncenter size-full"><img loading="lazy" decoding="async" width="330" height="414" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Solution.webp" alt="Solution" class="wp-image-48235" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Solution.webp 330w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/Solution-239x300.webp 239w" sizes="auto, (max-width: 330px) 100vw, 330px" /></figure></div>


<p>x = -2, f(x) = -4&nbsp;&nbsp;</p>



<p>x = -1, f(x) = -3&nbsp;&nbsp;</p>



<p>x = 0, f(x) = -2&nbsp;&nbsp;</p>



<p>x = 1, f(x) = -1&nbsp;&nbsp;</p>



<p>x = 2, f(x) = -1&nbsp;&nbsp;</p>



<p>x = 3, f(x) = -3&nbsp;&nbsp;</p>



<p>x = 4, f(x) = -5&nbsp;&nbsp;</p>



<p><strong>Question 3</strong>&nbsp;</p>



<p><strong>In the example explained in section 1.4, what is the difference in cost between one order of 200 wristbands, two orders of 100 wristbands each, and four orders of 50 wristbands each?</strong>&nbsp;</p>



<p><strong>Solution: </strong> </p>


<div class="wp-block-image">
<figure class="aligncenter size-full is-resized"><img loading="lazy" decoding="async" src="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/50-wristbands.webp" alt="50 wristbands" class="wp-image-48236" style="width:684px;height:156px" width="684" height="156" srcset="https://www.turito.com/learn-internal/wp-content/uploads/2023/08/50-wristbands.webp 1024w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/50-wristbands-300x69.webp 300w, https://www.turito.com/learn-internal/wp-content/uploads/2023/08/50-wristbands-768x176.webp 768w" sizes="auto, (max-width: 684px) 100vw, 684px" /></figure></div>


<p>x = 200&nbsp;&nbsp;</p>



<p>f(x) = 0.5x = 0.5 × 200 = 100&nbsp;&nbsp;</p>



<p>1 order of 200 wristbands costs $100.&nbsp;&nbsp;</p>



<p>x = 100&nbsp;&nbsp;</p>



<p>f(x) = x + 10 = 100 + 10 = $110&nbsp;&nbsp;</p>



<p>2 orders of 100 wristbands cost $220.&nbsp;&nbsp;</p>



<p>x = 50&nbsp;</p>



<p>f(x) = 2x + 20 = 2 × 50 + 20 = $120&nbsp;&nbsp;</p>



<p>4 orders of 50 wristbands cost $480.  </p>



<h4 class="wp-block-heading">Exercise</h4>



<p>Express the following functions as piecewise-defined functions:</p>



<ol class="wp-block-list" type="1">
<li>f(x) = 0.6|x|</li>



<li>f(x) = (1/7) |x|</li>



<li>f(x) = Ix – 100I</li>



<li>f(x) = 3|x|</li>



<li>f(x) = 2 |x-1000|</li>



<li>f(x) = (1/3) |x-100|</li>



<li>f(x) = 4.5|x|</li>



<li>f(x) = 0.5|x+10|</li>



<li>f(x) = 10|x|</li>



<li>f(x) = 9|x+100|</li>
</ol>
