Solving System of Equations by Elimination

There would have been various situations in your life where you have to deal with two or more physical quantities and need to find their values. To find the values of these quantities, you must learn how to solve equations, particularly solving system of equations by elimination.

Go through the solved examples thoroughly to understand the concept in-depth and master the techniques to solve the questions effectively. 

What is an Equation?

We do not know the exact number in a calculation, so we use Algebra. The term “algebra” was derived from “al-jabr,” meaning adding a number to both sides of an equation to either simplify or cancel terms.

An equation is a mathematical statement consisting of two algebraic expressions with an equal symbol. For example, 7x+2y +2 =13. Here 7 and 2 are the coefficients, and x and y are the variables. They are the unknown values in the equation. Also, 2 and 13 are called constants. The expression to the right of the equal sign is called the right-hand side, and that to the left is called the left-hand side of the equation.

Why are Equations Important?

The equals sign carries great importance because it signifies mathematical equality. Equations are very important in life because they help us clarify results. The most famous and used equation is:

Pythagoras theorem

That states, c²=a²+b², where c is the hypotenuse and a and b are the sides of a right-angled triangle.

Also, in today’s world, computer chips are used in all the machines we use in our daily routine, like automobiles, phones, washers, etc. Every chip in every machine is based on mathematical equations and algorithms.

Even the internet works on algorithms that comprise various equations. When you surf the internet, you search for a particular thing, and the search engine gives you results related to the search. This whole process works on an algorithm.

Solving system of Equations by Elimination

Do you know how to solve systems of equations by elimination? If not, then you are at the right place. There are several ways to solve the system of equations. One way is by using graphs. Graphing works well when the coefficients of the variables in the equation are small, easy to deal with, and the solution has integer values.

Another method is substitution. Substitution works well when we can easily solve one equation for one of the variables and when there are not too many fractions in the resulting expression.

Lastly, the third method of solving equations/linear equations systems is called the Elimination Method. After solving a system by substitution, we get two equations and two variables. The elimination process works on these equations in a different way to get to the solution: the value of the variables.

The Elimination Method is based on the Addition Property of Equality. The Addition Property of Equality states that you still have equality when you add the same quantity to both sides of an equation. We will look further into the Addition Property of Equality and solve our system of linear equations.

Systems of Linear Equations

A system of linear equations can be defined as a set of two or more linear equations. With two variables, suppose x and y, the graph of a system of two equations is a pair of lines in the plane.

There are three possibilities:

• The lines intersect at zero points. (The lines are parallel.)
• The lines intersect at exactly one point. (Most cases.)
• The lines intersect at infinitely many points. (The two equations represent the same line.)

Using Multiplication and Addition to Eliminate Variables

Here is how you can solve a system of equations when multiplication is necessary to eliminate a variable. When adding the equations or adding the opposite of one of the equations will not give you the desired result. Let us look at an example.

3x+4y=52

5x+y =30

Even after adding the above two equations or adding the opposite of anyone’s equation, you will still get an equation that has two variables. Let us now see how the multiplication property helps us get the results. First, let us multiply both sides of one of the equations by a number that allows you to eliminate the same variable in the other equation.

We do this with multiplication.  Notice that the first equation contains the term 

4y and the second equation contains the term y. If you multiply the second equation by −4 and then add both equations, the y variables will add up to 0. 

Let us look at the same example of solving systems of linear equations by elimination. 

Example 1 

Solve for x and y.

Equation A: 3x+4y=52

Equation B: 5x+y=30

To solve this question, you do not need to learn solving systems of equations by elimination calculator. You need to find the terms that can be eliminated. The equations do not have any x or y terms with the same coefficients.

Therefore here we use multiplication.

Multiply the second equation by −4 so the y variable in both equations has the same coefficient.

3x+4y=52

−4(5x+y)=−4(30)

Multiple and then add the equations.

3x+4y=52

−20x–4y=−120

Solve for x

−17x=−68

x=4

Now, Substitute x=4 into one of the original equations to find y.

3x+4y=52

3(4)+4y=52

12+4y=52

4y=40

y=10

Answer:

The solution is 

x= 4, y=10

Example 2

A park charges $10 for adults and $5 for kids. How many adult tickets and kids tickets were sold if a total of 548 tickets were sold for a total of $3750? 

Solution :

To solve this, you do not need to learn solving systems of equations by elimination calculator. Let x be the number of adult tickets and y be the number of kids’ tickets.

According to the question, we have

x + y  =  548 ———(1)

And also,

10x + 5y  =  3750

Divide both sides by 5.

2x + y  =  750 ——–(2)

Now, 

We will eliminate one of the variables to get the value of the other variable.

In (1) and (2), variable y is having the same coefficient. But, the variable y is having the same sign in both equations. 

To change the sign of y in (1), multiply both sides of (1) by a negative sign.

– (x + y)  =  – 548

– x – y  =  – 548 ——–(3)

Adding equations 2 and 3, we get

x=202

Substitute x = 202 in (1) to get the value of y. 

               (2) ——–> 202 + y  =  548 

Subtract 202 from both sides. 

y  =  346

Therefore, the number of adult tickets sold is 202 and the number of kids tickets sold is 346.

Example 3

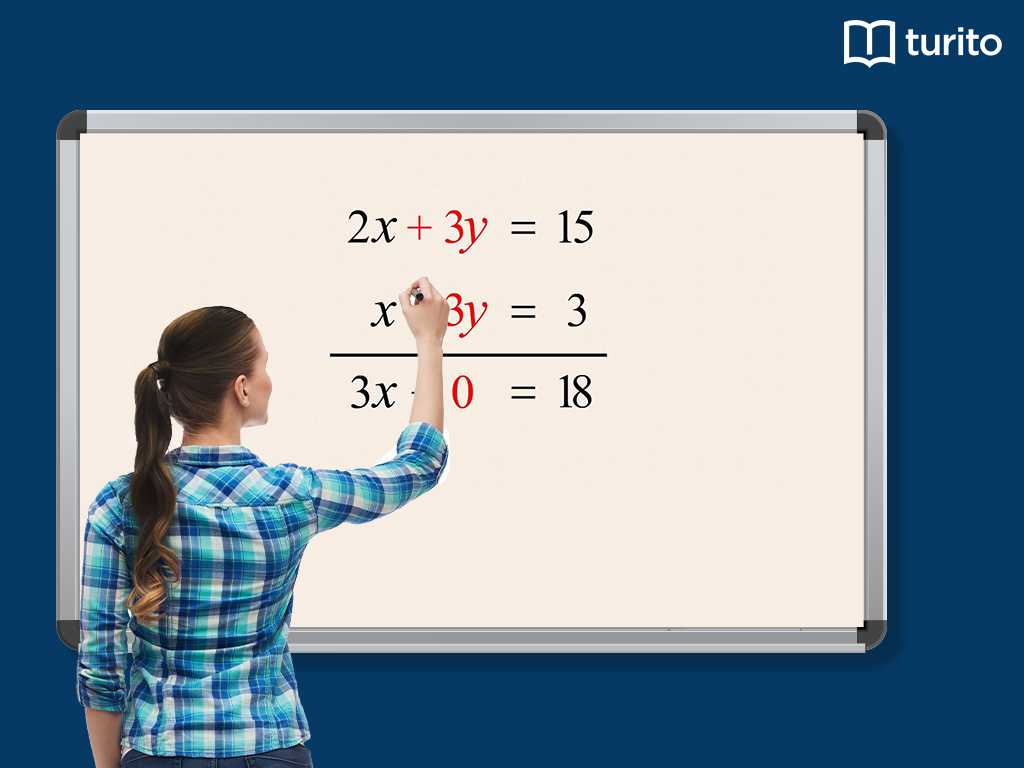
Solve the system of equation 2x + 3y = 11, x + 2y = 7 by the method of elimination.

Solution:

The given equations are:

2x + 3y = 11       …………… (i)

x + 2y = 7       …………… (ii)

Multiply the equation (ii) by 2, we get

{x + 2y = 7}       …………… (× 2)

2x + 4y = 14       …………… (iii)

Subtract equation (i) and (ii), we get

y=3

Substituting the value of y = 3 in equation (i), we get 

2x + 3y = 11 

2x + 3 × 3 = 11

2x + 9 = 11

2x = 11 – 9

2x = 2 

x = 1

Therefore, the solution of the system of the given equations is  x = 1 and y = 3 . 

Example 4

 x/2 + 2/3 y = -1 and x – 1/3 y = 3

Solution:

The given equations are:

x/2 + 2/3 y = -1       …………… (i)

x – 1/3 y = 3       …………… (ii)

Multiply equation (i) by 6 and (ii) by 3, we get;

3x + 4y = -6       …………… (iii)

3x – y = 9       …………… (iv)

Solving (iii) and (iv), we get;

y= -3

Subtracting the value of y in (ii), we get;

x – 1/3̶ × -3̶ = 3

or, x + 1 = 3

or, x = 3 – 1

or, x = 2

Therefore, x = 2 and y = -3 is the solution of the equation.

x/2 + 2/3 y = -1 and x – y/3 = 3

Example 5

Solve the system by elimination.  

−6x+15y=10

2x−5y=−5

Multiply the second equation by 3 to eliminate a variable.

−6x+15y=10

3(2x−5y)=3(−5)

Simplify and add.

−6x+15y=10 and 6x−15y=−15

We get the result as 

0≠5

Therefore we can conclude that the statement is false. Because the equations are inconsistent, so their graphs would be parallel lines.

Therefore, the system does not have a solution.

Frequently Asked Questions

1. What is the Elimination Method in Math?

Ans. The elimination method is a way to solve a system of equations with two variables. This method works by creating a new equation that eliminates one of the variables from both original equations, so you can solve for that variable.

2. How do you Solve Linear Equations Using the Elimination Method?

Ans. Solve linear equations using the elimination method by first dividing both sides of the equation by the coefficient of the variable you are solving for. For example, let’s say you have the equation 3x + 4 = 12 and want to solve for x. In this case, we would divide both sides by 3. This leaves us with x + 4/3 = 12/3 or x = 12/3 – 4. The solution is then x = 8/3.

3. What are the Steps Involved in the Elimination Method?

Ans. Step 1: List all of the possible values for each variable.

Step 2: Assign a value to each variable based on the conditions of the problem.

Step 3: Solve the remaining variable in each equation.

Step 4: Check your answers by substituting them back into each original equation.

4. When Should you Use the Elimination Method?

Ans. The elimination method is a way to solve problems by systematically eliminating all possible answers until only one remain. It’s most commonly used when you have several different ways to solve a problem and you need to find the right one.

The elimination method works well when you have a lot of information that can’t be used yet, because it helps you narrow down your options without getting bogged down in details.

The elimination method works best when:

You’re trying to solve a problem with multiple solutions, but only want one solution

You’re solving a problem that has many steps or parts

5. Why is Elimination Method Better?

Ans. The elimination method is better because it helps you to identify which variable is the cause of the problem. This allows you to quickly eliminate variables and find the solution to your problem.