Special Right Triangles: Types, Formulas, with Solved Examples.

Learn all about special right triangles– their types, formulas, and examples explained in detail for a better understanding. What are the shortcut ratios for the side lengths of special right triangles 30 60 90 and 45 45 90? How are these ratios related to the Pythagorean theorem? 

Right Angle Triangles

A triangle with a ninety-degree angle (also called a right angle) is called a right triangle. The side of the triangle that lies opposite to the right angle is called the triangle’s hypotenuse. The other two sides of the triangle are called the legs or the base and the triangle’s height. 

Since one angle is ninety degrees, the other two angles are always complementary. We can prove this as follows:

The sum of angles of a triangle = 180 degrees

Given: The triangle has a right angle, i.e., 90 degrees.

So, if the other two angles are x and y, 

We can write the equation as  90 + x + y = 180.

So, x + y = 90.

Thus, the two angles will always be complementary. Each will measure less than ninety degrees. 

What are Special Right Triangles?

A special right triangle is a right-angled triangle exhibiting some regular feature that makes triangle calculations easier as it has simple formulas. 

For instance, a right triangle with angles forming simple relationships, such as 45°–45°–90°, is an “angle-based” right triangle. On the other hand, a “side-based” right triangle has lengths of the sides forming ratios of whole numbers- 3: 4: 5. , or of other special numbers such as the golden ratio. 

If we know the relationships of the angles or ratios of sides of special right triangles, we can quickly calculate various lengths without having to resort to advanced methods.

Length of hypotenuse c² = a² + b², where a and b are the lengths of the triangle legs.

Length of a leg a² = c² – b², where c is the hypotenuse length, and b is the length of the other leg.

Types of Special Right Triangles

Special right triangles are angle-based, i.e., they are specified by the relationships of their angles. The angles are related as follows:

• The largest angle is 90 degrees. 
• The largest angle of the triangle is equal to the sum of the other two angles.
• The side opposite to the right angle is the hypotenuse. It is the longest side of the right-angle triangle.
• The altitude of a triangle arising from the right angle to the hypotenuse equally divides the main triangle into two similar triangles. These two triangles are also similar to the main triangle.
• We can deduce the side lengths from the basis of the unit circle or other geometric methods. It rapidly reproduces the values of trigonometric functions for the angles 30°, 45°, and 60°.
• The sides of these special right triangles are in particular ratios known as Pythagorean triples.
• Another important characteristic of special right triangles is that their legs are also the altitudes of the triangles. Thus, the area of a special right triangle is one-half the product of the legs’ lengths. 

The Two Main Types of Special Right Triangles are

• Special right triangles 30 60 90
• Special right triangles 45 45 90 

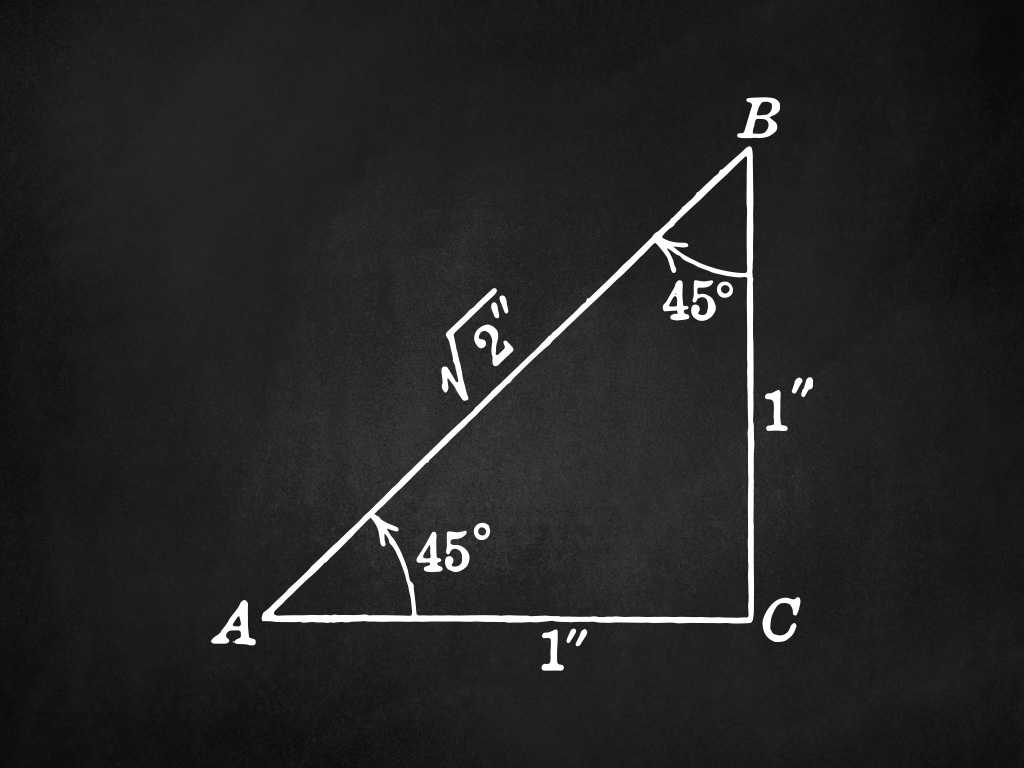
The 45°; 45°; 90° Special Right Triangle

This special right triangle has angles measuring 45°, 45°, and 90°. The ratio between the base, the height, and the hypotenuse of this triangle is 1: 1: √2.

Base: Height: Hypotenuse = x: x: x√2 = 1: 1: √2

This special right triangle is also an isosceles triangle. An isosceles triangle is one that has two side lengths equal. Its two angles are also equal.   

We can use the equation of a right triangle (or Pythagoras theorem) to calculate the hypotenuse of a 45°; 45°; 90° triangle as follows:

a² + b² = c²

base² + height² = Hypotenuse² 

Given that a 45°; 45°; 90° triangle is an isosceles triangle;

So, we can write the two sides as a = b = x;

Now, x² + x² = 2x²

Take square root on both sides of the equation

√x² + √x² = √(2x²)

x + x = x √2

Thus, the hypotenuse of a 45°; 45°; 90° special right triangle is x √2.

The 30°; 60°; 90° Special Right Triangle

This triangle has angles measuring 30°; 60°; 90°.  The ratio of the lengths of the triangle sides is x: x√3: 2x.

Base: Height: Hypotenuse = x: x√3: 2x

Special Right Triangles Formula

The special right triangle formula provides the ratio of the sides. The base, height, and hypotenuse of special right triangles 45 45 90 degrees are in a ratio: 

1: 1: √2

The base, height, and hypotenuse of special right triangles 30 60 90 degrees are in a ratio of

1: √3: 2

How to Solve Special Right Triangles Problems?

We mainly have to find the missing lengths of the sides while solving special right triangle problem questions. Here are a few examples of special right triangles that will help you understand how to implement the formula.

Example 1: Find the two sides of the special right triangle if the base of the triangle is 5√3. The angles measure 30, 60, and 90 degrees. Solution: Given: Base = 5√3  Now, using the special right triangles formula, the base, height, and hypotenuse of a triangle (angles 30, 60, and 90) are in a ratio of 1:√3: 2. Let the base be x= 5√3   Height = (5√3)√3= 5×3 = 15 Hypotenuse = 2x = 2×5√3 = 10√3

Example 2: Find the two sides of the special right triangle if the base of the triangle is 2√3. The angles measure 30, 60, and 90 degrees. Solution: Given: Base = 5√3  Now, using a special right triangles formula, the base, height, and hypotenuse of a triangle (angles 30, 60, and 90) are in a ratio of 1:√3: 2. Let the base be x= 2√3   Height = (2√3)√3= 2×3 = 6 Hypotenuse = 2x = 2×2√3 = 4√3

Example 2: What will be the hypotenuse of a special right triangle 30°- 60°- 90° whose longer side measures 6 inches? Solution: The ratio sides of special right triangles 30 60 90 = x: x√3:2x. ⇒ x√3 = 6 inches (given) Squaring both sides ⇒ (x√3)2 = 62 ⇒ 3x2 = 36 x2 = 12 x = 2√3 inches

Example 3: If the diagonal of the special right triangle is 10 cm, what will be the length of the other two sides of the triangle. Given one of its angles is 30 degrees. Solution: This is a 30°-60°-90° special right triangle, so we will use the ratio of x: x√3:2x. Given, the diagonal = hypotenuse = 10cm. ⇒2x = 10 cm ⇒ x = 5cm Substituting the value of x. x√3 = 5√3 cm The shorter side of the right triangle is 5cm, and the longer side is 5√3 cm.

Example 4: If you are on the top of a building, looking at a park in the distance, and the angle of depression is 53°. If the building is 100 feet tall, what is its distance from the park?  Solution: Given the angle of depression is 53°, we can calculate the third angle as 180-(90+53) = 180 -143. The third angle is 37°. Using the tangent function, we can find the distance of the building from the park. tan 37 = opposite/ adjacent tan 37 = d/100 d= 100 tan( 37) = 75.36 feet  The distance between the building and the park is 75.36 feet.

Practice Problems Question 1: The longer side of a special right triangle 30°; 60°; 90° is 8√3 cm. What is the measure of its hypotenuse and height? Question 2: The hypotenuse of a special right triangle 45°; 45°; 90° is 6√2 mm. What is the length of its base and height? Question 3: Given the diagonal of a right triangle measures 8 cm, what will be the length of the other two sides of the triangle if one of its angles is 30 degrees? Question 4: A ladder is inclined against a wall and makes an angle of 30 degrees with the ground. Given the length of the ladder is 9 m. Find the following: a. The height of the wall. b. The length between the wall and the foot of the ladder.  Question 5: The longer side of a special right triangle 30 60 90 is 12√3 cm. Find the measure of its hypotenuse.

Applications of Special Right Triangle Formula

There are several applications of special right triangles in real life. Some of the most common applications of special right triangles are:

• It is used in the branch of trigonometry.
• It provides a relation between its angles and sides to form the basis for trigonometry.
• It is also applicable in the construction and engineering field.
• We can use right triangles to measure distances. So, if we know the angle of elevation or an angle of depression, we can find the distance.

Frequently Asked Questions

1. What Are the Special Right Triangles

Ans. Special right triangles are triangles that have specific measurements. There are three types of special right triangles: equilateral, isosceles, and scalene. These three types are distinguished by their properties: the length of their sides and the size of their angles.

2. What Are the 2 Special Right Triangles?

Ans. There are two special right triangles: the 3-4-5 triangle and the 5-12-13 triangle. The 3-4-5 triangle has sides of 3, 4, and 5 units, while the 5-12-13 triangle has sides of 5, 12, and 13 units.

3. What Is the Special Right Triangle Formula in Geometry?

Ans. The special right triangle formula is the sum of the squares of two sides of a right triangle. The formula is:

a^2 + b^2 = c^2

Where c is the hypotenuse or longest side, and a and b are the other two sides.

4. How to Solve a Special Right Triangle?

Ans. Here’s how to solve special right triangles:

• First, draw the triangle ultimately.
• Next, label the three corners of the triangle with the letters A, B, and C.
• Then use the Pythagorean Theorem to solve for side “c”, which is the hypotenuse.

5. How to Find the Hypotenuse of a Special Right Triangle 30-60-90?

Ans. The hypotenuse, or longest side of a right triangle, is the side opposite the right angle. In a 30-60-90 triangle, the hypotenuse is always equal to the square root of 3 times the length of one of the other sides. For example, if you have a triangle with sides of length 10 and 20, then your hypotenuse will be 10 multiplied by itself times 3 (because it’s opposite a 60-degree angle), or 100.