Standard Deviation – Definition, Formula, Examples

Have you ever wondered why some localities have higher house rents while the other areas have lower rent rates? Because of demand! That is true. Then how does a real estate broker decide what should be the price of these areas? This is one of the situations where standard deviation comes into play. And now you might be thinking, what is standard deviation! 

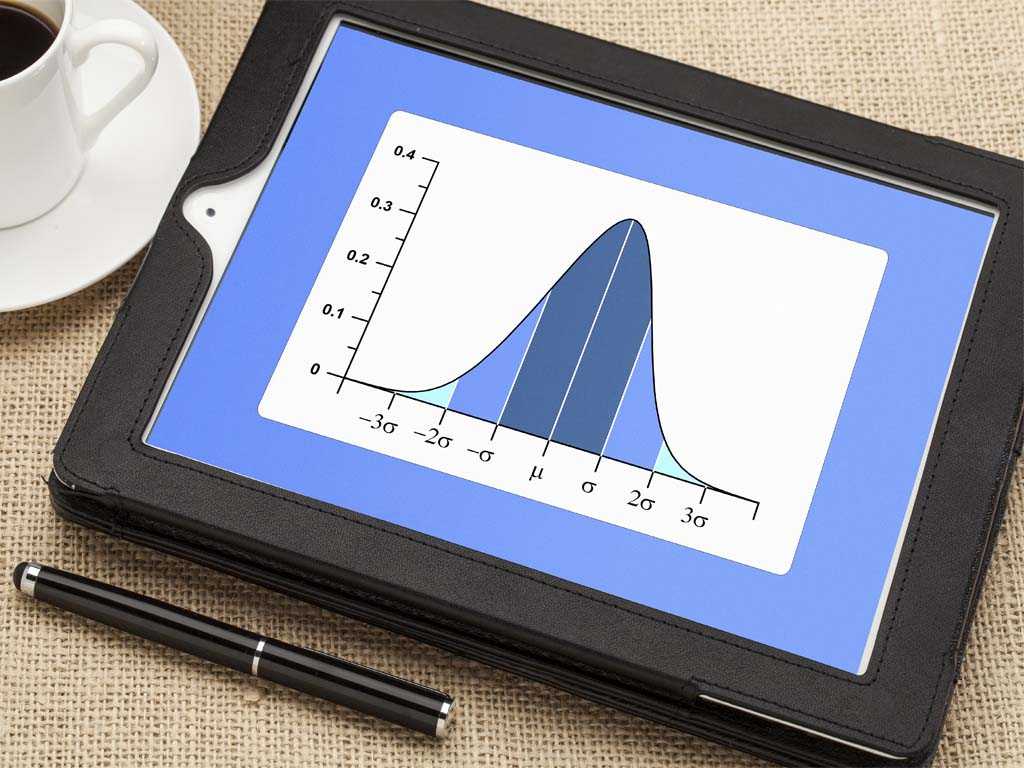
Understanding standard deviation means – Standard deviation is data disbursement. It tells how much your data is spread out, especially by showing the mean or average of the data given. The graph below shows the normal distribution of a large amount of data. The mean or average is denoted by μ, where the standard deviation symbol is σ. The colored bar represents the standard deviation away from the mean.  

A normal distribution can represent numerous situations in one’s life. It can represent a large amount of data belonging to any sector or field. For example, it can represent your school’s entire data in a single graph. How many marks each class scores and how it varies from the top marks of other classes can be represented on a normal distribution curve. This knowledge enables big companies to predict their future scenarios and plan accordingly. 

Standard deviation definition states it is a statistical measure to understand how reliable data is. A low standard deviation means the data is very close to the average. This means that the data is reliable. A high standard deviation denotes a large variance between the data and its average. Thus, it is not reliable. 

Standard deviation equation 

You might have trouble finding the standard deviation. Then, you can use the following formula to learn how to find the standard deviation of a given data set. 

Population formula:

The population standard deviation formula is given as:

Here,

σ = Population standard deviation

Sample standard deviation formula:  

Here,

s = Sample standard deviation

Steps to determine the standard deviation 

You can follow the steps given below to find the standard deviation:

Step 1: Study the data and determine its average. The average or mean could be found by adding all the numbers and dividing them by items. 

Step 2: Now, subtract the mean from each value given in the data set.

Step 3: Square the differences you obtained in step 2. Note: It is necessary to square the differences. Otherwise, they will cancel each other, resulting in zero value in the next step.

Step 4: You need to determine the average of the squared numbers. If given sample sizes, subtract 1 from the total number of items to find the average.

Step 5: The value you will get in step 4 will be the variance. You need to find the square root of the variance to get the standard deviation. 

Let us understand this by taking the values 2, 1, 3, 2, and 4.

• As indicated in step 1, you need to determine the mean (average):

2 + 1 +3 + 2 + 4 = 12

12 ÷ 5 = 2.4 (mean)

• As stated in step 2, you need to subtract the mean from each value:

2 – 2.4 = -0.4

1 – 2.4 = -1.4

3 – 2.4 = 0.6

2 – 2.4 = -0.4

4 – 2.4 = 1.6

• Square each of those differences obtained in step 2:

-0.4 x -0.4 = 0.16

-1.4 x -1.4 = 1.96

0.6 x 0.6 = 0.36

-0.4 x -0.4 = 0.16

1.6 x 1.6 = 2.56

• You need to determine the average of the squared numbers to get the variance.

0.16 + 1.96 + 0.36 + 0.16 + 2.56 = 5.2

5.2 ÷ 5 = 1.04 (variance)

• Find the square root of the variance to get the standard deviation.

The square root of 1.04 = 1.01. 

The standard deviation of the values 2, 1, 3, 2, and 4 is 1.01.

Finding a standard deviation from variance

Some students might find calculating variance easier than the standard deviation. You must also know you can find a standard deviation from variance. The formula to find the variance of a given data set is given:

Population formula:

σ² = Population variance

N = Number of observations in population

X_i = i^th observation in the population

μ = Population mean

Sample variance formula: 

Here,

s² = Sample variance

n = Number of observations in sample

X_i = i^th observation in the sample

x̅ = Sample mean

To find standard deviation in easier terms, you can find the square root of the variance. And to find the variance, you can square the standard deviation, depending upon the data you are given. 

Applications of standard deviation

As stated at the beginning of this article, standard deviations are used by real estate brokers to find what can be the price of a house for rent in any particular area. This helps them inform their clients of the type of variation in house prices they can expect. Furthermore, there are other uses of standard deviation as well. They are

• Use of standard deviation in human resources: Human Resource Managers use standard deviation to figure out salaries of a certain field to know how much variation is there in a particular company. 
• Standard deviation in marketing: Marketers often use it to analyze how their company performs. They calculate their revenue earned per advertisement and how much variation can be expected from the given ad. It helps them understand their competitors better. 
• Usage in test scores: Standard deviation is a widely used concept by professors in universities and schools. They take the help of standard deviation to calculate the final scores to figure out whether the student has scored near to the average score or not. They even calculate the variation for different classes of students to figure out which class got the highest variation in test scores. 

Key points about standard deviation 

• It is a measure to showcase how far the data is. It denotes the dispersion of the data. 
• Standard deviation is the root-mean-square of the data given. 
• It is expressed in the same units as that of the mean. 
• The standard deviation symbol is 𝜎. 
• It is used to indicate the number of observations in the data spread. 

Example 1: What is the standard deviation of rolling a die’s possibilities?

Solution: The sample space of rolling of a die is {1, 2, 3, 4, 5, 6}. 

The mean of the sample space = x̅ = (1 + 2 + 3 + 4 + 5 + 6) / 6 = 3.5

We will find standard deviation by using the variance formula. We know, the variance is given by: 

σ² = Σ (x_i – x̅)² / n

σ² = ⅙ (6.25 + 2.25 + 0.25 + 0.25 + 2.25 + 6.25)

σ² = 2.917

And, the standard deviation is the square root of variance. Therefore, the standard deviation, σ = √2.917 = 1.708

Example 2: Find the standard deviation for the given data set:

{12, 15, 17, 20, 30, 31, 43, 44, 54}

Solution: To find the standard deviation of the given data set, you must understand the following steps.

Step 1: Add the given numbers of the data set: 

12 + 15 + 17 + 20 + 30 + 31 + 43 + 44 + 54 = 266

Step 2: Next, square the answer from Step 1:

266 x 266 = 70756

Step 3: Divide the output of Step 2 by the number of items (n) given in your data set. In the given question, you have 9 items. Therefore:

70756 / 9 = 7861.777777777777 (dividing by n)

Step 4: Keep the output aside. Let’s begin with another step. Square the original numbers {12, 15, 17, 20, 30, 31, 43, 44, 54} one at a time, then add them up:

(12 x 12) + (15 x 15) + (17 x 17) + (20 x 20) + (30 x 30) + (31 x 31) + (43 x 43) + (44 x 44) + (54 x 54) = 9620

Step 5: Now subtract the output of step 4 from that obtained in step 3.

9620 – 7861.777777777777 = 1758.2222222222226

You need to keep in mind not to round off the digits in any of these steps. Rounding off can be done at the end when the final answer comes up. Rounding off every step will create differences from the final one. 

Step 6: You need to subtract 1 from n. Since you have 9 items, so n = 9:

9 – 1 = 8

Step 7: Divide Step 5 by Step 6 to get the variance:

1758.2222222222226 / 8 = 219.77777777777783

Step 8: Take the square root of Step 7:

√(219.77777777777783) = 14.824903971958058

The standard deviation is 14.825.

Frequently Asked Question?

1. What is the formula for finding the standard deviation?

The formula for finding the standard deviation is:

StDev = √[(x – x̅)^2/n]

2. How do you calculate standard deviation in statistics?

 Standard deviation is a measure of the dispersion of a data set from its mean. It is calculated by taking the square root of the variance and dividing it by the number of values in the data set. To calculate the standard deviation, you must first find the variance and then divide it by n-1, where n is the number of values in your data set.

3. When would you use standard deviation?

The standard deviation is a measure of how far data values are from the mean. It’s used to determine how much variation there is in a set of data.

4. What are the “three-standard-deviations rule”?

The “three-standard deviation rule” determines whether you have enough data to conclude. It’s based on the fact that when you’re trying to determine if something is a trend, you need to make sure there are enough data points to create a meaningful pattern. 

5. What does standard deviation tell us?

Standard deviation tells us how much our data varies from the mean. If the standard deviation is low, then our data points are close together. If the standard deviation is high, then our data points are spread apart.