Divisor: Definition, Formula, Properties, with Solved Examples.

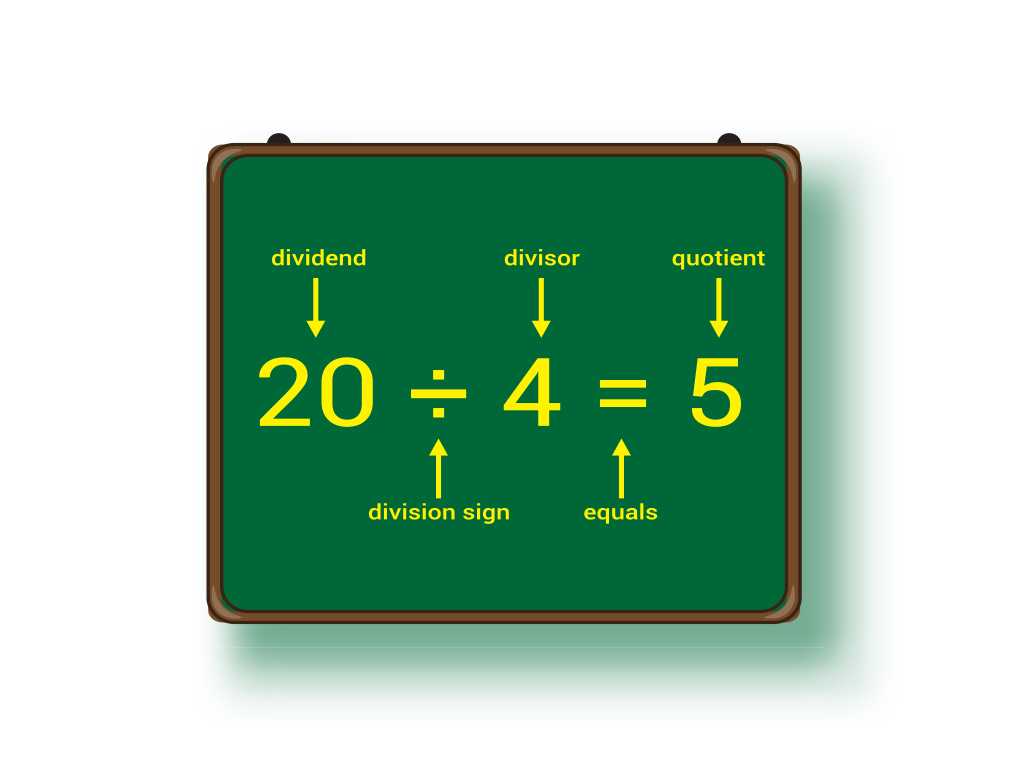
At its core, four fundamental arithmetic operations form the basis of mathematics. These four primary operations are addition, subtraction, multiplication, and division. The direct meaning of division is to break/divide a number into equal parts. Four main terms of the division operation are called the dividend, divisor, quotient, and remainder. The divisor is the number that performs the division operation on another number. The dividend is the number upon which the division operation is being done. The quotient is the final result we get after the division process is complete. Finally, the remainder is the portion of the dividend that’s left after the division operation is over. 

Meaning of Division

The division is an elementary arithmetic operation that is highly utilized in every realm of mathematics. Its purpose is to break a number into equal parts. 

For example, when we divide the number 20 by the number 4, we get the answer 5. We have divided the number 20 into 4 equal parts, with the value of each part equal to 5.

Division Symbol

Many symbols are used to represent the division operation. However, the two most heavily used symbols for division representation are “÷” and the fraction backslash “/”. The backlash is mostly used for fractions, where the numerator is written at the top and the denominator at the bottom.

For example, the statement 10 divided by 5 can be written as:

• 10÷5
• 10/5 

General Form of Division

Division can also be looked at as a process of repetitive subtraction. One of the popular methods to perform the division operation is the Long Division Method, where we keep on dividing the dividend with the divisor until we get zero remainders. At each step, we subtract from the dividend the greatest multiple of the divisor equal to or less than the divided. 

Given below is the general formula for division:

Dividend = (Divisor × Quotient) + Remainder

The division is essentially a reverse process of multiplication. This fact helps us verify our result by substituting the appropriate values in the general formula for division.

What is a Divisor?

In the process of division, the divisor is one of the important terms apart from the dividend, quotient, and remainder. The divisor represents the number that is dividing the dividend. Essentially it means the number of equal parts that we need to break the dividend into.

Let us answer the question of what is a divisor in more detail by having a look at the divisor definition.

Divisor Definition

A Divisor is a Number that Divides the Other Number in the Calculation. Divisor definition states that it is the term performing the division operation on the dividend. 

For example, when we divide the number 28 by the number 7, 7 is called the divisor, whereas the number 28 is called the dividend.

Now that we have answered what a divisor is, let us see a few more important concepts.

Formula for Divisor

Rearranging the terms in the general division formula, we can obtain the following formula.

Divisor = (Dividend – Remainder) / Quotient 

Divisor and its Examples

Let us consider the statement 33÷11=3. Here, the number 33 is the dividend, the number 11 is the divisor, and the number 3 is the quotient.

Let us consider the statement 50÷10=5. Here, the number 50 is the dividend, the number 10 is the divisor, and the number 5 is the quotient.

Interesting Properties of Divisor

The divisor is the number that divides the dividend into equal parts. However, it may or may not leave a remainder. Given below are some interesting properties of the divisor.

• The remainder is always less than the divisor for any division process.
• 1 is the divisor of all the numbers.
• If the quotient is equal to the dividend, then the divisor will always be equal to 1. 
• If the dividend is equal to the divisor, then the quotient will always be equal to 1.
• If the remainder is zero, this implies that the number is a perfect divisor of the dividend and can be divided completely.
• If the divisor is larger than the dividend, then the quotient will always be equal to a decimal number.

Divisors and Factors

The job of the divisor is to divide the dividend. If the divisor completely divides the dividend, leaving no remainder, then the divisor is termed a dividend factor. Therefore, all the number factors are also its divisors, but all the divisors of a number are not its factors.

Example 1: Consider the number 8. 1, 2, 4, and 8 are numbers that completely divide the number 8, leaving no remainders. These numbers are the factors as well as the divisor. 

Example 2: Consider the division of 12 by 5. After the division operation, we get 2 as the quotient and the remainder. Since the remainder is non-zero, the divisor is not capable of completely dividing the dividend in this case. Here 5 is a divisor of 12 but not its factor.

Example 3: Consider 15 divided by 5 gives 3. Here, 5 is a divisor.  Also, by dividing 15 by 3, we get 5. Here, 3 is a divisor. 

Dividend vs Divisor

Divisor and dividend are two of the main components of the division process. Using these two terms, we obtain the remaining components of the division operation – the quotient and the remainder. Let us understand the dividend vs. divisor difference from their definition.

The divisor is the number that performs the division operation on the dividend. The dividend is the number that the divisor performs the division operation upon. 

For even more clarity on dividend vs. divisor, consider the following example.

When we divide the number 60 by the number 15, we get 4 as the quotient and 0 as the remainder. The number 15, which performs the division operation, is called the divisor. The dividend is the number 60 on which the division operation is being performed.

Remainder

The remainder is another important term of the division operation. It is the leftover portion of the dividend after it is divided by the divisor. When the divisor is incapable of dividing the dividend into equal parts, we receive a non-zero remainder as a result. Some important properties of the remainder are given below.

The divisor is always greater than the obtained remainder for every division operation. If the remainder obtained is greater than or equal to the divisor, then it signifies that we’ve performed the division incorrectly.

Regarding the quotient, the remainder can be greater, smaller, or even equal to the quotient.

If the divisor can perfectly divide the dividend, then the remainder is always zero.

Long Division Method Example No. 1

Consider the division of 25 by 12.

Here 25 is the dividend and 12 is the divisor. On solving the division operation we get 2 as the quotient and 1 as the remainder.

Long Division Method Example No. 2

Consider the division of 235 by 15.

Here 235 is the dividend and 15 is the divisor. On solving the division operation we get 15 as the quotient and 10 as the remainder.

Fascinating facts about division

• De Morgan in 1845 introduced the oblique bar used as a sign of that process in the division.
• The oblique bar symbol used for the division was introduced by De Morgan in 1845.
• According to the rules of arithmetic, the division of a number by zero is undefined.
• The division is also represented by the term colon in some non-English-speaking countries. Gottfried Wilhelm Leibniz introduced this term in his 1684 Acta Eruditorum.
• Swiss Mathematician Johann Rahn introduced the obelus symbol “÷” in 1659.

Frequently Asked Questions

1. What is a divisor?

A divisor is a number that divides another number called the dividend to give a result. The quotient and the leftover portion of the divide are called the remainder. 

2. Can a number be called a divisor of itself?

Every number acts as a divisor of itself. On dividing a number by itself, it gives the quotient of 1 and the remainder as zero. 

3. Are negative divisors possible?

Yes, divisors can be positive and negative as well. We usually only consider the positive numbers as divisors. For example, the number 6 has a total of eight divisors: -6, -3, -2, -1, and 1, 2, 3, 6. However, we only consider the positive terms here.

4. How do we recognize the divisor and dividend in fractions?

Fractions or rational numbers are presented in the p/q form. Here p is called the numerator that is also the dividend. The term q denotes the denominator which is also the divisor.

5. What are prime divisors?

The divisors that also satisfy the condition of being a prime number are called prime divisors. For example, 1 is a divisor of every number since it is not a prime number; hence it is not a prime divisor.

6. What is the formula to calculate a divisor? 

Divisor = (Dividend – Remainder) / Quotient is the formula to calculate the divisor 

7. What distinguishes the factor from the divisor? 

Any number that divides another number is a divisor. However, a factor is a divisor that completely divides the number and leaves no remainder.