An arithmetic sequence or arithmetic progression is defined as a sequence of integers where the difference between any two numbers is always constant. This difference is known as the common difference and is denoted by the letter ‘d’. We can concur that if the numbers in a list increase or decrease with a constant common difference, they are in an arithmetic sequence or arithmetic progression.
Arithmetic Progression or arithmetic sequence is also denoted by ‘AP’. You can find a lot of different examples where the abbreviation will be given. Don’t get confused and understand that it means an arithmetic progression.
Given below are some series that are arithmetic progression:
- 1, 5, 9, 13, 17, ……
- 6, 3, 1, -3, -6, …..
- a, a + 2, a + 4, a + 6, …..
So why are these APs? It can be understood by seeing the common difference between each series term.
- In the first series, (5 – 1) = 4, ( 9 – 5) = 4, (13 – 9 ) = 4, and so on. You can see that every time two terms are being subtracted, the answer comes as 4. Therefore series 1 is an arithmetic progression.
- In the second series, ( 3 – 6) = -3, (1 – 3) = -2, and ( -3 – 1) = -4. Here as you can see, the difference between two consecutive terms is changing from -3 to -2 to -4. Hence this series is not an arithmetic sequence.
- Now let us look at the algebraic sequence. (a + 2) – a = 2, (a + 4) – (a + 2) = 2, (a + 6) – (a + 4) = 2, and so on. Thus each term has a common difference of 2 between them. Hence the algebraic sequence is an arithmetic sequence.
How to Find the Common Difference between an AP
The common difference ‘d’ can be calculated by subtracting the next term from the previous term. For instance, if we have an arithmetic sequence :
AP -> a, b, c, d, e, …….
The common difference = (b – a), or (c – b), or (d – c) {The second term subtracted from the first term}. Remember that the first term ‘a’ will never be the term from which any number would be subtracted.
If the series is: 9, 6, 3, 0, …… then the common difference will never be (9 – something) it shall either be (6 – 9) = -3, or (3 – 6) = -3 in every scenario.
This leads us to a very important deduction. Suppose the common difference between any two numbers of an arithmetic series is a positive integer. In that case, the series is said to be increasing, and if the common difference is a negative integer, the series is said to be decreasing.
In mathematics, the common difference is formulated as:
d = ( t x – t x-1), where ‘t’ refers to the term and ‘x’ = 2, 3, 4, 5, ……
If you want to make an AP, then follow these steps:
Step 1: Take a starting number that will act as the first term of the AP.
Step 2: Fix a common difference and add or subtract from the first term to get the second term.
Step 3: Now, add or subtract the common difference from the second term to get the third term.
Step 4: Continue these steps to get the desired AP.
Now that we have understood the basics of arithmetic progression and the common difference let us learn the arithmetic sequence formula and how to find any number of terms in an AP.
Arithmetic Sequence Formula
From the above concept, we get the standard form of writing an arithmetic progression, which is given as:
If k is the first term of the series then AP -> k, k + d, k + 2d, k + 3d, ………, k + (n-1)d. The arithmetic sequence formula may be used to discover any term in the arithmetic sequence. Let us look at an example to understand this section.
Example: Find the 13th term of the AP: 2, 5, 8, 11, …….
Solution: Given AP = 2, 5, 8, 11, …..
Common difference ‘d’ = (5 – 2) = 3
The first term ‘k’ = 2
The 13th term of the AP = k + (n – 1) d
= 2 + (13 – 1) d
= 2 + 12 x 3
= 38
Therefore the 13th term of the given AP is 38.
From this example, we have learned that if you are provided with the first term and the common difference of an AP, you can figure out the value of any number of terms of that AP. Also, the nth term formula is given as
L = k + (n – 1)d is also known as the explicit formula for arithmetic operations.
Arithmetic Sequence Formula Applications
Every day, if not every minute, we employ the arithmetic sequence formula without even recognizing it. A few examples of real-life uses of the arithmetic sequence formula are included below.
- An arithmetic sequence is used to organize seats in a stadium or theater.
- The second hand and the minutes and hours hands in a clock move-in Arithmetic Sequence.
- The AP is followed by the weeks in a month and the years. Each leap year is calculated by adding the preceding leap year by four.
Sum of Arithmetic Sequence
Hitherto, you must be clear about what is an arithmetic sequence and the arithmetic sequence formula. We shall learn about the concept and formula related to the sum of arithmetic sequences from this article.
When we add all the terms present in the AP, it is the sum of an arithmetic sequence. This concept was founded by Carl Friedrich Gauss, who later became one of the greatest German mathematicians. He was in school in the 19th century when he found the trick to summing the number of terms of an arithmetic sequence. For example:
Find the sum of AP 1, 4, 7, and 10.
Solution: The sum of AP = 1 + 4 + 7 + 10 = 22.
This is the case when an AP contains few terms. But, what if the AP is 1, 4, 7, 10, …………100. In such cases, we cannot write the entire sequence. This is where the sum of the arithmetic sequence formula comes into action. The sum of the arithmetic formula is used to find the sum up to the rth term in any sequence. It is given as
Sr = r/2 [ 2k + (r – 1) d ]
Or
Sr = r/2 [ k + kr ]
In this formula, Sr refers to the sum of the arithmetic series till rth term, k refers to the first term of the AP, kr refers to the last term of the AP, and d is a common difference.
We can find the sum of an arithmetic sequence in two ways. If we know the first and last term of the AP, we can use the 2nd formula to find the sum directly, but if we are given the position of the rth term, then we can find the sum using the first formula. Let us solve examples related to both the formulas for a better understanding.
Example 1: Find the sum of arithmetic sequence -5, 0, 5, 10, … up to 20 terms.
Solution: Given
First term k = -5
r = 20
d = 5 – 0 = 5
Therefore using the first formula: Sr = r/2 [ 2k + (r – 1) d ]
S20 = 20/2 [ (2 x -5) + (20 – 1) x 5 ]
S20 = 10 x [ (-10) + (19) x 5 ]
S20 = 10 x [ (-10) + 95 ]
S20 = 10 x [ 85 ]
S20 = 850
Answer: The sum of the AP -5, 0, 5, 10, … up to 20 terms is 850.
Example 2: The first and last terms of an AP are 22 and 66, respectively. Find the sum of the AP up to 8 terms.
Solution: Given
First-term k = 22
kr = 66
r = 8
d = not defined
Therefore using the first formula: Sr = r/2 [ k + kr ]
S8 = 8/2 [ 22 + 66 ]
S8 = 4 x [ 88 ]
S8 = 4 x 88
S8 = 352
Answer: The sum of 8 terms of the AP is 352.
Frequently Asked Questions
1. How do you calculate arithmetic sequence?
In an arithmetic sequence, each number is added to the previous number to calculate the next number. The first term is called t1, and each subsequent term is calculated as tn+1=tn+d where d is the increment between terms.
The formula for calculating a sequence of n terms is Tn=Tn-1 + D, where D represents the difference between successive terms in the sequence.
For example, if you want to calculate a sequence that goes from 2 to 8 by 2’s (2, 4, 6, 8), you would use the formula: Tn = Tn – 1 + D = 2 + 2 = 4.
2. How to solve arithmetic sequences?
Arithmetic sequences are a type of sequence that follows a pattern of addition, much like a series of numbers on a clock. They can be solved using the formula:
a[n] = a[n-1] + d, where “a” is the first term, “n” is the number in the sequence, “d” is the difference between each term, and “a[n]” is its nth term.
Let’s say we have this sequence: 2, 4, 6, 8. It can be represented as (2+1), (4+2), or (6+3). We can see that the difference between each term is two—one more than the previous one. So if we want to find out what number comes after 10 in our sequence, we’d need to add 2 more than 9—that would be 11.
3. Where do we use arithmetic sequences in real life?
Arithmetic sequences are used in real life everywhere.
They’re used to determine how much of a product a company will sell over a period of time, or how many people will be in a classroom. They’re used to calculate how much money you’ll have if you invest $10,000 today and put it into an account that gives 10% interest each year until you withdraw it. And they’re even used to figuring out how old we’ll be when our birthday comes around next year!
4. What does arithmetic sequence mean in math?
An arithmetic sequence is a series of numbers that are added to each other to form a sequence. For example, 2, 4, 6, 8, and 10 is an arithmetic sequence because each number is the sum of the preceding two numbers.
5. What is the formula for an arithmetic sequence?
The formula for an arithmetic sequence is:
a + d = first term
d = common difference
number of terms = n
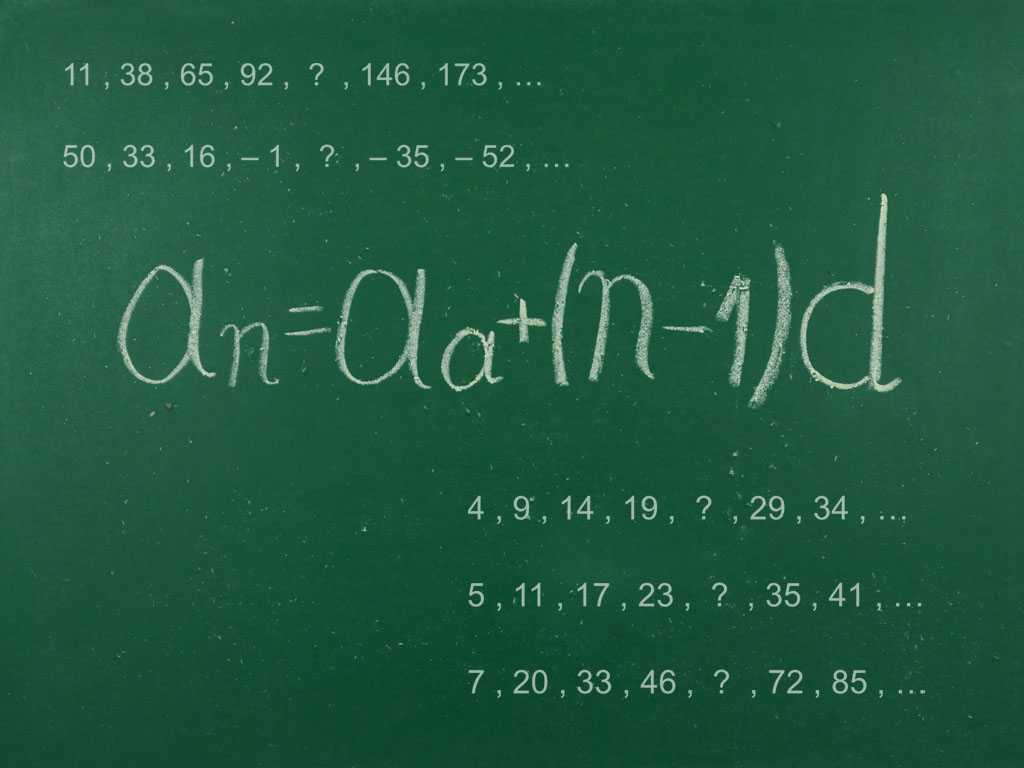
Related topics
Addition and Multiplication Using Counters & Bar-Diagrams
Introduction: We can find the solution to the word problem by solving it. Here, in this topic, we can use 3 methods to find the solution. 1. Add using counters 2. Use factors to get the product 3. Write equations to find the unknown. Addition Equation: 8+8+8 =? Multiplication equation: 3×8=? Example 1: Andrew has […]
Read More >>Dilation: Definitions, Characteristics, and Similarities
Understanding Dilation A dilation is a transformation that produces an image that is of the same shape and different sizes. Dilation that creates a larger image is called enlargement. Describing Dilation Dilation of Scale Factor 2 The following figure undergoes a dilation with a scale factor of 2 giving an image A’ (2, 4), B’ […]
Read More >>How to Write and Interpret Numerical Expressions?
Write numerical expressions What is the Meaning of Numerical Expression? A numerical expression is a combination of numbers and integers using basic operations such as addition, subtraction, multiplication, or division. The word PEMDAS stands for: P → Parentheses E → Exponents M → Multiplication D → Division A → Addition S → Subtraction Some examples […]
Read More >>System of Linear Inequalities and Equations
Introduction: Systems of Linear Inequalities: A system of linear inequalities is a set of two or more linear inequalities in the same variables. The following example illustrates this, y < x + 2…………..Inequality 1 y ≥ 2x − 1…………Inequality 2 Solution of a System of Linear Inequalities: A solution of a system of linear inequalities […]
Read More >>Other topics







Comments: