Multiplying polynomial is a basic concept in algebra. Before understanding Multiplying polynomials, one should know their background properly. To understand polynomials and how to multiply them, it is important to know what algebra is.
In mathematics, algebra is a branch that deals with variables or symbols to find unknown quantities. Apart from this, the variables present in an algebraic formula have arithmetic operations according to which an algebraic equation is solved.
What is a polynomial?
In mathematics, polynomials are identified as algebraic expressions. These expressions consist of coefficients and variables that make up the whole expression. One can perform arithmetic operations such as subtraction, multiplication, and addition to find out the value of the unknown variable in a polynomial.
A polynomial is known to be an expression that contains variables, exponents, and constants. Arithmetic operations combine these expressions to calculate the unknown variable’s value.
Depending on the Number of Expressions in an Algebraic Equation, Polynomials are Divided as:
- Monomial
A polynomial with only one non-zero term is known as a monomial. The term monomial is made up of only one term, making the operations of addition, subtraction, and multiplication simple.
A monomial consists of either a single variable or a single coefficient or product of a variable and a coefficient with whole integers as exponents, representing only one term.
Binomial and trinomial are both considered polynomials because they have two and three terms. There cannot be a variable in the numerator.
- Binomial
A binomial is an algebra equation with two different terms joined by an addition or subtraction operator. Because there are two other terms in 3xy + 8y, it is a binomial.
Different sorts of algebraic expressions can be classified based on the number of terms present, such as monomial, binomial, trinomial, and so on.
In other words, a binomial expression is an algebraic expression that consists of two unlike terms with constants and variables. Arithmetic operators like + and – are used to connect these terms. Algebraic expressions include binomials, monomials, trinomials, and more.
- Trinomial
A three-term algebraic expression is known as a trinomial. An algebraic expression is made up of one or more terms like variables and constants. As separators, these expressions use symbols or operations like +, –, and. This algebraic phrase includes a trinomial and a monomial, binomial, and polynomial.
An algebraic expression with three non-zero terms and more than one variable is known as a trinomial. A trinomial is said to be a polynomial with three terms instead of two. A polynomial is an algebraic expression with one or more terms that are expressed in standard form.
On the other hand, Trinomial can be stated using several variables and three words. x³ + y² + xy, 4x² – 4x³ + z, and xyz³ + x²z² + zy² are some instances.
Every polynomial expression is incomplete without the involvement of constants and variables. It specifies that for a polynomial equation, various expressions come together to complete the equation. The different expressions present in a polynomial term are:
- Constants – as: 1, 2, 3…
- Variables – as: a, p, x, y…
- Exponents – as 7 in a⁷ and so on.
What are the rules for multiplying polynomials?
The multiplication of polynomials includes every step that leads to the generation of the resulting equation. There are three steps to multiply polynomials. These steps are:
- Using the distributive law, multiply each term of one polynomial by the other term in the different polynomial.
- Using the exponent rule, add all the powers given in the same variables.
- Add and subtract the like terms and simplify the resulting polynomial.
It is important to note that the resulting degree of the polynomial after multiplying will always be greater than the individual degree of a polynomial.
How to perform multiplying polynomials using exponent law?
If in a polynomial equation, a variable has different exponents, then the exponent law is used to simplify the given equation.
For example,
Multiply 3x² × 2x
Here, the multiplication of birth, the coefficient, and the variable takes place separately.
= (3 × 2) × (x² × x)
= 6 × x2+1
= 6x³
Multiplying Polynomials with Exponents
The polynomials may be monomial, binomial, or trinomial after being multiplied. The procedures used to multiply any two polynomials are as follows:
Multiply the coefficients.
Exponent rules should be used to multiply the variables as needed.
Let’s look at an example to better grasp how to multiply polynomials with exponents.
Example: Multiply 10x3 with 5x2
We will follow the same procedure for multiplying polynomials with exponents as we had done above.
- Step 1: First we will multiply the coefficients i.e., 10× 5 = 50
- Step 2: Next, we will multiply the variables but, in this case, the powers of both variables will be added as per the rules of exponents i.e., x3 × x2 = x5
The final answer is 10x3 × 5x2 = 50x5
How do multiply polynomials that have different variables?
Do you know how to multiply polynomials that have different variables? Follow the steps given below.
- The first thing to do is place both polynomials in a single line.
- Now, use distributive law to separate the first polynomial from the second.
- After doing this, one can multiply the separated monomial value from the polynomial with every term given in the second polynomial.
- Now that everything has aligned, simplify the given polynomial expression if possible.
Example: Multiply 7x2 with 4y.
- Step 1: In order to begin, we must multiply the coefficients of both polynomials. i.e., 7 × 4= 28
- Step 2: The polynomials mentioned above cannot be multiplied since they have two separate variables. We shall therefore leave them unchanged.
The final answer is 7x2× 4y = 28x2y
What do you understand by multiplying polynomials by polynomials?
As mentioned above, it is known that there are different types of polynomials according to their degrees. there are monomials, binomials, trinomials, and so on.
Nevertheless, the steps for multiplying any type of polynomial remain the same. It means the above steps that explain the multiplication of a polynomial can be used to multiply various types of polynomials.
How to multiply monomial by monomial?
A monomial is a polynomial that has a single term only. It means that if two or more monomial terms multiply together, then the resulting product will also be a monomial.
For example,
To multiply this, 5x × 6x the steps are-
= 5x × 6x
= 30x²
Now, to multiply, 6x × 3y × 2z the steps will be-
= 6x × 3y × 2z
= (6 × 3 × 2) × (x × y × z)
= 36xyz
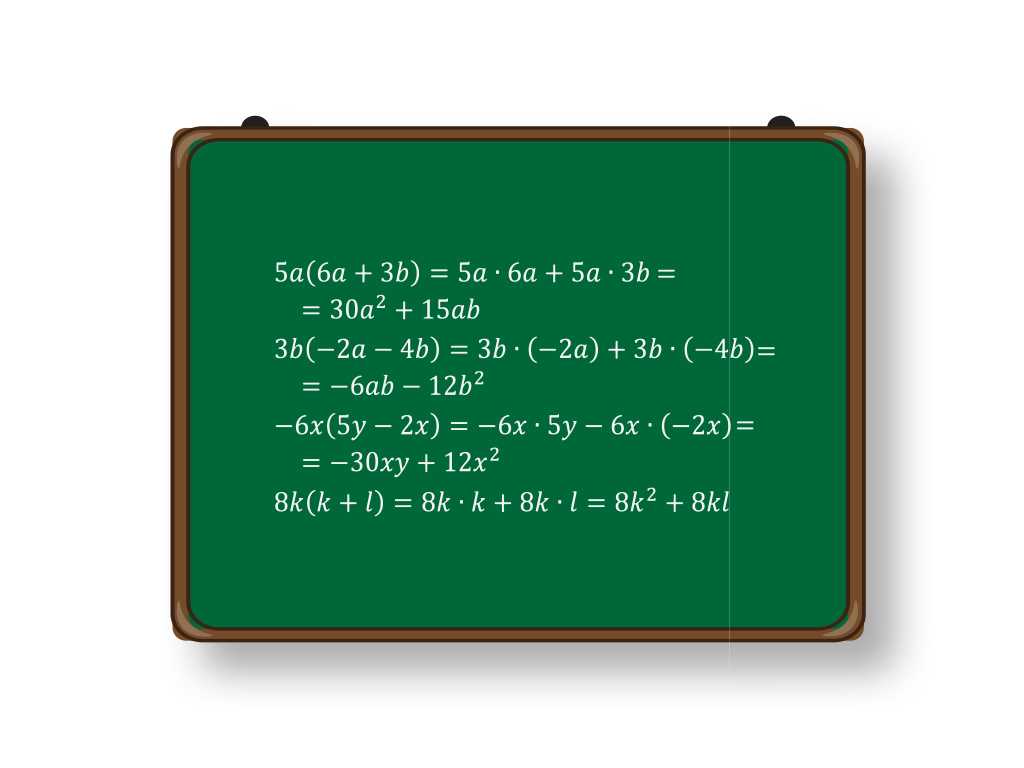
How to Solve and Multiply Binomial by Binomial?
As the name indicates, a binomial is a polynomial that contains two terms. The distributive law east followed for the multiplication of a binomial by a binomial.
As a binomial has two terms, the resulting polynomial will have four terms when multiplied.
An algebraic expression with two terms joined by a plus or minus sign is called a binomial. Binomial multiplication is analogous to multiplying two whole numbers or fractions. We’ll look at a variety of approaches to comprehending the concept of multiplying binomials.
Binomial multiplication is equivalent to the multiplication of any two-digit number. The sole distinction is that it employs the concept of algebraic expression multiplication. One binomial’s terms are multiplied by the other binomial’s terms. Following this, the algebraic total of these products is computed.
How to Multiply a Binomial with a Trinomial?
A product of a binomial and a trinomial expression is different from the polynomial multiplication problem. Even though standard methods cannot be employed since a trinomial has more than two terms, the Distributive Property is nevertheless used to organize the individual products.
According to the distributive property, each term in the binomial equation must be multiplied by each term in the trinomial.
A trinomial is a polynomial with three terms. We use the distributive law of multiplication when multiplying polynomials, such as a binomial by a trinomial. As a result, the product is projected to have 2 3 = 6 words. Let’s look at an example.
For the equation: (a² − 3a) × (a + 2b − 3c)
= a² × (a + 2b − 3c) − 3a × (a + 2b − 3c) (Distributive law of multiplication)
= (a² × a) + (a² × 2b) + (a² × −3c) − (3a × a) − (3a × 2b) − (3a × −3c) (Distributive law of multiplication)
= a³ + 2a²b − 3a²c − 3a² − 6ab + 9ac
Now, by rearranging the given terms,
(a² − 2a) × (a + 2b − 3c)
= a³ − 3a² + 3a²b − 3a²c− 6ab + 9ac
What are some Important Facts Regarding Multiplying Polynomials?
The following pointers should be kept in mind when multiplying polynomials:
- Distributive When two polynomials are multiplied, the law of multiplication is applied twice.
- Look for terms that are similar and combine them. This may minimize the product’s predicted number of phrases.
- Whenever possible, write the terms in decreasing order of exponent.
- When you open the brackets, be very careful with the signs.
Some Tips to Remember About Multiplying Polynomials
Polynomial expressions are an important segment of algebraic equations. These expressions help to simplify, calculate, and represent numerous sorts of data.
The Following are Some Useful Tips About the Multiplication of Polynomials.
- When polynomials are multiplied, the coefficient values are multiplied with a coefficient value, and the values of a variable are multiplied with a variable.
- Various methods can be used to solve the multiplication of polynomials. One can use the following methods – distributive property, the grid method, and the box method.
- One must always use exponent law to multiply a polynomial equation with exponent.
Conclusion
Multiplication of polynomials is a convenient way to simplify various equations and calculations. The above details regarding the multiplication of different types of polynomials are assistance for numerous individuals. The article covers the description of different types of polynomials and the various steps to multiply them.
Frequently Asked Questions
1. How to multiply 3 polynomials?
Ans. Divide each term in the first polynomial by its degree.
Multiply the resulting coefficients together and then add the products.
Find the sum of all terms in each binomial (the factor times itself).
Add these products together to get your answer!
2. How to multiply large polynomials?
Ans. Find the product of the first terms.
Find the product of the second term.
Add these two numbers together.
Repeat steps 1-3 until you have multiplied all of the terms together.
3. How Many Methods are there for Multiplying Polynomials?
Ans. There are three methods for multiplying polynomials.
The FOIL method
The distributive property
The FOIL method with exponents
4. What is the Best Method for Multiplying Polynomials?
Ans. The best method for multiplying polynomials is to use the FOIL method. FOIL stands for First Outer Inner Last. This method involves first multiplying the two first terms, then multiplying the outer terms, then multiplying the inner terms, and finally adding all these products together. This can be done by hand or on a calculator or computer.
5. How can we Multiply Polynomials Using the Box Method?
Ans. Multiplying polynomials is hard. To multiply polynomials using the box method, you need to multiply the coefficients of each term, then multiply the constant terms, and finally add up all your results to get your final answer.
Related topics
Addition and Multiplication Using Counters & Bar-Diagrams
Introduction: We can find the solution to the word problem by solving it. Here, in this topic, we can use 3 methods to find the solution. 1. Add using counters 2. Use factors to get the product 3. Write equations to find the unknown. Addition Equation: 8+8+8 =? Multiplication equation: 3×8=? Example 1: Andrew has […]
Read More >>Dilation: Definitions, Characteristics, and Similarities
Understanding Dilation A dilation is a transformation that produces an image that is of the same shape and different sizes. Dilation that creates a larger image is called enlargement. Describing Dilation Dilation of Scale Factor 2 The following figure undergoes a dilation with a scale factor of 2 giving an image A’ (2, 4), B’ […]
Read More >>How to Write and Interpret Numerical Expressions?
Write numerical expressions What is the Meaning of Numerical Expression? A numerical expression is a combination of numbers and integers using basic operations such as addition, subtraction, multiplication, or division. The word PEMDAS stands for: P → Parentheses E → Exponents M → Multiplication D → Division A → Addition S → Subtraction Some examples […]
Read More >>System of Linear Inequalities and Equations
Introduction: Systems of Linear Inequalities: A system of linear inequalities is a set of two or more linear inequalities in the same variables. The following example illustrates this, y < x + 2…………..Inequality 1 y ≥ 2x − 1…………Inequality 2 Solution of a System of Linear Inequalities: A solution of a system of linear inequalities […]
Read More >>Other topics







Comments: