Algebra, a branch of mathematics, deals with the symbols and arithmetic operations related to those symbols. This branch of mathematics covers a vast syllabus in itself. Suppose one needs to divide Algebra into sections. In that case, there are various sections like Polynomials, Linear equations in one variable, Linear equations in two variables, Quadratic equations, Arithmetic progressions, Rational expressions, Exponents, and many more.
Rational expressions are counted under the Elementary Algebra and are one of the important matters. It is one of the most important topics in Mathematics. Do you know about solving rational equations? No! Then, this article is exclusively only for you.
In this article, you will learn about rational equations and other terms related to them.
What Is Rational Expression?
An expression that is written in the form of numerator and denominator, i.e., in the form of p(x)q(x), where denominator q(x)0, is known as a rational expression.
Do you know?
Every polynomial is also a rational expression. If p(x) is any polynomial, then we can write p(x) = P(x)1, which is a form of rational expression.
Here are some examples of radical expressions:
3x – 45x³ + 2x+ 3, 2x² + 4x – 7x – 3x + 9, 6x+1, 4x²+6x-101, etc.
Suppose p(x) and q(x) are polynomials with integral coefficients such that the HCF of p(x) and q(x) is 1. We can say that p(x)q(x)is a rational expression in its lowest terms.
What Is Rational Equation?
A rational equation can be defined as an equation that involves at least one rational expression, or you can say a fraction. For example,
2x+14 = x3 is a rational equation.
These fractions can be on one side or both sides of the equation. It can also be a ratio of two polynomials.
A rational expression can be reduced to the lowest terms if its numerator and denominator are reduced to the lowest. For example,
p(x) = (x+3)(x-1)x(x+3) = (x-1)x
The numerator and denominator of p(x) can not be reduced anymore. Therefore, it is the lowest term of p(x).
How To Solve Rational Expressions?
Solving rational expressions can be performed by applying arithmetic operations like addition, subtraction, multiplication, division, etc.
Below are the Methods for Solving Rational Expressions Using these Operations:
1. Addition Operations:
Addition of rational expressions is defined as,
- p(x)q(x) + r(x)q(x) = p(x) + r(x)q(x)
- p(x)q(x) + r(x)s(x) = p(x)s(x) + r(x)q(x)q(x)s(x)
2. Subtraction Operations:
Subtraction of rational expressions is defined as,
- p(x)q(x) – r(x)s(x) = p(x)s(x) – r(x)q(x)q(x)s(x)
3. Multiplication Operations:
Multiplication of rational expressions is defined as,
- p(x)q(x) x r(x)s(x) = p(x).r(x)q(x).s(x)
4. Division Operations:
Division of rational expressions is defined as,
- p(x)q(x) r(x)s(x) = p(x)q(x) x s(x)r(x) = p(x).s(x)q(x).r(x)
How To Solve Rational Equations?
Solving rational equations implies getting the value of variables present in the rational equation. Now, the question arises of how to solve rational equations? Solving rational equations means clearing fractions by multiplying both sides of the equation by the least common multiple of the denominator. Remember, the denominator should not be zero.
There are many methods for solving rational equations. Solution by LCD method, Factorization method, solving by LCM method, cross-multiplying method, etc. Nowadays, solving rational equation calculators are also used to solve the given rational equations.
Solving rational equation calculators are online tools used to find the solution of the variable in the given rational equation. Suppose you want to find the solution to the rational equation using an online calculator. You need to enter the numerator and denominator in the input box and then click on the simplified option.
Steps for Solving Rational Equations by LCD Method are as Follows:
Step 1: Factorize all the denominators to find the least common denominator.
Step 2: Multiply both sides of the equation, i.e., every term of the equation with the least common denominator.
Step 3: Solve the resulting equation.
Step 4: If you get extraneous solutions, check the solutions and reject all those values resulting in the zero denominators in the original equation.
For example,
Solve 1x + 2x² = x+92x².
Solution:
Step 1: Find the LCD.
On both sides of the equation, the denominators are x, x², and 2x².
So, the LCD we get is 2x².
Step 2: Multiplying LCD with both sides of equation.
2x² (1x + 2x²) = 2x² (x+92x²)
Step 3: Solving the resultant equation.
[2x². 1x] + [2x². 2x²] = 2x² (x+92x²)
2x + 4 = x + 9
2x – x = 9 – 4
x = 5
Therefore, x = 5 is the solution for 1x + 2x² = x+92x².
Some Examples Of How To Solve Rational Equations
Here are some examples that will help you understand the method for solving rational equations.
Example 1: Solve the Equation
3(x+2)(x-4) – (x+4)(x-2) = (x-2)(x-4).
Solution:
Step 1: Find LCD.
Denominators are (x-4) and (x-2). So, the LCD in this case is (x-4)(x-2).
Step 2: Multiplying LCD with each term of the equation.
[(x – 4).(x – 2).3(x+2)(x-4)] – [(x – 4).(x – 2).(x+4)(x-2)] = [(x – 4).(x – 2).(x-2)(x-4)]
[(x – 2).3(x + 2)] – [(x – 4).(x+4)] = [(x – 2).(x – 2)]
Step 3: Solve the resulting equation.
[3(x² – 4)] – [x² – 16] = [x² + 4 – 4x]
3x² – 12 – x² + 16 = x² – 4x + 4
2x² + 4 = x² – 4x + 4
2x² – x² + 4x = 4 – 4
x² + 4x = 0
x(x + 4) = 0
x = 0, -4
Therefore, x = {0, -4} are the solutions for 3(x+2)(x-4) – (x+4)(x-2) = (x-2)(x-4).
Example 2: Solve the following equation
2(3) = 3x.
Solution:
Step 1: Find LCD.
In this case, the denominator of both sides is the same. Hence, LCD is 3.
Step 2: Multiply both sides with LCD.
3 (2(3)) = 3(3x)
Step 3: Solve the resulting equation.
2 = x
Or, x = 2
Therefore, x = 2 for the given rational equation.
How To Solve Equations With Rational Exponents?
Radical expressions, they can be written without using the radical symbol. Rational or fractional exponents are used in those cases to denote radical expressions.
For example,
nx = x1/n
Here n is called a radical symbol, while 1/n is a rational exponent. A rational exponent indicates power in the numerator and a root in the denominator.
The Steps to Solve Equations with Rational Exponents are as Follows:
Step 1: Isolate the expression with a rational exponent.
Step 2: Raise both sides of the equation to the reciprocal power.
Step 3: Solve for the value of the variable.
For example,
Solve x2/3 = 64.
Solution:
In this case, the rational exponent is already isolated. So, we move to the next step.
Step 1: Raising the power of both sides.
(x2/3)3/2 = (64)3/2
Step 2: Solving for the value of a variable.
x = (64)3/2
x = [(8²)3/2]
x = 8(2×3/2)
x = 83
x = 512
Therefore, x = 512 for x2/3 = 64.
How To Solve Equations With Rational Coefficients?
In simple words, the coefficient is defined as the number or term multiplied by the variable. In the case of the rational coefficient, the coefficient multiplied by the variable must be in the form of a fraction.
For example,
23x = 8
Here, ⅔ is the rational coefficient of x.
To solve equations with rational coefficients, you need to follow these steps:
Step 1: Isolate the expression with rational coefficient.
Step 2: Find the LCD of the equation.
Step 3: Solve the variable.
Example: Solve 710n + 32 = 35n + 3.
Solution:
Step 1: Isolate the expressions with a rational coefficient.
710n – 35n = 3 – 32
Step 2: Find the LCD.
Here, denominators are 10, 5, 1, and 2. So, the LCD is 10.
Step 3: Solve the value for the variable.
10(710n ) – 10(35n) = 10(3) – 10(32)
7n – 6n = 30 – 15
n = 15
Therefore, n = 15 for 710n + 32 = 35n + 3.
Solving Rational Equations Worksheet
Solving rational equations worksheets will help you understand the topic more precisely. To practice more on this topic, you can try the following given problems.
- If 5xx+2 = 7, then solve for x.
- Combine 2x+4 + 3x-1 into a single rational expression.
- Solve for x,
x²-5x+6x²+3x+2 = 0
- Solve the following equation:
x-37 = 4x+127
- If 3x+2 – 1x = 15x, then solve for x.
- If 3x+4 + 7x-4 = 8x²-16, then solve for x.
- Simplify 9x = 18.
Frequently Asked Questions
1. How do you simplify a rational expression?
Ans. To simplify a rational expression, you divide out all the common factors.
For example, if you have the expression 2x+3, you can divide out 2 to get x+3.
If you have the expression 6x-7+9x-9, you can divide out 6 and 9 to get 3x-2.
2. What is the first step to simplifying a rational expression?
Ans. The first step to simplifying a rational expression is to write out the numerator and denominator as a pair of fractions. This makes it easier to see where you can combine like terms and then simplify by reducing fractions.
3. What is a simple rational expression?
Ans. A simple rational expression is a rational expression where there are no fractions, only integers, and variables. For example, the expression 2x/3 is a simple rational expression because it contains only integers (2 and 3) and variables (x).
4. What is a rational expression example?
Ans. A rational expression is an algebraic expression that can be written in the form p/q, where p and q are polynomials. For example, 5/2, 3×2+4y-5, and 2x+5 are all rational expressions.
5. How do you simplify expressions with rational expressions?
Ans. When you have a rational expression, it’s important to simplify it first do this, you take all the fractions and multiply them by their denominators so that they’re fractions with no denominators. For example, if the original equation was “x/6 + x/7 = 2x/14”, then after simplifying it would become “6x + 7x = 14x”.You then get rid of any like terms by adding or subtracting them together until they’re gone. So you’d get 6x + 7x = 14x – 6x = 8x.
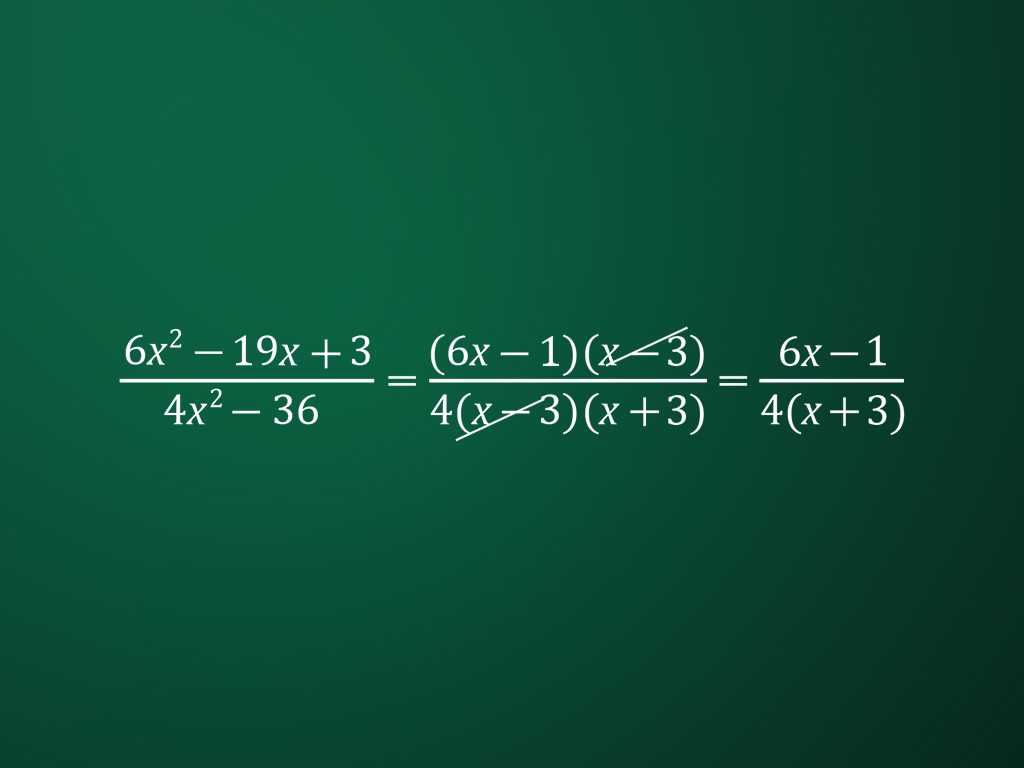
Related topics
Addition and Multiplication Using Counters & Bar-Diagrams
Introduction: We can find the solution to the word problem by solving it. Here, in this topic, we can use 3 methods to find the solution. 1. Add using counters 2. Use factors to get the product 3. Write equations to find the unknown. Addition Equation: 8+8+8 =? Multiplication equation: 3×8=? Example 1: Andrew has […]
Read More >>Dilation: Definitions, Characteristics, and Similarities
Understanding Dilation A dilation is a transformation that produces an image that is of the same shape and different sizes. Dilation that creates a larger image is called enlargement. Describing Dilation Dilation of Scale Factor 2 The following figure undergoes a dilation with a scale factor of 2 giving an image A’ (2, 4), B’ […]
Read More >>How to Write and Interpret Numerical Expressions?
Write numerical expressions What is the Meaning of Numerical Expression? A numerical expression is a combination of numbers and integers using basic operations such as addition, subtraction, multiplication, or division. The word PEMDAS stands for: P → Parentheses E → Exponents M → Multiplication D → Division A → Addition S → Subtraction Some examples […]
Read More >>System of Linear Inequalities and Equations
Introduction: Systems of Linear Inequalities: A system of linear inequalities is a set of two or more linear inequalities in the same variables. The following example illustrates this, y < x + 2…………..Inequality 1 y ≥ 2x − 1…………Inequality 2 Solution of a System of Linear Inequalities: A solution of a system of linear inequalities […]
Read More >>Other topics







Comments: