Ordered Pair: Definition, Examples, & Properties 

Overview

In mathematics, the ordered pair is among the basic tools. It’s one of the first concepts taught in middle school algebra classrooms, and it’s most typically used to find a point on the graphs.

Let’s find out more about ordered pairs, including their definition, meanings, properties, and more. 

What is an Ordered Pair?

Ordered pairs in mathematics are defined as a set of 2 numbers that exist in a particular order. The order followed by the pair is fixed. It is a very important concept of the Cartesian plane and set theory. Geometry has a lot of applications of ordered pairs. In this article, we shall dive into the world of ordered pairs and learn what is an ordered pair with a few examples. 

Ordered pairs are always written inside brackets. The parentheses are the most common brackets used to represent ordered pairs. They are generally written as:

(Value 1, Value 2)

In most cases, the value 1 is the x coordinate, and value 2 is the y coordinate of the cartesian plane. As you can see the values are enclosed between the parentheses separated by a comma. You can write this as (x, y) or (a, b). The values depend on the point of the graph.

In geometry, the ordered pairs represent different points on the graph. For example: (0,0) is the ordered pair of the origin. The magnitude of the values tells us the position of the point on the cartesian plane. For instance (-1, -4) lies in the fourth quadrant of the cartesian plane. These are thus helpful in identifying the points on a graph, finding the distance between 2 points, interpreting the type of graph, finding the equation of the line, etc.

Did you know that the Cartesian plane was constructed by Rene Descartes and Pierre de Fermat, two mathematicians who invented analytic geometry in the early 16th century? 

Definition of Ordered Pair

As discussed above the ordered pairs are represented as (a,b). In every ordered pair:

‘a’ is known as the abscissa and is defined as the distance measure of a point from the horizontal axis (x-axis).

‘b’ is called the ordinate. It is defined as the distance measured between the y-axis and any point. 

It can be represented through a table with more differences. 

‘A’ Element of Ordered Pair	‘B’ Element of Ordered Pair
It is x- coordinate	It is y-coordinate
It is also known as an “abscissa.”	It is also known as an “ordinate.”
It indicates the point’s horizontal distance from the origin.	It indicates the point’s vertical distance from the origin.
It appears on the X-axis	It appears on the Y- axis
It displays how far away the point is from the Y-axis.	It displays how far away the point is from the X-axis.
For example,  if a coordinate plane point is at (2, 4), its distance from the y-axis is 2.	For example, if a coordinate plane point is at (2, 4), its distance from the y-axis is 4.

To mark a point on the graph, you must place a dot at the ordered pair’s coordinates. The x-coordinate indicates how many steps are required to reach the x-axis. The y-coordinate indicates that we will be moving along the y-axis in a large number of steps.

The ordered pair, on the other hand, has many more applications and is crucial in the foundation of set theory in many branches of mathematics. This mathematical structure is fundamentally similar to a set of two components but with one essential difference: order. The ordered pair (a,b) is not equal to (b, a) unlike the set {a,b} = {b, a}.

Set of Ordered Pairs

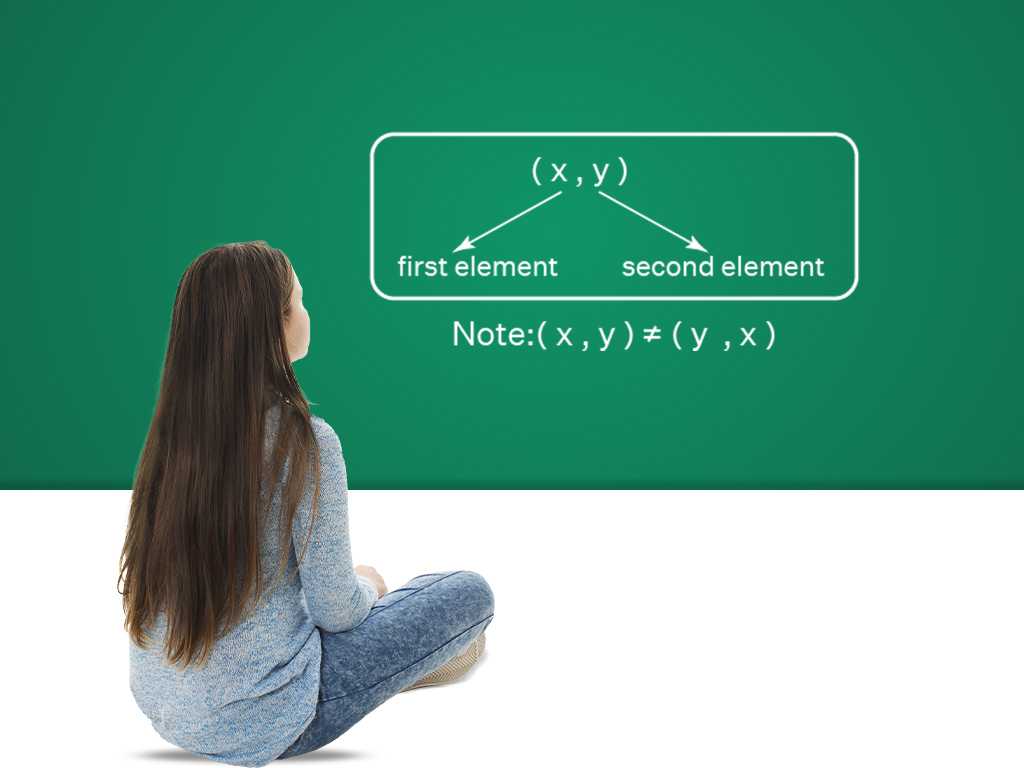
A set of ordered pairs is a pair of components that appear in a specific order and are wrapped in brackets. If ‘x’ and ‘y’ are two components, then (x, y) and (y, x) are the two distinct ordered pairs. The x element in an ordered pair (a, b) is referred to as the first element, while the y element is referred to as the second element.

If X and Y are two sets with the properties x ∈ X and y ∈ Y, then the ordered pair of elements is (a, b), with ‘a’ being the first element and ‘b’ being the second member in the ordered pair.

When we reverse the arrangement of the elements in an ordered pair, the ordered pair changes as well, becoming (y, x) rather than (x, y) ≠ (y, x).

2-tuples and sequence of length 2 are other names for ordered pairs. The ordered pairs can also have the same value for both elements. This is represented as B = (b,b). For example, (11, 11) or (5, 5).  

Ordered Pair Examples 

The ordered pair (7, 3) is a pair of two integers that are carefully in order, with 7 as the abscissa and 3 as the ordinate. If you interchange the places of this ordered pair, we get (3, 7). We can see that (7, 3) is not at all equal to (3, 7). Thus remember that the order of the pieces in a pair is crucial.

Example: If (a, b) = (2, -7) determine the value of 5a – 6b + 12?

Solution: We are given that ordered pair (a, b) = (2, -7). 

For such types of questions, we have to directly substitute the value of the parameters. In this case a = 2 and b = -7. Substituting the value of ‘a’ and ‘b’ we get:

5 x (2) – 6 x (-7) + 12

→ 10 – (-42) + 12

→ 10 + 42 + 12

→ 64

Let us try to define an ordered triplet, or 3-tuple, before moving on. We might define it by expanding the description above to include three sets, with the third comprising x, y, and z, but as we increase the definition to n-tuples, this becomes too complicated. Instead, we’ll use the following definition for the 3-tuple:

(x, y, z) = ((x,y),z) or (x, (y,z))

We can infer a 3-tuple is merely an ordered pair with another pair as the first element. It’s simple to find how we may broaden the definition of a 3-tuple to include an n-tuple. Simply said, the last tuple should be the 1st element of an ordered pair, and then continue, each time adding a new element.

Properties of Ordered Pairs

Equality of Ordered Pairs

Two ordered pairs can be equal. This is a unique property of ordered pairs. Let us consider two ordered pairs (x, y) and (u, v). These ordered pairs will be equal if their corresponding initial elements and second elements are equal to each other. 

This can be understood by the above-mentioned pairs ordered. They will be equal if x = u and y = v. (x, y) = (u, v). Let us see a problem in comprehending the use of the equality of ordered pairs concept.

Question 1: Find the values of a and b when (3a – 11, b + 4) = (2a + 9, 21)

Solution: We are given that 3a – 11 = 2a + 9 and b + 4 = 21

This was possible due to the equality of ordered pairs of property

LHS

⇒ 3a – 11 = 2a + 9

⇒ 3a – 2a = 9 + 11

⇒ a = 20. 

RHS 

⇒ b + 4 = 21

⇒ b = 17

Hence a = 20 and b = 17.

How to Plot Ordered Pairs on a Graph

Plotting the ordered pairs on a graph is basic mathematics. It is as easy as applying butter to our pancakes. Once you know the trick you can easily plot any number on the Cartesian plot. The steps to accurately place ordered pairs on a graph are:

• Write the point as an ordered pair (if not already given in bracket form).
• The first element of the ordered pair tells us how many steps to move on the x-axis of the graph. Move to the required number and draw a dotted vertical line on the graph.
• Similarly, the second element of the ordered pair defines the steps to move on the y-axis of the graph. Reach the required integer on the y-axis and make another dotted line but horizontally on the graph.
• Look for the point where both the dotted lines intersect with each other. That point is the required ordered pair. 
• If a negative sign appears on the value of abscissa or ordinate, then move opposite in the direction of the line. 

Example: Plot the point ‘A’ on a graph with coordinates as x = 5 and y = 8.

Solution: We shall follow the steps mentioned above and plot point A.

Step 1: According to the first step we shall write point A as an ordered pair hence A = (5, 8)

Step 2: The position from the “x” is shown by the first element in the ordered pair “axis, which is number five. Find number 5 on the x-axis and make a dotted line (vertical).

Step 3: The position from the “y” is shown by the second element in the ordered pair “axis, which is number eight. Find number 8 on the y-axis and draw a horizontal dotted line. 

or 

Begin at the origin to find the point on the graph. Starting at the origin, take 6 steps towards the “x” axis (to the right). Move four steps towards the “y” axis from here (upwards).

Step 4: Mark point A at the intersection of the dotted line. This is the ordered pair you needed to plot on the graph. 

Ordered Pair Application

• In computer science, ordered pairs are commonly employed for programming and deploying parameters in the functions.
• It is used to represent the center and vertices of geometric figures in statistics.
• Data understanding and visual understanding are both aided by ordered pairs.

Frequently Asked Questions

1. What Is an Ordered Pair in Math?

Ans. An ordered pair is a combination of two values that are paired together to represent a specific location in the plane. The first value represents the x value or horizontal distance from the origin (the point where all lines meet). The second value represents the y value or vertical distance from the origin.

2. What Is the Difference Between Ordered and Unordered Pairs?

Ans. An ordered pair is a set of two numbers that are separated by an “=” sign. An unordered pair is a set of two numbers that are separated by a comma. It’s not necessary to write them in order, so they’re “unordered.”

3. What Is the Ordered Pair of 2 5?

Ans. An ordered pair of 2 5 is a pair of numbers that can be written in the form (x, y) where x and y are real numbers. The first number in an ordered pair is called the x-coordinate, and the second number is called the y-coordinate.

4. How Do You Know If an Ordered Pair Is Not Valid?

Ans. An ordered pair is not valid if it does not contain a real number. In other words, if either of the coordinates in an ordered pair is negative or greater than or equal to one, the ordered pair is invalid.

5. Which Value Is Listed First in an Ordered Pair?

Ans. If you’re trying to find out which value is listed first in an ordered pair, you’ve come to the right place! Here’s the trick: if you have two numbers, x, and y, then x is always listed as the first value in an ordered pair. So if you see (3, 7), then 3 is the first number and 7 is the second number.